题目内容
2.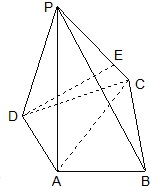 如图,在四棱锥P-ABCD中,PA⊥底面ABCD,AC⊥AB,AD⊥DC,∠DAC=60°,PA=AC=2,AB=1,点E在棱PC上,且DE⊥PB.
如图,在四棱锥P-ABCD中,PA⊥底面ABCD,AC⊥AB,AD⊥DC,∠DAC=60°,PA=AC=2,AB=1,点E在棱PC上,且DE⊥PB.(Ⅰ) 求CE的长;
(Ⅱ) 求二面角A-PB-C的正弦值.
分析 (I)建立空间直角坐标系,设出点的坐标得出$\overrightarrow{DE}=(\frac{{\sqrt{3}}}{2},\frac{3}{2}-h,h),\overrightarrow{PB}=(1,0,-2)$.
根据DE⊥PB,运用数量积求解即可.
(II)平面PBC的法向量为$\overrightarrow n=(x,y,z)$,根据$\overrightarrow{PC}=(0,2,-2),\overrightarrow{PB}=(1,0,-2)$,
易知$\overrightarrow{AC}=(0,2,0)$是平面PAB的法向量,运用$cos\left?{\overrightarrow n,\overrightarrow{AC}}\right>=\frac{{\overrightarrow n•\overrightarrow{AC}}}{{|{\overrightarrow n}|•|{\overrightarrow{AC}}|}}=\frac{{\sqrt{6}}}{6}$,求解正弦即可.
解答 解:(Ⅰ) 如图,以$\overrightarrow{AB},\overrightarrow{AC},\overrightarrow{AP}$分别为x,y,z轴的正半轴方向,建立空间直角坐标系,则P(0,0,2),A(0,0,0),B(1,0,0),$C(0,2,0),D(-\frac{{\sqrt{3}}}{2},\frac{1}{2},0)$.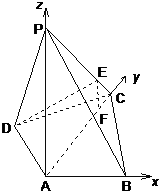
过E作EF⊥AC于F,由已知,得EF∥PA,
设EF=h,则E(0,2-h,h).
∴$\overrightarrow{DE}=(\frac{{\sqrt{3}}}{2},\frac{3}{2}-h,h),\overrightarrow{PB}=(1,0,-2)$.
∵DE⊥PB,∴$\overrightarrow{DE}•\overrightarrow{PB}=\frac{{\sqrt{3}}}{2}-2h=0$,$h=\frac{{\sqrt{3}}}{4}$,
∴$CE=\sqrt{2}h=\frac{{\sqrt{6}}}{4}$.
(Ⅱ)由(Ⅰ)得$\overrightarrow{PC}=(0,2,-2),\overrightarrow{PB}=(1,0,-2)$,
设平面PBC的法向量为$\overrightarrow n=(x,y,z)$,
则 $\left\{\begin{array}{l}\overrightarrow n•\overrightarrow{PC}=0\\ \overrightarrow n•\overrightarrow{PB}=0\end{array}\right.$.
∴$\left\{\begin{array}{l}2y-2z=0\\ x-2z=0\end{array}\right.$,取z=1,得$\overrightarrow n=(2,1,1)$.
易知$\overrightarrow{AC}=(0,2,0)$是平面PAB的法向量,
∴$cos\left?{\overrightarrow n,\overrightarrow{AC}}\right>=\frac{{\overrightarrow n•\overrightarrow{AC}}}{{|{\overrightarrow n}|•|{\overrightarrow{AC}}|}}=\frac{{\sqrt{6}}}{6}$,
则二面角A-PB-C的正弦值为sin<$\overrightarrow{n}$,$\overrightarrow{AC}$>=$\frac{\sqrt{30}}{6}$.
点评 本题考查了空间向量的在求解空间角中的应用,考查了学生的空间思维能力,计算能力,属于中档题.

 阅读快车系列答案
阅读快车系列答案
| A. | 870 | B. | 30 | C. | 6 | D. | 3 |
| A. | min{|a+b|,|a-b|}≤min{|a|,|b|} | B. | max{|a+b|,|a-b|}≤max{|a|,|b|} | ||
| C. | min{|a+b|2,|a-b|2}≥|a|2+|b|2 | D. | max{|a+b|2,|a-b|2}≥{|a|2+|b|2 |
| A. | $-\frac{1}{2}$ | B. | $\frac{1}{2}$ | C. | -2 | D. | 2 |
| A. | 9 | B. | 14 | C. | 15 | D. | 16 |