题目内容
给出集合A={-2,-1,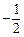 ,
,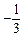 ,
,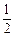 ,1,2,3}
,1,2,3} 。已知a∈A,使得幂函数
。已知a∈A,使得幂函数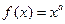 为奇函数,指数函数
为奇函数,指数函数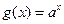 在区间(0,+∞)上为增函数。
在区间(0,+∞)上为增函数。
(1)试写出所有符合条件的a,说 明理由;
明理由;
(2)判断f(x)在(0,+∞)的单调性,并证明;
(3)解方程:f[g(x)]=g[f (x)]。
(x)]。
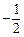 ,
,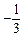 ,
, ,1,2,3}
,1,2,3} 。已知a∈A,使得幂函数
。已知a∈A,使得幂函数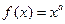 为奇函数,指数函数
为奇函数,指数函数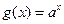 在区间(0,+∞)上为增函数。
在区间(0,+∞)上为增函数。(1)试写出所有符合条件的a,说
 明理由;
明理由;(2)判断f(x)在(0,+∞)的单调性,并证明;
(3)解方程:f[g(x)]=g[f
 (x)]。
(x)]。(1)a=3
(2)f(x)=x3在(0,+∞)上为增函数
(3)x1=0,x2=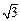 ,x3=
,x3=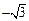
(2)f(x)=x3在(0,+∞)上为增函数
(3)x1=0,x2=
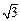 ,x3=
,x3=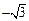
解:(1)指数函数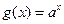 在区间(0,+∞)上为增函数,∴a>1,∴a只可能为2或3。而当a=2时,幂函数f(x)=x2为偶函数,只有当a=3时,幂函数f(x)=x3为奇函数
在区间(0,+∞)上为增函数,∴a>1,∴a只可能为2或3。而当a=2时,幂函数f(x)=x2为偶函数,只有当a=3时,幂函数f(x)=x3为奇函数 故a=3…3分
故a=3…3分
(2)f(x)=x3在(0,+∞)上为增函数。
证明:在(0,+∞)上任取x1,x2,x1<x2,
f(x1)-f(x2)=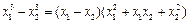 =
=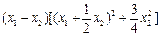 ,
,
∵x1< x2,∴x1-x2<0,
x2,∴x1-x2<0,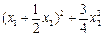 >0,∴f(x1)-f(x2)>0,∴f(x1)>f(x2)。
>0,∴f(x1)-f(x2)>0,∴f(x1)>f(x2)。
∴f(x)=x3在(0,+∞)上为增函数。 …8分
(3)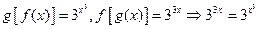 ....10分
....10分
根据指数函数的性质,得3x=x3,∴x1=0,x2=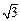 ,x3=
,x3=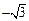 。 …12分
。 …12分
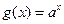 在区间(0,+∞)上为增函数,∴a>1,∴a只可能为2或3。而当a=2时,幂函数f(x)=x2为偶函数,只有当a=3时,幂函数f(x)=x3为奇函数
在区间(0,+∞)上为增函数,∴a>1,∴a只可能为2或3。而当a=2时,幂函数f(x)=x2为偶函数,只有当a=3时,幂函数f(x)=x3为奇函数 故a=3…3分
故a=3…3分(2)f(x)=x3在(0,+∞)上为增函数。
证明:在(0,+∞)上任取x1,x2,x1<x2,
f(x1)-f(x2)=
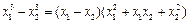 =
=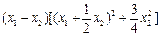 ,
,∵x1<
 x2,∴x1-x2<0,
x2,∴x1-x2<0,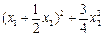 >0,∴f(x1)-f(x2)>0,∴f(x1)>f(x2)。
>0,∴f(x1)-f(x2)>0,∴f(x1)>f(x2)。∴f(x)=x3在(0,+∞)上为增函数。 …8分
(3)
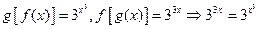 ....10分
....10分根据指数函数的性质,得3x=x3,∴x1=0,x2=
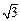 ,x3=
,x3=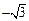 。 …12分
。 …12分
练习册系列答案
相关题目
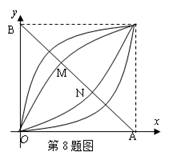
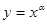 ,当
,当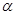 取不同的正数时,在区间
取不同的正数时,在区间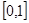 上它们的图像是一族美丽的曲线(如图).设点
上它们的图像是一族美丽的曲线(如图).设点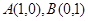 ,连接AB,线段AB恰好被其中的两个幂函数
,连接AB,线段AB恰好被其中的两个幂函数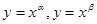 的图像三等分,即有
的图像三等分,即有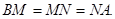 那么,ab=( )
那么,ab=( )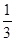
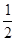
 (n=2k,k∈Z)的图象在[0,+∞)上单调递增,解不等式f(x2-x)>f(x+3).
(n=2k,k∈Z)的图象在[0,+∞)上单调递增,解不等式f(x2-x)>f(x+3).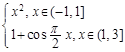 ,则函数
,则函数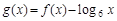 的零点个数是( )
的零点个数是( )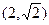 在幂函数
在幂函数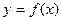 的图象上,则
的图象上,则 .
. 在定义域上递增。
在定义域上递增。 的解析式;
的解析式; ,在区间上的最大值为5。若存在,求出m的值;若不存在,请说明理由。
,在区间上的最大值为5。若存在,求出m的值;若不存在,请说明理由。 时,
时,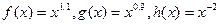 的大小关系是______________.
的大小关系是______________.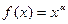 的图像经过点
的图像经过点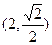 ,则
,则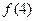 的值为( )
的值为( )
