题目内容
(本小题满分14分)设椭圆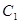 与抛物线
与抛物线 的焦点均在
的焦点均在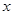 轴上,
轴上,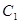 的中心和
的中心和 的顶点均为原点,从每条曲线上至少取两个点,将其坐标记录于下表中:
的顶点均为原点,从每条曲线上至少取两个点,将其坐标记录于下表中:
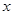 |  |  |  |  |  |
 |  |  |  |  |  |
1)求
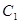 ,
, 的标准方程, 并分别求出它们的离心率
的标准方程, 并分别求出它们的离心率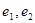 ;
;2)设直线
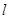 与椭圆
与椭圆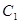 交于不同的两点
交于不同的两点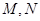 ,且
,且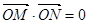 (其中
(其中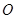 坐标原点),请问是否存在这样的直线
坐标原点),请问是否存在这样的直线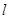 过抛物线
过抛物线 的焦点
的焦点 若存在,求出直线
若存在,求出直线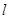 的方程;若不存在,请说明理由.
的方程;若不存在,请说明理由.
(1)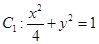 ,
,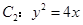 ,
,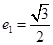 ,
,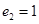 。(2)
。(2)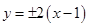 。
。
解析试题分析:(1)∵焦点在x轴上,且椭圆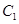 与抛物线
与抛物线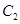 的中心与顶点在原点,又过点
的中心与顶点在原点,又过点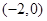 ,
,
故点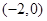 在椭圆上,点
在椭圆上,点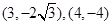 在抛物线
在抛物线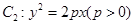 上
上 ,
,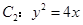
∴点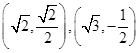 在
在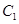 上,
上,
设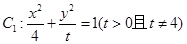
把点 代入得
代入得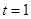 ,
,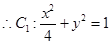
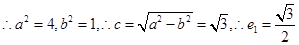
由抛物线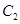 知
知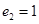
(2)由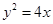 得
得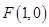
若l与x轴垂直,则l:x=1
由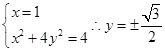
设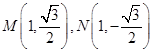 不满足
不满足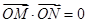
若存在直线l不与x轴垂直,可设为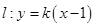
设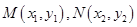
由
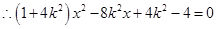

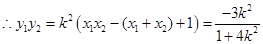


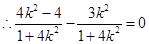

所求的直线为
考点:椭圆与抛物线的标准方程及简单性质;直线与椭圆的综合应用。
点评:(1)做第一问的关键是确定哪两个点在椭圆上,哪两个点在抛物线上。(2)在求直线与圆锥曲线相交的有关问题时,通常采用设而不求的方法,在求解过程中一般采取步骤为:设点→联立方程→消元→韦达定理。

练习册系列答案
相关题目
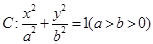 的焦点为
的焦点为 、
、 ,离心率为
,离心率为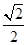 ,过点
,过点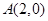 的直线
的直线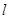 交椭圆
交椭圆 于
于 、
、 两点.
两点.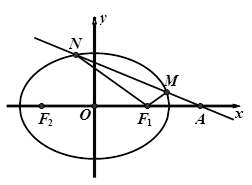
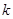 的取值范围;
的取值范围; 和
和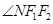 是否总相等?若相等,请给出证明,若不相等,说明理由.
是否总相等?若相等,请给出证明,若不相等,说明理由.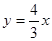 ,右焦点
,右焦点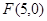 ,双曲线的实轴为
,双曲线的实轴为 ,
,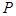 为双曲线上一点(不同于
为双曲线上一点(不同于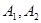 ),直线
),直线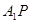 ,
,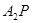 分别与直线
分别与直线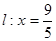 交于
交于 两点
两点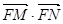 是否为定值,若为定值,求出该值;若不为定值,说明理由。
是否为定值,若为定值,求出该值;若不为定值,说明理由。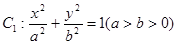 经过点
经过点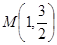 ,且其右焦点与抛物线
,且其右焦点与抛物线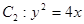 的焦点F重合.
的焦点F重合.  的方程;
的方程; 经过点
经过点 与椭圆
与椭圆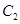 相交于C、D两点.求
相交于C、D两点.求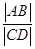 的最大值.
的最大值. ,焦点在x轴上,离心率为
,焦点在x轴上,离心率为 ,又椭圆上任一点到两焦点的距离和为
,又椭圆上任一点到两焦点的距离和为 ,过点M(0,
,过点M(0, )与x轴不垂直的直线
)与x轴不垂直的直线 交椭圆于P、Q两点.
交椭圆于P、Q两点.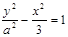 的焦点为F1,F2.离心率为2。
的焦点为F1,F2.离心率为2。 ,求线段AB中点M的轨迹方程,并说明轨迹是什么曲线。
,求线段AB中点M的轨迹方程,并说明轨迹是什么曲线。 过点
过点 ,且离心率为
,且离心率为 .
. 的方程;
的方程; 为椭圆
为椭圆 是椭圆
是椭圆 分别交直线
分别交直线 于
于 两点.
两点.  为直径的圆恒过
为直径的圆恒过 轴上的定点.
轴上的定点. 分别是椭圆的
分别是椭圆的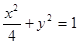 左,右焦点。
左,右焦点。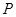 是第一象限内该椭圆上的一点,且
是第一象限内该椭圆上的一点,且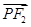 ·
·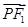 =
=
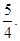 求点
求点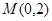 的直线与椭圆交于不同的两点
的直线与椭圆交于不同的两点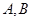 ,且
,且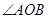 为锐角(其中O为坐标原点),求直线
为锐角(其中O为坐标原点),求直线 的斜率
的斜率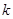 的取值范围。
的取值范围。 中,点
中,点 到两点
到两点 的距离之和为4,设点
的距离之和为4,设点 ,直线
,直线 与
与 两点。
两点。 ,求
,求 的值。
的值。