题目内容
设曲线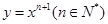 在点(1,1)处的切线与x轴的交点的横坐标为
在点(1,1)处的切线与x轴的交点的横坐标为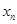 ,令
,令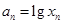 ,则
,则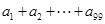 的值为
的值为
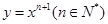 在点(1,1)处的切线与x轴的交点的横坐标为
在点(1,1)处的切线与x轴的交点的横坐标为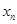 ,令
,令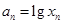 ,则
,则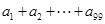 的值为
的值为 -2 ;
因为对y=xn+1(n∈N*)求导得y′=(n+1)xn,
令x=1得在点(1,1)处的切线的斜率k=n+1,
在点(1,1)处的切线方程为y-1=k(xn-1)=(n+1)(xn-1),
不妨设y=0,xn=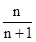 ,则所求的为-2.
,则所求的为-2.
令x=1得在点(1,1)处的切线的斜率k=n+1,
在点(1,1)处的切线方程为y-1=k(xn-1)=(n+1)(xn-1),
不妨设y=0,xn=
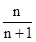 ,则所求的为-2.
,则所求的为-2.
练习册系列答案
 名校课堂系列答案
名校课堂系列答案
相关题目
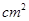 )是关于截去的小正方形的边长x(单位:
)是关于截去的小正方形的边长x(单位: )的函数。⑴ 随着x的变化,容积V是如何变化的?
)的函数。⑴ 随着x的变化,容积V是如何变化的?













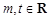 ,函数
,函数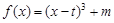 .
.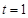 时,若
时,若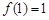 ,求函数
,求函数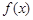 的单调区间;
的单调区间;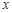 的不等式
的不等式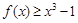 在区间
在区间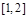 上有解,求
上有解,求 的取值范围;
的取值范围; ,
, =
= 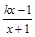 (
(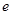 是自然对数的底)
是自然对数的底)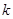 的取值范围;
的取值范围; >0,都有
>0,都有 ,求满足条件的最大整数
,求满足条件的最大整数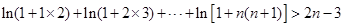 ,
, .
.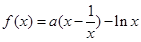
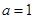 时,求曲线
时,求曲线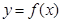 在点
在点 处的切线方程;
处的切线方程;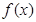 在其定义域内为增函数,求实数
在其定义域内为增函数,求实数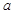 的取值范围;
的取值范围;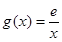 ,若在
,若在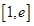 上至少存在一点
上至少存在一点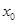 使
使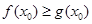 成立,求实数
成立,求实数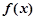 是定义在
是定义在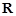 上的奇函数,且对任意
上的奇函数,且对任意 都有
都有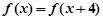 ,当
,当 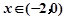 时,
时,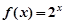 ,则
,则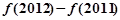 的值为( )
的值为( )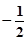
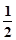
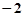
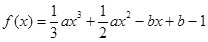 在
在 处的切线与
处的切线与 轴平行,若
轴平行,若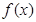 的图象经过四个象限,则实数
的图象经过四个象限,则实数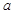 的取值范围是 。
的取值范围是 。 的
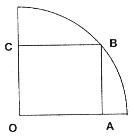
的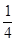 圆形(O为圆心)铝皮上截取一块矩形材料OABC,其中点B在圆弧上,点A、C在两半径上,现将此矩形铝皮OABC卷成一个以AB为母线的圆柱形罐子的侧面(不计剪裁和拼接损耗),设矩形的边长
圆形(O为圆心)铝皮上截取一块矩形材料OABC,其中点B在圆弧上,点A、C在两半径上,现将此矩形铝皮OABC卷成一个以AB为母线的圆柱形罐子的侧面(不计剪裁和拼接损耗),设矩形的边长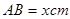 ,圆柱的体积为
,圆柱的体积为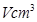 .
.
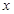 的函数关系式;
的函数关系式;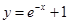 在
在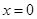 处的切线方程为_____________.
处的切线方程为_____________.