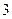题目内容
(本小题满分12分)已知定义域为R的函数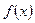 为奇函数,且满足
为奇函数,且满足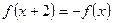 ,当x∈[0,1]时,
,当x∈[0,1]时,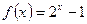 .
.
(1)求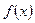 在[-1,0)上的解析式;
在[-1,0)上的解析式;
(2)求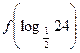 .
.
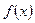 为奇函数,且满足
为奇函数,且满足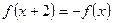 ,当x∈[0,1]时,
,当x∈[0,1]时,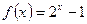 .
.(1)求
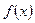 在[-1,0)上的解析式;
在[-1,0)上的解析式;(2)求
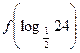 .
.(1)
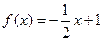
(2)
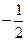
解 (1)令x∈[-1,0),则-x∈(0,1],∴f(-x)=2-x-1.
又∵f(x)是奇函数,∴f(-x)=-f(x),∴-f(x)=f(-x)=2-x-1,
∴f(x)=-(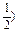 x+1.
x+1.
(2)∵f(x+2)=-f(x),∴f(x+4)=-f(x+2)=f(x),∴f(x)是以4为周期的周期函数,
∵log 24=-log224∈(-5,-4),∴log
24=-log224∈(-5,-4),∴log 24+4∈(-1,0),
24+4∈(-1,0),
∴f(log 24)=f(log
24)=f(log 24+4)=-(
24+4)=-(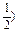
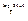 +1=-24×
+1=-24×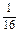 +1=-
+1=-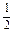 .
.
又∵f(x)是奇函数,∴f(-x)=-f(x),∴-f(x)=f(-x)=2-x-1,
∴f(x)=-(
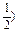 x+1.
x+1.(2)∵f(x+2)=-f(x),∴f(x+4)=-f(x+2)=f(x),∴f(x)是以4为周期的周期函数,
∵log
 24=-log224∈(-5,-4),∴log
24=-log224∈(-5,-4),∴log 24+4∈(-1,0),
24+4∈(-1,0),∴f(log
 24)=f(log
24)=f(log 24+4)=-(
24+4)=-(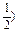
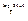 +1=-24×
+1=-24×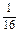 +1=-
+1=-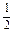 .
.
练习册系列答案
 名校课堂系列答案
名校课堂系列答案
相关题目
 在定义域R上不是常数函数,且
在定义域R上不是常数函数,且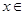 R,
R, ,则
,则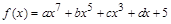 ,其中
,其中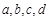 为常数,若
为常数,若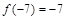 ,则
,则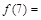 _____
_____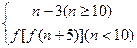 ,其中n∈N,则f(8)等于
,其中n∈N,则f(8)等于 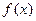 为奇函数,当
为奇函数,当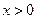 时,
时,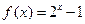 ,则
,则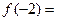
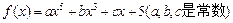 ,且
,且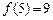 ,则
,则 的值为 ▲ .
的值为 ▲ .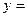
 是定义在R上的奇函数,且当
是定义在R上的奇函数,且当 时不等
时不等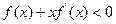 成立,
成立, 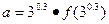 ,
,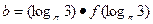 ,
,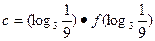 则
则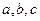 的大小关系是 ( )
的大小关系是 ( )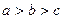 B
B 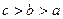 C
C 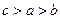 D
D 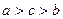
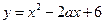 是偶函数,则
是偶函数,则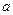 的值是 .
的值是 .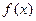 为偶函数,且
为偶函数,且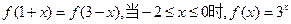 ,若
,若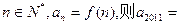 ( )
( )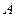 .
.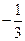
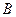 .
.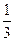
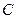 .
.
 .
.