题目内容
(本小题满分12分)
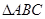 中,角
中,角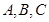 的对边分别为
的对边分别为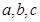 ,且
,且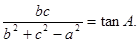
(1) 求角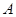 ;
;
(2) 设函数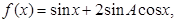 将函数
将函数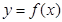 的图象上各点的纵坐标保持不变,横坐标缩短到原来的
的图象上各点的纵坐标保持不变,横坐标缩短到原来的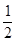 ,把所得图象向右平移
,把所得图象向右平移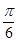 个单位,得到函数
个单位,得到函数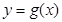 的图象,求函数
的图象,求函数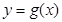 的对称中心及单调递增区间.
的对称中心及单调递增区间.
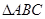 中,角
中,角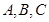 的对边分别为
的对边分别为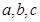 ,且
,且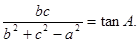
(1) 求角
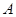 ;
;(2) 设函数
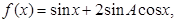 将函数
将函数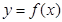 的图象上各点的纵坐标保持不变,横坐标缩短到原来的
的图象上各点的纵坐标保持不变,横坐标缩短到原来的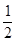 ,把所得图象向右平移
,把所得图象向右平移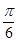 个单位,得到函数
个单位,得到函数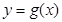 的图象,求函数
的图象,求函数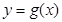 的对称中心及单调递增区间.
的对称中心及单调递增区间.(1) ;
;
(2),对称中心(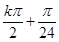 ,0),
,0),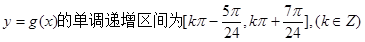
 ;
;(2),对称中心(
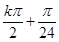 ,0),
,0),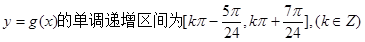
本试题主要是考查了三角函数的图像和性质的运用,以及运用三角形的正弦定理和余弦定理求解三角形的综合运用。
(1)中由于COSA的余弦定理表达式可以结合已知的表达式得到求解
(2)主要是体现了函数的图像变换,先化简原来的三角函数为单一三角函数,然后变换得到结论。并结合三角函数的性质得到对称中心和单调区间。
解:(1)因为
 ---------------3分
---------------3分
又 -------------------5分
-------------------5分
(2)由(1)得: ----------------6分
----------------6分
由题可得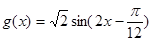 --------------------8分
--------------------8分
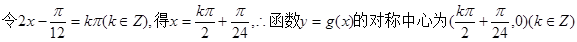 ------------------10分
------------------10分
令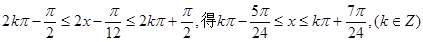
即函数 ---------------12分
---------------12分
(1)中由于COSA的余弦定理表达式可以结合已知的表达式得到求解
(2)主要是体现了函数的图像变换,先化简原来的三角函数为单一三角函数,然后变换得到结论。并结合三角函数的性质得到对称中心和单调区间。
解:(1)因为

 ---------------3分
---------------3分又
 -------------------5分
-------------------5分(2)由(1)得:
 ----------------6分
----------------6分由题可得
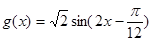 --------------------8分
--------------------8分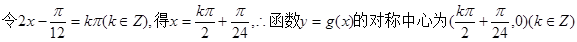 ------------------10分
------------------10分令
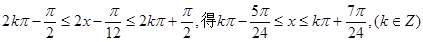
即函数
 ---------------12分
---------------12分
练习册系列答案
相关题目
 (
( ).
). 的最小正周期及单调递增区间;
的最小正周期及单调递增区间; 
 内角
内角 的对边长分别为
的对边长分别为 ,若
,若
 且
且 试求角B和角C.
试求角B和角C. 中,
中, 的对边分别为
的对边分别为 且
且 成等差数列.
成等差数列. 的范围.
的范围.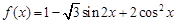 .
.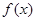 的最大值及取得最大值时的
的最大值及取得最大值时的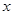 集合;
集合;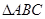 的角
的角 的对边分别为
的对边分别为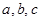 ,且
,且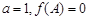 .求
.求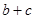 的取值范围
的取值范围 的单调递增区间的是
的单调递增区间的是




 在
在 上有两个不等的实数根
上有两个不等的实数根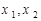 ,则
,则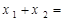 ( )
( )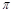
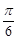
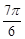
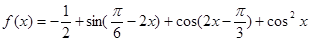
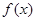 的最小正周期;
的最小正周期;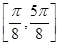 上的最大值,并求出
上的最大值,并求出 )的图象与x轴的交点中,相邻两个交点之间的距离为
)的图象与x轴的交点中,相邻两个交点之间的距离为
 时,求f(x)的值域.
时,求f(x)的值域. 的图象绕坐标原点逆时针方向旋转
的图象绕坐标原点逆时针方向旋转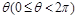 角,得到曲线
角,得到曲线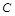 .若对于每一个旋转角
.若对于每一个旋转角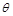 ,曲线
,曲线


