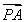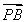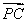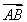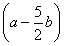题目内容
已知函数f(x)＝
sin2x＋sin xcos x，x∈
.
(1)求f(x) 的零点；
(2)求f(x)的最大值和最小值．
（1）零点为
或π（2）最大值为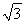
，最小值为－1＋
【解析】(1)令f(x)＝0得sin x·(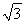
sin x＋cos x)＝0，
所以sin x＝0，或tan x＝－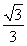
.
由sin x＝0，x∈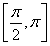
，得x＝π；
由tan x＝－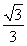
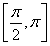，x∈，得x＝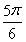
.
综上，函数f(x)在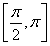上的零点为或π.
(2)f(x)＝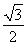
(1－cos 2x)＋
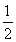sin 2x＝sin
＋.
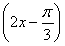因为x∈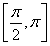，所以2x－
∈
.
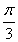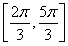当2x－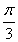
＝
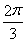，即x＝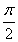
时，f(x)的最大值为；
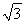当2x－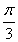
＝
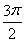，即x＝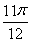
时，f(x)的最小值为－1＋.
练习册系列答案
一线名师提优试卷系列答案
阳光试卷单元测试卷系列答案
相关题目
