题目内容
9.在△ABC中,BC边上的垂直平分线与BC,AC分别交于点D,M,若$\overrightarrow{AM}•\overrightarrow{BC}$=6,且|$\overrightarrow{AB}$|=2.则|$\overrightarrow{AC}$|=( )| A. | $\sqrt{10}$ | B. | $\sqrt{6}$ | C. | 4 | D. | 2$\sqrt{2}$ |
分析 画出图形,并连接AD,$\overrightarrow{AM}=\overrightarrow{AD}+\overrightarrow{DM}$,再根据DM⊥BC即可得到$\overrightarrow{AM}•\overrightarrow{BC}=\overrightarrow{AD}•\overrightarrow{BC}$,而$\overrightarrow{AD}=\frac{1}{2}(\overrightarrow{AB}+\overrightarrow{AC})$,$\overrightarrow{BC}=\overrightarrow{AC}-\overrightarrow{AB}$,再根据$\overrightarrow{AM}•\overrightarrow{BC}=6,|\overrightarrow{AB}|=2$即可求出|$\overrightarrow{AC}$|.
解答  解:如图,DM⊥BC,∴$\overrightarrow{DM}•\overrightarrow{BC}=0$;
解:如图,DM⊥BC,∴$\overrightarrow{DM}•\overrightarrow{BC}=0$;
∴$\overrightarrow{AM}•\overrightarrow{BC}=(\overrightarrow{AD}+\overrightarrow{DM})•\overrightarrow{BC}$=$\overrightarrow{AD}•\overrightarrow{BC}=\frac{1}{2}(\overrightarrow{AB}+\overrightarrow{AC})•(\overrightarrow{AC}-\overrightarrow{AB})$=$\frac{1}{2}({\overrightarrow{AC}}^{2}-{\overrightarrow{AB}}^{2})$;
∵$|\overrightarrow{AB}|=2,\overrightarrow{AM}•\overrightarrow{BC}=6$;
∴$|\overrightarrow{AC}|=4$.
故选:C.
点评 考查向量加法、减法的几何意义,两非零向量垂直的充要条件,向量的数量积的运算.

 学业测评一课一测系列答案
学业测评一课一测系列答案 小学课时作业全通练案系列答案
小学课时作业全通练案系列答案
| A. | $\frac{2}{3}$ | B. | $\frac{1}{3}$ | C. | $\frac{\sqrt{6}}{3}$ | D. | $\frac{\sqrt{6}}{2}$ |
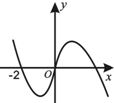
 已知函数f(x)的导函数f′(x)的图象如图所示,那么函数f(x)的图象最有可能的是图中的( )
已知函数f(x)的导函数f′(x)的图象如图所示,那么函数f(x)的图象最有可能的是图中的( )


 如图,在四棱锥P-ABCD中,底面ABCD为直角梯形,AD∥BC,AD⊥DC,平面PAD⊥底面ABCD,Q为AD的中点,M是棱PC的中点,PA=PD=2,BC=$\frac{1}{2}$AD=1,CD=$\sqrt{3}$.
如图,在四棱锥P-ABCD中,底面ABCD为直角梯形,AD∥BC,AD⊥DC,平面PAD⊥底面ABCD,Q为AD的中点,M是棱PC的中点,PA=PD=2,BC=$\frac{1}{2}$AD=1,CD=$\sqrt{3}$. 如图,三棱柱ABC-A1B1C1中,A1A⊥平面ABC,AC=BC,AB=2A1A=4.以AB,BC为邻边作平行四边形ABCD,连接A1D和DC1.
如图,三棱柱ABC-A1B1C1中,A1A⊥平面ABC,AC=BC,AB=2A1A=4.以AB,BC为邻边作平行四边形ABCD,连接A1D和DC1.