题目内容
已知函数f(x)=cos2x+cos(2x- ),给出下列结论:
),给出下列结论:
①f(x)是最小正周期为π的偶函数;
②f(x)的图象关于 对称;
对称;
③f(x)的最大值为2;
④将函数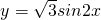 的图象向左平移
的图象向左平移 就得到y=f(x)的图象.
就得到y=f(x)的图象.
其中正确的是
- A.①②
- B.②③
- C.②④
- D.③④
C
分析:先利用两角差的余弦公式和两角和的正弦公式,将函数f(x)化为y=Asin(ωx+φ)型函数,再利用正弦函数的图象和性质及函数图象变换理论,逐一判断正误即可
解答:函数f(x)=cos2x+cos(2x- )=cos2x+
)=cos2x+ cos2x+
cos2x+ sin2x=
sin2x=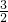 cos2x+
cos2x+ sin2x
sin2x
= (
( cos2x+
cos2x+ sin2x)=
sin2x)= sin(2x+
sin(2x+ ),
),
∵f(x)为非奇非偶函数,故①错误;
将x= 代入t=2x+
代入t=2x+ ,得t=
,得t= ,而x=
,而x= 为正弦函数的对称轴,故②正确;
为正弦函数的对称轴,故②正确;
显然f(x)的最大值为 ,③错误;
,③错误;
将函数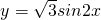 的图象向左平移
的图象向左平移 就得到y=
就得到y= sin2(x+
sin2(x+ )=
)= sin(2x+
sin(2x+ )=f(x),故④正确
)=f(x),故④正确
故选 C
点评:本题主要考查了三角变换公式在化简函数中的应用,y=Asin(ωx+φ)型函数的图象和性质,函数图象的平移变换,属基础题
分析:先利用两角差的余弦公式和两角和的正弦公式,将函数f(x)化为y=Asin(ωx+φ)型函数,再利用正弦函数的图象和性质及函数图象变换理论,逐一判断正误即可
解答:函数f(x)=cos2x+cos(2x-
 )=cos2x+
)=cos2x+ cos2x+
cos2x+ sin2x=
sin2x=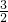 cos2x+
cos2x+ sin2x
sin2x=
 (
( cos2x+
cos2x+ sin2x)=
sin2x)= sin(2x+
sin(2x+ ),
),∵f(x)为非奇非偶函数,故①错误;
将x=
 代入t=2x+
代入t=2x+ ,得t=
,得t= ,而x=
,而x= 为正弦函数的对称轴,故②正确;
为正弦函数的对称轴,故②正确;显然f(x)的最大值为
 ,③错误;
,③错误;将函数
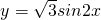 的图象向左平移
的图象向左平移 就得到y=
就得到y= sin2(x+
sin2(x+ )=
)= sin(2x+
sin(2x+ )=f(x),故④正确
)=f(x),故④正确故选 C
点评:本题主要考查了三角变换公式在化简函数中的应用,y=Asin(ωx+φ)型函数的图象和性质,函数图象的平移变换,属基础题

练习册系列答案
相关题目
已知函数f(x)=
,则关于x的方程f2(x)+bf(x)+c=0有5个不同实数解的充要条件是( )
|
| A、b<-2且c>0 |
| B、b>-2且c<0 |
| C、b<-2且c=0 |
| D、b≥-2且c=0 |
 已知函数f(x)的图象如图所示,则函数的值域为( )
已知函数f(x)的图象如图所示,则函数的值域为( )