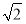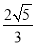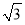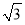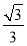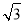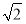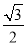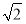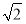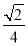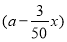题目内容

已知函数f(x)＝coscos－sin xcos x＋

(1)求函数f(x)的最小正周期和最大值；

(2)求函数f(x)单调递增区间．

 

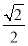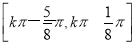（1）最小正周期为T＝π，最大值为（2），k∈Z

【解析】(1)∵f(x)＝coscos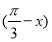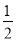－sin 2x＋

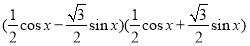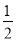＝－sin 2x＋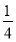

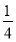＝cos2x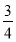－sin2x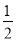－sin 2x＋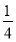

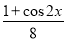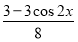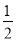＝－－sin 2x＋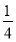

＝ (cos 2x－sin 2x)＝cos，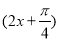

函数f(x)的最小正周期为T＝π，

函数f(x)的最大值为.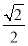

(2)由2kπ－π≤2x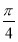＋≤2kπ，k∈Z，

得kπ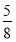－π≤x≤kπ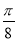－，k∈Z，

故函数f(x)的单调递增区间为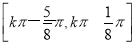，k∈Z.

 

练习册系列答案
相关题目

已知椭圆C：＝1(a＞b＞0)的离心率为，其左、右焦点分别是F1、F2，过点F1的直线l交椭圆C于E、G两点，且△EGF2的周长为4.

(1)求椭圆C的方程；

(2)若过点M(2,0)的直线与椭圆C相交于两点A、B，设P为椭圆上一点，且满足＋＝t (O为坐标原点)，当|－|＜时，求实数t的取值范围．

 

记[x]为不超过实数x的最大整数．例如，[2]＝2，[1.5]＝1，[－0.3]＝－1.设a为正整数，数列{xn}满足x1＝a，xn＋1＝ (n∈N*)．现有下列命题：

①当a＝5时，数列{xn}的前3项依次为5,3,1；

②对数列{xn}都存在正整数k，当n≥k时总有xn＝xk；

③当n≥1时，xn＞－1；

④对某个正整数k，若xk＋1≥xk，则xk＝[]．

其中的真命题有________．(写出所有真命题的编号)

 

已知数列{an}为等差数列，且a1＋a7＋a13＝4π，则tan(a2＋a12)＝ (　　)．

A．－ B.

C．± D．－

 

在△ABC中，角A，B，C所对的边分别为a，b，c，若角A，B，C依次成等差数列，a＝1，b＝，则S△ABC等于(　　)．

A. B. C. D．2

 

已知锐角A，B满足2tan A＝tan(A＋B)，则tan B的最大值为(　　)．

A．2 B. C. D.

 

函数f(x)＝x(a＞0)的单调递减区间是________．

 

某镇政府为了更好地服务于农民，派调查组到某村考察．据了解，该村有100户农民，且都从事蔬菜种植，平均每户的年收入为3万元．为了调整产业结构，该镇政府决定动员部分农民从事蔬菜加工．据估计，若能动员x(x＞0)户农民从事蔬菜加工，则剩下的继续从事蔬菜种植的农民平均每户的年收入有望提高2x%，而从事蔬菜加工的农民平均每户的年收入将为3 (a＞0)万元．

(1)在动员x户农民从事蔬菜加工后，要使从事蔬菜种植的农民的总年收入不低于动员前从事蔬菜种植的农民的总年收入，求x的取值范围；

(2)在(1)的条件下，要使这100户农民中从事蔬菜加工的农民的总年收入始终不高于从事蔬菜种植的农民的总年收入，求a的最大值．

 

如图，AB是圆O的直径，点C在圆O上，延长BC到D使BC＝CD，过C作圆O的切线交AD于E.若AB＝6，ED＝2，则BC＝________.

 

 

违法和不良信息举报电话：027-86699610 举报邮箱：58377363@163.com

精英家教网