题目内容
已知函数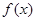 定义在区间
定义在区间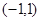 上,
上,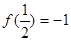 ,且当
,且当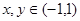 时,
时,
恒有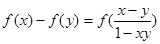 .又数列
.又数列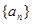 满足
满足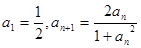 .
.
(1)证明: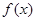 在
在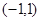 上是奇函数;
上是奇函数;
(2)求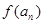 的表达式;
的表达式;
(3)设 为数列
为数列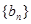 的前
的前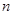 项和,若
项和,若 对
对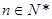 恒成立,求
恒成立,求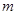 的最小值.
的最小值.
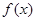 定义在区间
定义在区间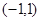 上,
上,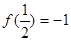 ,且当
,且当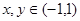 时,
时,恒有
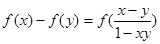 .又数列
.又数列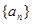 满足
满足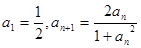 .
.(1)证明:
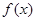 在
在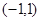 上是奇函数;
上是奇函数;(2)求
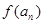 的表达式;
的表达式;(3)设
 为数列
为数列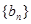 的前
的前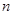 项和,若
项和,若 对
对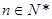 恒成立,求
恒成立,求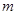 的最小值.
的最小值.(Ⅰ)证明略
(Ⅱ)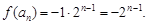
(III) m的最小值为7.
(Ⅱ)
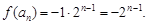
(III) m的最小值为7.
本试题主要是考查了函数与数列的综合运用
(1)通过赋值法得到函数奇偶性的判定。
(2)因为令x=an,y=-an,于是,由已知得2f (an)="f" (an+1),从而求解得到解析式。
(3)由(II)得f(an+1)=-2n,那么整体思想得到参数m的最值。
(1)通过赋值法得到函数奇偶性的判定。
(2)因为令x=an,y=-an,于是,由已知得2f (an)="f" (an+1),从而求解得到解析式。
(3)由(II)得f(an+1)=-2n,那么整体思想得到参数m的最值。

练习册系列答案
相关题目
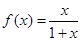
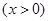 ,数列
,数列 满足:
满足: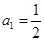 ,
,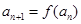
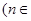 N*
N* .
.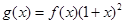 ,数列
,数列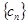 满足:
满足: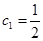 ,
,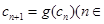 N*),
N*), 的正整数,都满足:
的正整数,都满足: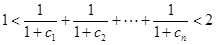 .
. 是等差数列,且a2+ a5+ a8+ a11=48,则a6+ a7= ( )
是等差数列,且a2+ a5+ a8+ a11=48,则a6+ a7= ( ) 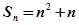 ,那么它的通项公式为an="_________" .
,那么它的通项公式为an="_________" .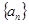 中,
中,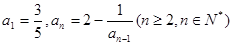 ,数列
,数列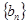 满足
满足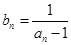 .
. 的通项公式为
的通项公式为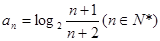 ,设其前n项和为Sn,
,设其前n项和为Sn,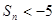 成立的自然数n( )
成立的自然数n( )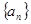 中,3(
中,3( +
+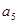 )+2(a
)+2(a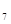 +
+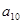 +
+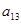 )=24,则此数列前13项之和( )
)=24,则此数列前13项之和( )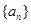 中,
中,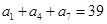 ,
,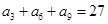 ,则数列
,则数列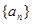 中,已知
中,已知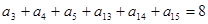 ,则
,则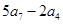 = .
= .