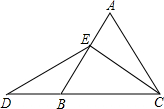
题目内容
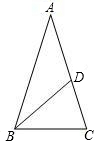
在等边△ABC中,点E在线段AB上,点D在CB的延长线上,且ED=EC.
(1)当点E为线段AB的中点时,试求
的值;
(2)当点E不是线段AB的中点时,
的值是否发生变化?为什么?
(1)当点E为线段AB的中点时,试求
|
(2)当点E不是线段AB的中点时,
|

(1)过点E作EF⊥BC于F,设等边三角形的边长为2a,
∵点E为线段AB的中点,
∴AE=BE=
×2a=a,∠BCE=30°,
∵ED=EC,
∴∠D=∠BCE=30°,
∴∠BED=∠ABC-∠D=60°-30°=30°,
∴BD=BE=a,
=
=
;
(2)不变.理由如下:
过点E作EF⊥BC于F,设BD=x,等边三角形的边长为2a,
∵ED=EC,
∴CF=
CD=
(2a+x),
∴BF=BC-CF=2a-
(2a+x)=a-
x,
在Rt△BEF中,BE=2BF=2a-x,
∴AE=AB-BE=2a-(2a-x)=x,
∴
=
=
.
∵点E为线段AB的中点,
∴AE=BE=
| 1 |
| 2 |
∵ED=EC,
∴∠D=∠BCE=30°,

∴∠BED=∠ABC-∠D=60°-30°=30°,
∴BD=BE=a,
|
|
| 1 |
| 2 |
(2)不变.理由如下:
过点E作EF⊥BC于F,设BD=x,等边三角形的边长为2a,
∵ED=EC,
∴CF=
| 1 |
| 2 |
| 1 |
| 2 |
∴BF=BC-CF=2a-
| 1 |
| 2 |
| 1 |
| 2 |
在Rt△BEF中,BE=2BF=2a-x,
∴AE=AB-BE=2a-(2a-x)=x,
∴
|
|
| 1 |
| 2 |

练习册系列答案
 阅读快车系列答案
阅读快车系列答案
相关题目