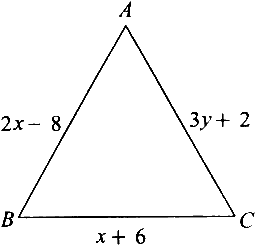题目内容
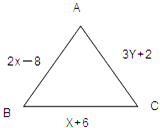 已知三角形ABC为等边三角形,其三边长如下图所示,求
已知三角形ABC为等边三角形,其三边长如下图所示,求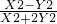 ×1
×1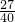 的值.
的值.
解:根据题意有:
2x-8=x+6,
2x-x=6+8,
x=14;
3y+2=14+6,
3y+2-2=14+6-2,
3y=18,
y=6.
把 x=14,y=6代入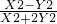 ×1
×1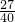 ,
,
原式=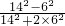 ×
×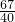 ,
,
=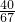 ×
×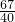 ,
,
=1.
答: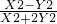 ×1
×1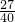 的值为1.
的值为1.
分析:根据等边三角形的性质可得方程2x-8=x+6,解方程求出x=14;再根据等边三角形的性质可得方程3y+2=14+6,解方程求出y;再将x,y的值代入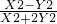 ×1
×1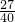 ,计算即可求解.
,计算即可求解.
点评:考查了等边三角形的性质,解方程及含字母式子的求值,本题的关键是得到关于x,y的方程,求得x,y的值.
2x-8=x+6,
2x-x=6+8,
x=14;
3y+2=14+6,
3y+2-2=14+6-2,
3y=18,
y=6.
把 x=14,y=6代入
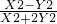 ×1
×1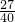 ,
,原式=
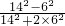 ×
×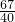 ,
,=
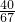 ×
×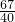 ,
,=1.
答:
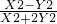 ×1
×1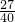 的值为1.
的值为1.分析:根据等边三角形的性质可得方程2x-8=x+6,解方程求出x=14;再根据等边三角形的性质可得方程3y+2=14+6,解方程求出y;再将x,y的值代入
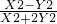 ×1
×1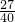 ,计算即可求解.
,计算即可求解.点评:考查了等边三角形的性质,解方程及含字母式子的求值,本题的关键是得到关于x,y的方程,求得x,y的值.

练习册系列答案
 举一反三同步巧讲精练系列答案
举一反三同步巧讲精练系列答案 口算与应用题卡系列答案
口算与应用题卡系列答案 名师点睛字词句段篇系列答案
名师点睛字词句段篇系列答案
相关题目
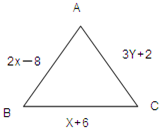 已知三角形ABC为等边三角形,其三边长如下图所示,求
已知三角形ABC为等边三角形,其三边长如下图所示,求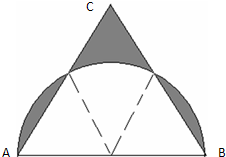 已知等边三角形ABC,边长是6厘米,以AB为直径画半圆(如图),求阴影部分的面积.(π取3.14)
已知等边三角形ABC,边长是6厘米,以AB为直径画半圆(如图),求阴影部分的面积.(π取3.14)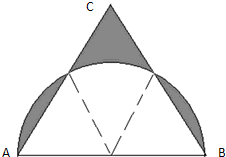 已知等边三角形ABC,边长是6厘米,以AB为直径画半圆(如图),求阴影部分的面积.(π取3.14)
已知等边三角形ABC,边长是6厘米,以AB为直径画半圆(如图),求阴影部分的面积.(π取3.14)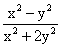 ×1
×1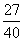 的值。
的值。