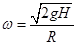题目内容
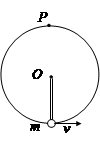
如图所示,质量M=2kg的滑块套在光滑的水平轨道上,质量m=1kg的小球通过长L=0.5m的轻质细杆与滑块上的光滑轴O连接,小球和轻杆可在竖直平面内绕O轴自由转动,开始轻杆处于水平状态,现给小球一个竖直向上的初速度v0="4" m/s,g取10m/s2。
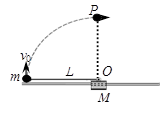
(1)若锁定滑块,试求小球通过最高点P时对轻杆的作用力大小和方向。
(2)若解除对滑块的锁定,试求小球通过最高点时的速度大小。
(3)在满足(2)的条件下,试求小球击中滑块右侧轨道位置点与小球起始位置点间的距离。

(1)若锁定滑块,试求小球通过最高点P时对轻杆的作用力大小和方向。
(2)若解除对滑块的锁定,试求小球通过最高点时的速度大小。
(3)在满足(2)的条件下,试求小球击中滑块右侧轨道位置点与小球起始位置点间的距离。
(1)2N(2)2m/s(3)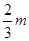
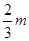
(1)设小球能通过最高点,且此时的速度为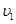 ,在上升过程中,因只有重力做功,小球的机械能守恒。则
,在上升过程中,因只有重力做功,小球的机械能守恒。则
 ①
①
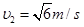 ②
②
设小球到达最高点时,轻杆对小球的作用力为F,方向向下,则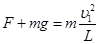 ③
③
由②③式,得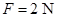 ④
④
由牛顿第三定律可知,小球对轻杆的作用力大小为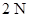 ,方向竖直向上。
,方向竖直向上。
(2)解除锁定后,设小球通过最高点时的速度为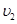 ,此时滑块的速度为V。在上升过程中,因系统在水平方向不受外力作用,水平方向的动量守恒。以水平向右的方向为正方向,有
,此时滑块的速度为V。在上升过程中,因系统在水平方向不受外力作用,水平方向的动量守恒。以水平向右的方向为正方向,有
 ⑤
⑤
在上升过程中,因只有重力做功,系统的机械能守恒,则
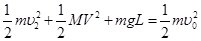 ⑥
⑥
由⑤⑥式,得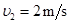 ⑦
⑦
(3)设小球击中滑块右侧轨道的位置点与小球起始位置点间的距离为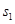 ,滑块向左移动的距离为
,滑块向左移动的距离为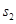 ,任意时刻小球的水平速度大小为
,任意时刻小球的水平速度大小为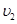 ,滑块的速度大小为
,滑块的速度大小为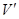 。由系统水平方向的动量守恒,得
。由系统水平方向的动量守恒,得 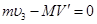 ⑧
⑧
将⑧式两边同乘以 ,得
,得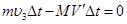 ⑨
⑨
因⑨式对任意时刻附近的微小间隔 都成立,累积相加后,有
都成立,累积相加后,有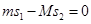 ⑩
⑩
又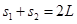
由⑩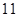 式得
式得 
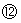
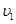 ,在上升过程中,因只有重力做功,小球的机械能守恒。则
,在上升过程中,因只有重力做功,小球的机械能守恒。则 ①
① 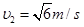 ②
②设小球到达最高点时,轻杆对小球的作用力为F,方向向下,则
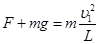 ③
③由②③式,得
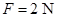 ④
④由牛顿第三定律可知,小球对轻杆的作用力大小为
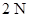 ,方向竖直向上。
,方向竖直向上。(2)解除锁定后,设小球通过最高点时的速度为
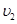 ,此时滑块的速度为V。在上升过程中,因系统在水平方向不受外力作用,水平方向的动量守恒。以水平向右的方向为正方向,有
,此时滑块的速度为V。在上升过程中,因系统在水平方向不受外力作用,水平方向的动量守恒。以水平向右的方向为正方向,有 ⑤
⑤ 在上升过程中,因只有重力做功,系统的机械能守恒,则
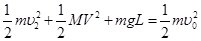 ⑥
⑥由⑤⑥式,得
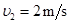 ⑦
⑦(3)设小球击中滑块右侧轨道的位置点与小球起始位置点间的距离为
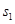 ,滑块向左移动的距离为
,滑块向左移动的距离为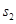 ,任意时刻小球的水平速度大小为
,任意时刻小球的水平速度大小为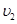 ,滑块的速度大小为
,滑块的速度大小为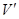 。由系统水平方向的动量守恒,得
。由系统水平方向的动量守恒,得 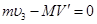 ⑧
⑧将⑧式两边同乘以
 ,得
,得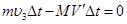 ⑨
⑨因⑨式对任意时刻附近的微小间隔
 都成立,累积相加后,有
都成立,累积相加后,有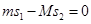 ⑩
⑩又
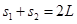
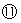
由⑩
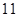 式得
式得 

练习册系列答案
 小天才课时作业系列答案
小天才课时作业系列答案 一课四练系列答案
一课四练系列答案 黄冈小状元满分冲刺微测验系列答案
黄冈小状元满分冲刺微测验系列答案 新辅教导学系列答案
新辅教导学系列答案 阳光同学一线名师全优好卷系列答案
阳光同学一线名师全优好卷系列答案
相关题目
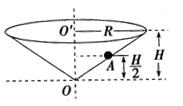
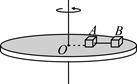
 ,杆以O为支点绕竖直线旋转.质量为m的小环套在杆上可沿杆滑动,当杆的角速度为ω1时,小环旋转平面在A处;当杆角速度为ω2时,小环旋转平面在B处,设环在A、B两处对杆的压力分别为N1、N2.则有( )
,杆以O为支点绕竖直线旋转.质量为m的小环套在杆上可沿杆滑动,当杆的角速度为ω1时,小环旋转平面在A处;当杆角速度为ω2时,小环旋转平面在B处,设环在A、B两处对杆的压力分别为N1、N2.则有( )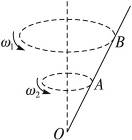

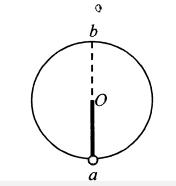
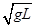
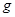 ,则
,则