题目内容
函数
的单调递增区间是（ ）A．（2kπ-
π，2kπ-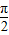
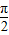）（k∈Z）B．（2kπ-
，2kπ+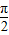
）（k∈Z）C．（2kπ-
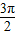，2k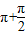
）（k∈Z）D．（kπ-
，k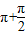
）（k∈Z）
【答案】分析：利用三角函数的恒等变换化简函数的解析式为 tan（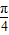
+
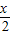），令 kπ-
＜
+
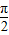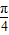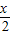＜kπ+
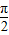，k∈z，求得x的范围，可得函数的增区间．
解答：解：由于函数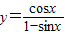
=
=
=
=
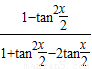
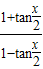=tan（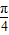
+
），
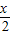令 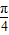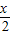kπ-
＜
+
＜kπ+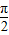
，k∈z，求得 x∈（2kπ-
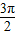，2k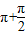
）（k∈Z），
故函数的增区间为（2kπ-
，2k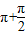
）（k∈Z），
故选C．
点评：本题主要考查三角函数的恒等变换及化简求值，正切函数的增区间，属于中档题．
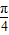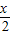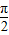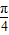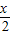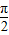+），令 kπ-＜+＜kπ+，k∈z，求得x的范围，可得函数的增区间．解答：解：由于函数
===
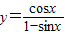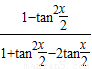
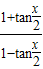==tan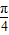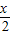（+），令 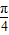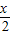kπ-
＜+＜kπ+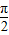，k∈z，求得 x∈（2kπ-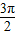，2k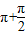）（k∈Z），故函数的增区间为（2kπ-
，2k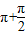）（k∈Z），故选C．
点评：本题主要考查三角函数的恒等变换及化简求值，正切函数的增区间，属于中档题．
练习册系列答案
相关题目
