题目内容
已知f(x)是周期为2的奇函数,当x∈(0,1)时,f(x)=2x,则f(log
23)值( )
| 1 |
| 2 |
分析:先求出-5 log2
<-4,log2
∈(0,1),由f(x)是周期为2的奇函数,可得f (log
23)=-f(log2
),根据 当x∈(0,1)时,f(x)=2x ,可求得-f(log2
) 的值,从而得到要求的式子的值.
| 1 |
| 23 |
| 23 |
| 16 |
| 1 |
| 2 |
| 23 |
| 16 |
| 23 |
| 16 |
解答:解:∵log
23=log2
,
<
<
,∴-5 log2
<-4,
∴-1<log2
+4<0,且 log2
+4=log2
,故 -log2
=log2
∈(0,1).
由f(x)是周期为2的奇函数,可得f (log
23)=f(log2
+4)=f (log2
)=-f(-log2
)=-f(log2
).
∵当x∈(0,1)时,f(x)=2x ,
∴-f(log2
)=-2log2
=-
.
故f(log
23)=-f(log2
)=-
,
故选C.
| 1 |
| 2 |
| 1 |
| 23 |
| 1 |
| 32 |
| 1 |
| 23 |
| 1 |
| 16 |
| 1 |
| 23 |
∴-1<log2
| 1 |
| 23 |
| 1 |
| 23 |
| 16 |
| 23 |
| 16 |
| 23 |
| 23 |
| 16 |
由f(x)是周期为2的奇函数,可得f (log
| 1 |
| 2 |
| 1 |
| 23 |
| 16 |
| 23 |
| 16 |
| 23 |
| 23 |
| 16 |
∵当x∈(0,1)时,f(x)=2x ,
∴-f(log2
| 23 |
| 16 |
| 23 |
| 16 |
| 23 |
| 16 |
故f(log
| 1 |
| 2 |
| 23 |
| 16 |
| 23 |
| 16 |
故选C.
点评:本题主要考查函数的周期性和奇偶性的综合应用,对数恒等式,体现了转化的数学思想,求得f(log
23)=-f(log2
),是解题的关键,属于基础题.
| 1 |
| 2 |
| 23 |
| 16 |

练习册系列答案
相关题目
已知f(x)是周期为2的奇函数,当0<x<1时,f(x)=lgx.设a=f(
),b=f(
),c=f(
),则( )
| 6 |
| 5 |
| 3 |
| 2 |
| 5 |
| 2 |
| A、a<b<c |
| B、b<a<c |
| C、c<b<a |
| D、c<a<b |
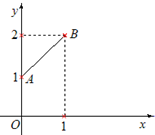 已知f(x)是周期为2的偶函数.当0≤x≤1时,f(x)的图象是如图中的线段AB,那么
已知f(x)是周期为2的偶函数.当0≤x≤1时,f(x)的图象是如图中的线段AB,那么