题目内容
已知椭圆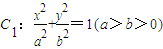 的长轴长为4,离心率为
的长轴长为4,离心率为 ,F1,F2分别为其左右焦点.一动圆过点F2,且与直线x=-1相切.
,F1,F2分别为其左右焦点.一动圆过点F2,且与直线x=-1相切.(Ⅰ) (ⅰ)求椭圆C1的方程;(ⅱ)求动圆圆心轨迹C的方程;
(Ⅱ)在曲线C上有四个不同的点M,N,P,Q,满足
 与
与 共线,
共线,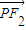 与
与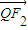 共线,且
共线,且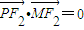 ,求四边形PMQN面积的最小值.
,求四边形PMQN面积的最小值.
【答案】分析:(Ⅰ)利用待定系数法求出椭圆C1的a,b,c即可;因一动圆过点F2,且与直线x=-1相切可得此圆心到定点和到定直线的距离相等,它是抛物线,从而解决;
(Ⅱ)欲求四边形PMQN面积的最小值,先建立面积关于某一个变量的函数关系式,设直线MN的方程为:y=k(x-1)
利用抛物线定义求出|MN|,再结合向量垂直关系求得|PQ|,最后利用基本不等式求出所列函数的最小值即可.
解答:解:(Ⅰ)(ⅰ)由已知可得 ,
,
则所求椭圆方程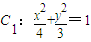 .
.
(ⅱ)由已知可得动圆圆心轨迹为抛物线,且抛物线C的焦点为(1,0),准线方程为x=-1,则动圆圆心轨迹方程为C:y2=4x.
(Ⅱ)由题设知直线MN,PQ的斜率均存在且不为零
设直线MN的斜率为k(k≠0),M(x1,y1),N(x2,y2),则直线MN的方程为:y=k(x-1)
联立C:y2=4x消去y可得k2x2-(2k2+4)x+k2=0
由抛物线定义可知:
设直线PQ的方程为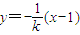 ,与椭圆的方程联立得
,与椭圆的方程联立得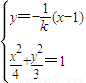
化简后,利用弦长公式可得|PQ|=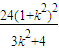
又
令1+k2=t>1
故有
又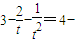
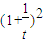 ∈(0,3)
∈(0,3)
可得
所以四边形PMQN面积的最小值为8.
点评:本小题主要考查曲线与方程,直线和圆锥曲线等基础知识,以及求平面图形面积最小值的基本技能和综合运用数学知识解决问题的能力.
(Ⅱ)欲求四边形PMQN面积的最小值,先建立面积关于某一个变量的函数关系式,设直线MN的方程为:y=k(x-1)
利用抛物线定义求出|MN|,再结合向量垂直关系求得|PQ|,最后利用基本不等式求出所列函数的最小值即可.
解答:解:(Ⅰ)(ⅰ)由已知可得
 ,
,则所求椭圆方程
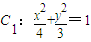 .
.(ⅱ)由已知可得动圆圆心轨迹为抛物线,且抛物线C的焦点为(1,0),准线方程为x=-1,则动圆圆心轨迹方程为C:y2=4x.
(Ⅱ)由题设知直线MN,PQ的斜率均存在且不为零
设直线MN的斜率为k(k≠0),M(x1,y1),N(x2,y2),则直线MN的方程为:y=k(x-1)
联立C:y2=4x消去y可得k2x2-(2k2+4)x+k2=0
由抛物线定义可知:

设直线PQ的方程为
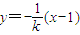 ,与椭圆的方程联立得
,与椭圆的方程联立得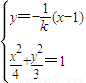
化简后,利用弦长公式可得|PQ|=
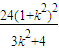
又

令1+k2=t>1
故有

又
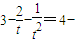
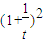 ∈(0,3)
∈(0,3)可得

所以四边形PMQN面积的最小值为8.
点评:本小题主要考查曲线与方程,直线和圆锥曲线等基础知识,以及求平面图形面积最小值的基本技能和综合运用数学知识解决问题的能力.

练习册系列答案
相关题目

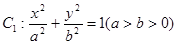 的长轴长为4,离心率为
的长轴长为4,离心率为 ,
, 分别为其左右焦点.一动圆过点
分别为其左右焦点.一动圆过点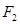 ,且与直线
,且与直线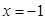 相切.
相切.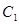 的方程;
(ⅱ)求动圆圆心
的方程;
(ⅱ)求动圆圆心 的轨迹方程;
的轨迹方程; ,椭圆
,椭圆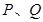 ,满足
,满足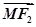 与
与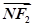 共线,
共线,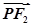 与
与 共线,且
共线,且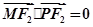 ,求四边形
,求四边形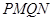 面积的最小值.
面积的最小值.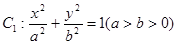 的长轴长为4,离心率为
的长轴长为4,离心率为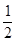 ,
, 分别为其左右焦点.一动圆过点
分别为其左右焦点.一动圆过点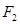 ,且与直线
,且与直线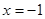 相切.
相切. 的方程; (ⅱ)求动圆圆心
的方程; (ⅱ)求动圆圆心 轨迹的方程;
轨迹的方程;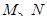 ,椭圆
,椭圆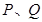 ,满足
,满足 与
与 共线,
共线,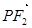 与
与 共线,且
共线,且 ,求四边形
,求四边形 面积的最小值.
面积的最小值. 的长轴长为4,且点
的长轴长为4,且点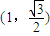 在该椭圆上.
在该椭圆上.