题目内容
12.对于向量$\overrightarrow{{a}_{1}}$、$\overrightarrow{{a}_{2}}$、$\overrightarrow{{a}_{3}}$,记$\overrightarrow{{S}_{3}}$=$\overrightarrow{{a}_{1}}$+$\overrightarrow{{a}_{2}}$+$\overrightarrow{{a}_{3}}$,对于$\overrightarrow{{a}_{k}}$(k∈{1,2,3})如果有|$\overrightarrow{{a}_{k}}$|=|$\overrightarrow{{S}_{3}}$-$\overrightarrow{{a}_{k}}$|,则称向量$\overrightarrow{{a}_{k}}$是这一向量的“等横向量”.(1)判断向量$\overrightarrow{{a}_{1}}$=(2,2),是否是向量组$\overrightarrow{{a}_{1}}$=(2,2)、$\overrightarrow{{a}_{2}}$=(sinα,sinα)、$\overrightarrow{{a}_{3}}$=(cosα,cosα的“等横向量”,并说明理由;
(2)如果向量组$\overrightarrow{{a}_{1}}$=(sinx,cosx)、$\overrightarrow{{a}_{2}}$(sin2x,cos2x)、$\overrightarrow{{a}_{3}}$(sin3x,cos3x)中的每一个向量都是它的“等横向量”,求x的值;
(3)如果向量$\overrightarrow{{a}_{1}}$=(u,v)、$\overrightarrow{{a}_{2}}$=(sinα、sinα)、$\overrightarrow{{a}_{3}}$=(cosα,cosα)中的每一个向量都是它的“等横向量”,求u+v的取值范围.
分析 (1)$\overrightarrow{{S}_{3}}$=(2+sinα+cosα,2+sinα+cosα),可得$|\overrightarrow{{a}_{1}}|$=$2\sqrt{2}$,$|\overrightarrow{{S}_{3}}-\overrightarrow{{a}_{1}}|$=$\sqrt{2+2sin2α}$≤2,可得$|\overrightarrow{{a}_{1}}|$≠$|\overrightarrow{{S}_{3}}-\overrightarrow{{a}_{1}}|$,即可判断出.
(2)由已知可得:$|\overrightarrow{{a}_{1}}|=|\overrightarrow{{a}_{2}}|=|\overrightarrow{{a}_{3}}|$=1,$|\overrightarrow{{S}_{3}}-\overrightarrow{{a}_{1}}|$=$\sqrt{2+2cosx}$=1,化为cosx=-$\frac{1}{2}$;同理由$|\overrightarrow{{S}_{3}}-\overrightarrow{{a}_{2}}|$=1,化为cos2x=-$\frac{1}{2}$;由$|\overrightarrow{{S}_{3}}-\overrightarrow{{a}_{3}}|$=$\sqrt{2+2cosx}$=1,化为cosx=-$\frac{1}{2}$.联立$\left\{\begin{array}{l}{cosx=-\frac{1}{2}}\\{cos2x=-\frac{1}{2}}\end{array}\right.$,解得x即可得出.
(3)由已知可得:$|\overrightarrow{{a}_{1}}|$=$\sqrt{{u}^{2}+{v}^{2}}$,$|\overrightarrow{{a}_{2}}|=|\overrightarrow{{a}_{3}}|$=1.由向量$\overrightarrow{{a}_{1}}$,$\overrightarrow{{a}_{2}}$,$\overrightarrow{{a}_{3}}$中的每一个向量都是它的“等横向量”.可得$\sqrt{{u}^{2}+{v}^{2}}$=$|\overrightarrow{{S}_{3}}-\overrightarrow{{a}_{1}}|$=$\sqrt{2+2sin2α}$,化为u2+v2=2+2sin2α,由$|\overrightarrow{{S}_{3}}-\overrightarrow{{a}_{2}}|$=$\sqrt{2si{n}^{2}α}$,化为u2+v2+2(u+v)cosα+2cos2α=0;由$|\overrightarrow{{S}_{3}}-\overrightarrow{{a}_{3}}|$=$\sqrt{2co{s}^{2}α}$,化为u2+v2+2(u+v)sinα-2cos2α=0.化为(cosα+sinα)(u+v)=2+2sin2α=2(cosα+sinα)2,分类讨论:当cosα+sinα≠0时,当cosα+sinα=0时,即可得出.
解答 解:(1)$\overrightarrow{{S}_{3}}$=(2+sinα+cosα,2+sinα+cosα),
∵$|\overrightarrow{{a}_{1}}|$=$\sqrt{{2}^{2}+{2}^{2}}$=$2\sqrt{2}$,$|\overrightarrow{{S}_{3}}-\overrightarrow{{a}_{1}}|$=$\sqrt{(sinα+cosα)^{2}+(sinα+cosα)^{2}}$=$\sqrt{2+2sin2α}$≤2,$|\overrightarrow{{a}_{1}}|$≠$|\overrightarrow{{S}_{3}}-\overrightarrow{{a}_{1}}|$,
因此向量$\overrightarrow{{a}_{1}}$=(2,2)不是“等横向量”;
(2)∵向量组$\overrightarrow{{a}_{1}}$=(sinx,cosx)、$\overrightarrow{{a}_{2}}$(sin2x,cos2x)、$\overrightarrow{{a}_{3}}$(sin3x,cos3x),
∴$\overrightarrow{{S}_{3}}$=(sinx+sin2x+sin3x,cosx+cos2x+cos3x),
∴$|\overrightarrow{{a}_{1}}|=|\overrightarrow{{a}_{2}}|=|\overrightarrow{{a}_{3}}|$=1,由$|\overrightarrow{{S}_{3}}-\overrightarrow{{a}_{1}}|$=$\sqrt{(sin2x+sin3x)^{2}+(cos2x+cos3x)^{2}}$=$\sqrt{2+2cosx}$=1,化为cosx=-$\frac{1}{2}$;
同理由$|\overrightarrow{{S}_{3}}-\overrightarrow{{a}_{2}}|$=$\sqrt{2+cos2x}$=1,化为cos2x=-$\frac{1}{2}$;
由$|\overrightarrow{{S}_{3}}-\overrightarrow{{a}_{3}}|$=$\sqrt{2+2cosx}$=1,化为cosx=-$\frac{1}{2}$.
联立$\left\{\begin{array}{l}{cosx=-\frac{1}{2}}\\{cos2x=-\frac{1}{2}}\end{array}\right.$,解得x=$2kπ+π±\frac{π}{3}$(k∈Z).
∴x值的集合为{x|x=$2kπ+π±\frac{π}{3}$(k∈Z)}.
(3)∵向量$\overrightarrow{{a}_{1}}$=(u,v)、$\overrightarrow{{a}_{2}}$=(sinα、sinα)、$\overrightarrow{{a}_{3}}$=(cosα,cosα),
∴$\overrightarrow{{S}_{3}}$=(u+sinα+cosα,v+sinα+cosα),
$|\overrightarrow{{a}_{1}}|$=$\sqrt{{u}^{2}+{v}^{2}}$,$|\overrightarrow{{a}_{2}}|=|\overrightarrow{{a}_{3}}|$=1.
∵向量$\overrightarrow{{a}_{1}}$=(u,v)、$\overrightarrow{{a}_{2}}$=(sinα、sinα)、$\overrightarrow{{a}_{3}}$=(cosα,cosα)中的每一个向量都是它的“等横向量”.
∴$\sqrt{{u}^{2}+{v}^{2}}$=$|\overrightarrow{{S}_{3}}-\overrightarrow{{a}_{1}}|$=$\sqrt{(sinα+cosα)^{2}+(sinα+cosα)^{2}}$=$\sqrt{2+2sin2α}$,化为u2+v2=2+2sin2α,
由$|\overrightarrow{{S}_{3}}-\overrightarrow{{a}_{2}}|$=$\sqrt{(u+cosα)^{2}+(v+cosα)^{2}}$=$\sqrt{2si{n}^{2}α}$,化为u2+v2+2(u+v)cosα+2cos2α=0;
由$|\overrightarrow{{S}_{3}}-\overrightarrow{{a}_{3}}|$=$\sqrt{(u+sinα)^{2}+(v+sinα)^{2}}$=$\sqrt{2co{s}^{2}α}$,化为u2+v2+2(u+v)sinα-2cos2α=0.
化为(cosα+sinα)(u+v)=2+2sin2α=2(cosα+sinα)2,
当cosα+sinα≠0时,u+v=2(cosα+sinα)=2$\sqrt{2}$$sin(α+\frac{π}{4})$∈$[-2\sqrt{2},2\sqrt{2}]$,且不等于0.
当cosα+sinα=0时,$α=\frac{3π}{4}+kπ$(k∈Z),u=v=0.
综上可得:(u+v)∈$[-2\sqrt{2},2\sqrt{2}]$.
点评 本题考查了新定义“等横向量”、向量的坐标运算及其数量积运算性质、三角函数化简计算,考查了推理能力与计算能力,属于难题.

 天天向上一本好卷系列答案
天天向上一本好卷系列答案 小学生10分钟应用题系列答案
小学生10分钟应用题系列答案| A. | x2>6.635 | B. | x2≤6.635 | C. | x2≤3.841 | D. | x2>3.841 |
| A. | 2×($\frac{2}{3}$)n-1 | B. | 2×($\frac{1}{3}$)n-1 | C. | 2×($\frac{4}{3}$)n-1 | D. | 2×($\frac{4}{3}$)n |
| A. | 21 | B. | $\frac{23}{3}$ | C. | $\frac{13}{3}$ | D. | -9 |
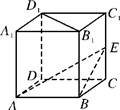 如图,在正方体ABCD-A1B1C1D1中,E为棱CC1的中点.
如图,在正方体ABCD-A1B1C1D1中,E为棱CC1的中点. 如图圆O是半径为1的圆,点PO、P1、P2…、P11将圆12等分,则$\overrightarrow{O{P}_{0}}$$•\overrightarrow{O{P}_{i}}$(i=0,1,2,3,…,11)的取值集合是{-1,-$\frac{1}{2}$,-$\frac{\sqrt{3}}{2}$,0,$\frac{1}{2}$,-$\frac{\sqrt{3}}{2}$,1}.
如图圆O是半径为1的圆,点PO、P1、P2…、P11将圆12等分,则$\overrightarrow{O{P}_{0}}$$•\overrightarrow{O{P}_{i}}$(i=0,1,2,3,…,11)的取值集合是{-1,-$\frac{1}{2}$,-$\frac{\sqrt{3}}{2}$,0,$\frac{1}{2}$,-$\frac{\sqrt{3}}{2}$,1}.