题目内容
在△ABC中,角A,B,C所对的边分别为a,b,c,已知cos C+(cos A-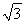 sin A)cos B=0.
sin A)cos B=0.
(1)求角B的大小;
(2)若a+c=1,求b的取值范围.
(1) 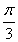 (2)
(2)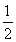 ≤b<1
≤b<1
【解析】(1)由已知得-cos(A+B)+cos Acos B-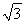 sin Acos B=0,即有sin Asin B-
sin Acos B=0,即有sin Asin B-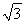 sin Acos B=0,
sin Acos B=0,
因为sin A≠0,所以sin B-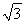 cos B=0,
cos B=0,
即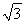 cos B=sin B.所以tan B=
cos B=sin B.所以tan B=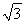 ,
,
又因为0<B<π,所以B=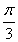 .
.
(2)由余弦定理得b2=a2+c2-2accos B,
因为a+c=1,cos B=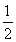 ,
,
所以b2=(a+c)2-3ac≥(a+c)2-3 2=
2=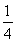 (a+c)2=
(a+c)2=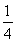 ,∴b≥
,∴b≥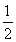 .
.
又a+c>b,∴b<1,∴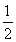 ≤b<1.
≤b<1.

练习册系列答案
 计算高手系列答案
计算高手系列答案
相关题目