题目内容
2010年11月广州成功举办了第十六届亚运会.在华南理工大学学生会举行的亚运知识有奖问答比赛中,甲、乙、丙同时回答一道有关亚运知识的问题,已知甲回答对这道题目的概率是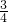 ,甲、丙两人都回答错的概率是
,甲、丙两人都回答错的概率是 ,乙、丙两人都回答对的概率是
,乙、丙两人都回答对的概率是 .
.
(1)求乙、丙两人各自回答对这道题目的概率.
(2)求回答对这道题目的人数的随机变量ξ的分布列和期望.
解:(1)设乙、丙各自回答对的概率分别是p1,p2,
根据题意,得
 ,
,
解得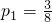 ,
,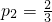 .
.
故乙答对的概率为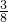 ,丙答对的概率为
,丙答对的概率为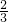 .
.
(2)ξ可能取值0,1,2,3,
P(ξ=0)=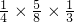 =
=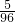 ;
;
P(ξ=1)=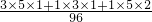 =
=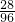 ;
;
P(ξ=2)=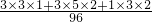 =
=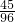 ;
;
P(ξ=3)=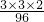 =
=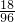 .
.
∴ξ的分布列如下:
数学期望为Eξ= +
+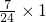 +
+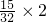 +
+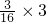 =
=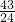 .
.
分析:(1)设乙、丙各自回答对的概率分别是p1,p2,根据题意,得 ,由此能求出乙、丙两人各自回答对这道题目的概率.
,由此能求出乙、丙两人各自回答对这道题目的概率.
(2)ξ可能取值0,1,2,3,分别求出P(ξ=0),P(ξ=1),P(ξ=2),P(ξ=3),由此能求出ξ的分布列和数学期望为Eξ.
点评:本题考查离散型随机变量的分布列和数学期望,是中档题,在历年高考中都是必考题型,解题时要认真审题,仔细解答.
根据题意,得
 ,
,解得
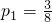 ,
,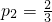 .
.故乙答对的概率为
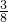 ,丙答对的概率为
,丙答对的概率为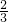 .
.(2)ξ可能取值0,1,2,3,
P(ξ=0)=
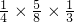 =
=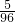 ;
;P(ξ=1)=
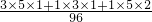 =
=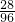 ;
;P(ξ=2)=
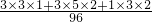 =
=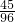 ;
;P(ξ=3)=
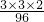 =
=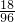 .
.∴ξ的分布列如下:
| ξ | 0 | 1 | 2 | 3 |
| p | 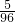 |  | 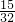 | 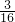 |
 +
+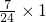 +
+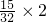 +
+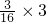 =
=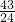 .
.分析:(1)设乙、丙各自回答对的概率分别是p1,p2,根据题意,得
 ,由此能求出乙、丙两人各自回答对这道题目的概率.
,由此能求出乙、丙两人各自回答对这道题目的概率.(2)ξ可能取值0,1,2,3,分别求出P(ξ=0),P(ξ=1),P(ξ=2),P(ξ=3),由此能求出ξ的分布列和数学期望为Eξ.
点评:本题考查离散型随机变量的分布列和数学期望,是中档题,在历年高考中都是必考题型,解题时要认真审题,仔细解答.

练习册系列答案
 小学课时作业全通练案系列答案
小学课时作业全通练案系列答案 金版课堂课时训练系列答案
金版课堂课时训练系列答案 单元全能练考卷系列答案
单元全能练考卷系列答案
相关题目
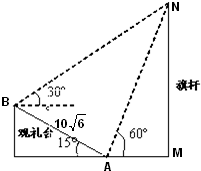 2010年11月12日广州亚运会上举行升旗仪式.如图,在坡度为15°的观礼台上,某一列座位所在直线AB与旗杆所在直线MN共面,在该列的第一个座位A和最后一个座位B测得旗杆顶端N的仰角分别为60°和30°,且座位A、B的距离为
2010年11月12日广州亚运会上举行升旗仪式.如图,在坡度为15°的观礼台上,某一列座位所在直线AB与旗杆所在直线MN共面,在该列的第一个座位A和最后一个座位B测得旗杆顶端N的仰角分别为60°和30°,且座位A、B的距离为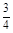 ,甲、丙两人都回答错的概率是
,甲、丙两人都回答错的概率是 ,乙、丙两人都回答对的概率是
,乙、丙两人都回答对的概率是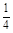 .
.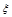 的分布列和期望.
的分布列和期望.