题目内容
(本题满分14分)
已知函数y=sin(2x)-8(sin x+cos x)+19(0≤x≤π),求函数y的最大值与最小值.
【解答】令t=sin x+cos x,则t=![]() sin(x+
sin(x+![]() ), ………4分
), ………4分
∵0≤x≤π,∴![]() ≤x+
≤x+![]() ≤
≤![]() ,
,![]() ≤sin(x+
≤sin(x+![]() )≤1,即-1≤t≤
)≤1,即-1≤t≤![]() .
.
由t=sin x+cos x两边平方得2sin xcos x=t2-1,∴sin 2x=t2-1 ………10分
y= t2-1-8t+19,即f(t)=(t-4)2+2,∵-1≤t≤![]()
∴ymax= f(-1)=27 ymin= f(![]() )=20-8
)=20-8![]() ………14分
………14分

练习册系列答案
相关题目
 B=[0,3],求实数m的值
B=[0,3],求实数m的值 CRB,求实数m的取值范围
CRB,求实数m的取值范围 是⊙
是⊙ :
: 上的任意一点,过
上的任意一点,过 垂直
垂直 轴于
轴于 ,动点
,动点 满足
满足 。
。 ,在动点
,在动点 、
、 ,使
,使 (O是坐标原点),若存在,求出直线
(O是坐标原点),若存在,求出直线 的方程,若不存在,请说明理由。
的方程,若不存在,请说明理由。 .
. 的定义域;
的定义域; 是否有根?如果有根
是否有根?如果有根 ,请求出一个长度为
,请求出一个长度为 的区间
的区间 ,使
,使
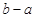 ).
).