题目内容
已知函数![]() .
.
(1)若![]() ,求x的取值范围;
,求x的取值范围;
(2)若![]() 对于
对于![]() ∈[1,2]恒成立,求实数m的取值范围.
∈[1,2]恒成立,求实数m的取值范围.
解:(1)当x<0时,f(x)=0;
当x≥0时,f(x)=2x-![]() .
.
由条件可知2x-![]() 》2,即22x-1.5·2x-1》0,
》2,即22x-1.5·2x-1》0,
解得2x》2
∵2x>0,∴x》1.
(2)当t∈[1,2]时,2t![]() +m
+m![]() ≥0,
≥0,
即m(22t-1)≥-(24t-1).
∵22t-1>0,∴m≥-(22t+1).
∵t∈[1,2],∴-(1+22t)∈[-17,-5],
故m的取值范围是[-5,+∞).

练习册系列答案
 名校课堂系列答案
名校课堂系列答案
相关题目
 ,
,
 ,求
,求 的单调区间;
的单调区间; 时,求证:
时,求证: .
.
 .
. 为
为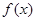 的极值点,求实数
的极值点,求实数 的值;
的值; 在
在 上为增函数,求实数
上为增函数,求实数 时,方程
时,方程 有实根,求实数
有实根,求实数 的最大值.
的最大值. 。
。 ,求函数
,求函数 的值;
的值; .
.
 中任取一个元素
中任取一个元素 ,从集合
,从集合 中任取一个元素
中任取一个元素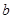 ,求方程
,求方程 有两个不相等实根的概率;
有两个不相等实根的概率; 中任取的一个数,
中任取的一个数, 中任取的一个数,求方程
中任取的一个数,求方程