题目内容
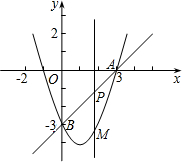
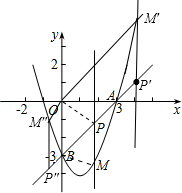
如图,在平面直角坐标系中,抛物线 经过A(3,0),B(0,-3)两点,点P是直线AB上一动点,过点P作
经过A(3,0),B(0,-3)两点,点P是直线AB上一动点,过点P作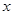 轴的垂线交抛物线于点M,设点P的横坐标为t,
轴的垂线交抛物线于点M,设点P的横坐标为t,【小题1】分别求直线AB和这条抛物线的解析式(4分)
【小题2】若点P在第四象限,连结BM、AM,当线段PM最长时,求
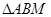 的面积。(4分)
的面积。(4分)③ 【小题3】是否存在这样的点P,使得以点P、M、B、O为顶点的四边形为平行四边形?若存在,请直接写出点P的横坐标;若不存在,请说明理由(3分)。
【小题1】把A(3,0)B(0,﹣3)代入y=x2+mx+n,得
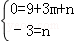
解得
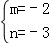 ,
,所以抛物线的解析式是y=x2﹣2x﹣3.
设直线AB的解析式是y=kx+b,
把A(3,0)B(0,﹣3)代入y=kx+b,得
 ,
,解得
 ,
,所以直线AB的解析式是y=x﹣3;(4分)
【小题2】设点P的坐标是(t,t﹣3),则M(t,t2﹣2t﹣3),
因为p在第四象限,
所以PM=(t﹣3)﹣(t2﹣2t﹣3)=﹣t2+3t,
当t=﹣
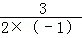 =
=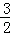 时,二次函数的最大值,即PM最长值为
时,二次函数的最大值,即PM最长值为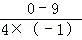 =
=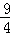 ,
,则S△ABM=S△BPM+S△APM=
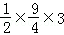 =
=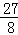 .(4分)
.(4分)【小题3】存在,理由如下:
∵PM∥OB,
∴当PM=OB时,点P、M、B、O为顶点的四边形为平行四边形,
①当P在第四象限:PM=OB=3,PM最长时只有
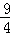 ,所以不可能有PM=3.
,所以不可能有PM=3.②当P在第一象限:PM=OB=3,(t2﹣2t﹣3)﹣(t﹣3)=3,解得t1=
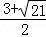 ,t2=
,t2=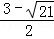 (舍去),所以P点的横坐标是
(舍去),所以P点的横坐标是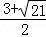 ;
;③当P在第三象限:PM=OB=3,t2﹣3t=3,解得t1=
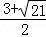 (舍去),t2=
(舍去),t2=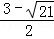 ,所以P点的横坐标是
,所以P点的横坐标是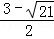 .
.所以P点的横坐标是
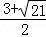 或
或 .
. 解析:
解析:(1)分别利用待定系数法求两函数的解析式:把A(3,0)B(0,﹣3)分别代入y=x2+mx+n与y=kx+b,得到关于m、n的两个方程组,解方程组即可;
(2)设点P的坐标是(t,t﹣3),则M(t,t2﹣2t﹣3),用P点的纵坐标减去M的纵坐标得到PM的长,即PM=(t﹣3)﹣(t2﹣2t﹣3)=﹣t2+3t,然后根据二次函数的最值得到
当t=﹣
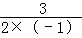 =
=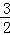 时,PM最长为
时,PM最长为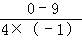 =
=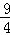 ,再利用三角形的面积公式利用S△ABM=S△BPM+S△APM计算即可;
,再利用三角形的面积公式利用S△ABM=S△BPM+S△APM计算即可;(3)由PM∥OB,根据平行四边形的判定得到当PM=OB时,点P、M、B、O为顶点的四边形为平行四边形,然后讨论:当P在第四象限:PM=OB=3,PM最长时只有
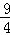 ,所以不可能;当P在第一象限:PM=OB=3,(t2﹣2t﹣3)﹣(t﹣3)=3;当P在第三象限:PM=OB=3,t2﹣3t=3,分别解一元二次方程即可得到满足条件的t的值.
,所以不可能;当P在第一象限:PM=OB=3,(t2﹣2t﹣3)﹣(t﹣3)=3;当P在第三象限:PM=OB=3,t2﹣3t=3,分别解一元二次方程即可得到满足条件的t的值. 
练习册系列答案
相关题目
 如图,在平面直角坐标中,四边形OABC是等腰梯形,CB∥OA,OA=7,AB=4,∠COA=60°,点P为x轴上的一个动点,但是点P不与点0、点A重合.连接CP,D点是线段AB上一点,连接PD.
如图,在平面直角坐标中,四边形OABC是等腰梯形,CB∥OA,OA=7,AB=4,∠COA=60°,点P为x轴上的一个动点,但是点P不与点0、点A重合.连接CP,D点是线段AB上一点,连接PD.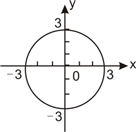 (2012•渝北区一模)如图,在平面直角坐标xoy中,以坐标原点O为圆心,3为半径画圆,从此圆内(包括边界)的所有整数点(横、纵坐标均为整数)中任意选取一个点,其横、纵坐标之和为0的概率是
(2012•渝北区一模)如图,在平面直角坐标xoy中,以坐标原点O为圆心,3为半径画圆,从此圆内(包括边界)的所有整数点(横、纵坐标均为整数)中任意选取一个点,其横、纵坐标之和为0的概率是 如图,在平面直角坐标中,等腰梯形ABCD的下底在x轴上,且B点坐标为(4,0),D点坐标为(0,3),则AC长为
如图,在平面直角坐标中,等腰梯形ABCD的下底在x轴上,且B点坐标为(4,0),D点坐标为(0,3),则AC长为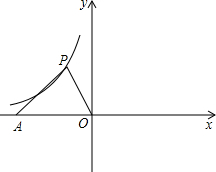 如图,在平面直角坐标xOy中,已知点A(-5,0),P是反比例函数
如图,在平面直角坐标xOy中,已知点A(-5,0),P是反比例函数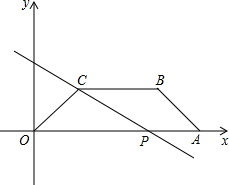 ∠COA=45°,动点P从点O出发,在梯形OABC的边上运动,路径为O→A→B→C,到达点C时停止.作直线CP.
∠COA=45°,动点P从点O出发,在梯形OABC的边上运动,路径为O→A→B→C,到达点C时停止.作直线CP.