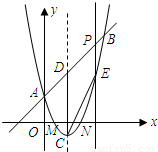
题目内容
如图,⊙M与y轴的正半轴相切于点C,与x轴交于A(x1,0)、B(x2,0)两点,且x2>x1>0,抛物线y= (x2-5x+2m)经过A、B、C三点.
(x2-5x+2m)经过A、B、C三点.(1)求m的值;
(2)求sin∠AMB的值;
(3)在图中的曲线上是否存在点P,使以P、A、C为顶点的三角形与△COA相似?若存在,求出所有符合条件的点P的坐标;若不存在,请说明理由.
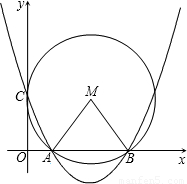
【答案】分析:(1)过点M作x轴的垂线,垂足为点D,在直角三角形AMD中用勾股定理计算求出m的值.
(2)利用(1)中求出的m的值,得到点A,B,M的坐标,求出线段AB,MD,AM的长,然后在△ABM中,用面积法求出sin∠AMB的值.
(3)分别过A,C两点作AC的垂线,与抛物线交于点P1和点P2,因为△AOC中,OA=1,OC=2,所以当△PAC中,满足两直角边的比是1:2时,点P就存在,否则,就不存在.
解答: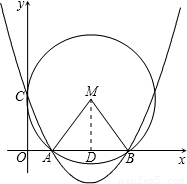 解:(1)如图:过点M作MD⊥AB于点D,
解:(1)如图:过点M作MD⊥AB于点D,
当x=0时,y=m,∴C(0,m)
当y=0时,有 x2-
x2- x+m=0
x+m=0
∴x1+x2=5,x1x2=2m,
AD= AB=
AB= (x2-x1)=
(x2-x1)=

=
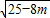 .
.
∵⊙M与y轴相切于点C,
∵AB=0B-OA=x2-x1,
∴OD=AD+OA= AB+OA=
AB+OA=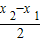 +x1=
+x1= (x1+x2),
(x1+x2),
∴CM=AM=OD= (x1+x2)=
(x1+x2)= .
.
DM=OC=m,
在直角三角形AMD中,
AM2=AD2+MD2,
即: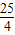 =
= +m2,
+m2,
解得:m1=0,m2=2.
∵m>0,
∴m=2.
(2)∵m=2,
∴y= x2-
x2- x+2
x+2
∴C(0,2)
当y=0时, x2-
x2- x+2=0
x+2=0
解得:x1=1,x2=4,
∴A(1,0),B(4,0),
∴AB=3,AD= ,AM=
,AM= ,MD=2
,MD=2
∵S△ABM= AB•MD=
AB•MD= AM•BM•sin∠AMB,
AM•BM•sin∠AMB,
∴ ×3×2=
×3×2= ×
× ×
× ×sin∠AMB,
×sin∠AMB,
∴sin∠AMB=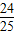 .
.
(3)如图: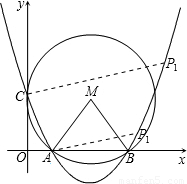
分别过点A,C作AC的垂线交抛物线于P1和P2,
∵A(1,0),C(0,2),AC=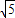
∴AC:y=-2x+2
AP1:y= x-
x- ,
,
AP2:y= x+2,
x+2,
由 得:p1(5,2),AP1=2
得:p1(5,2),AP1=2 ,
,
∵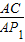 =
=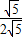 =
= =
=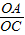 ,
,
∴△P1AC∽△COA.
由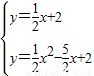 得:P2(6,5),CP2=3
得:P2(6,5),CP2=3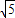 ,
,
∵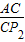 =
= =
= ≠
≠ ,
,
∴△P2AC与△AOC不相似.
因此,存在点P(5,2).
点评:本题考查的是二次函数的综合题,(1)根据二次函数的性质,利用勾股定理求出m的值.(2)用面积法求出角的正弦值.(3)根据相似三角形的性质求出点P的坐标.
(2)利用(1)中求出的m的值,得到点A,B,M的坐标,求出线段AB,MD,AM的长,然后在△ABM中,用面积法求出sin∠AMB的值.
(3)分别过A,C两点作AC的垂线,与抛物线交于点P1和点P2,因为△AOC中,OA=1,OC=2,所以当△PAC中,满足两直角边的比是1:2时,点P就存在,否则,就不存在.
解答:
 解:(1)如图:过点M作MD⊥AB于点D,
解:(1)如图:过点M作MD⊥AB于点D,当x=0时,y=m,∴C(0,m)
当y=0时,有
 x2-
x2- x+m=0
x+m=0∴x1+x2=5,x1x2=2m,
AD=
 AB=
AB= (x2-x1)=
(x2-x1)=

=

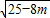 .
.∵⊙M与y轴相切于点C,
∵AB=0B-OA=x2-x1,
∴OD=AD+OA=
 AB+OA=
AB+OA=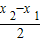 +x1=
+x1= (x1+x2),
(x1+x2),∴CM=AM=OD=
 (x1+x2)=
(x1+x2)= .
.DM=OC=m,
在直角三角形AMD中,
AM2=AD2+MD2,
即:
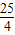 =
= +m2,
+m2,解得:m1=0,m2=2.
∵m>0,
∴m=2.
(2)∵m=2,
∴y=
 x2-
x2- x+2
x+2∴C(0,2)
当y=0时,
 x2-
x2- x+2=0
x+2=0解得:x1=1,x2=4,
∴A(1,0),B(4,0),
∴AB=3,AD=
 ,AM=
,AM= ,MD=2
,MD=2∵S△ABM=
 AB•MD=
AB•MD= AM•BM•sin∠AMB,
AM•BM•sin∠AMB,∴
 ×3×2=
×3×2= ×
× ×
× ×sin∠AMB,
×sin∠AMB,∴sin∠AMB=
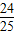 .
.(3)如图:

分别过点A,C作AC的垂线交抛物线于P1和P2,
∵A(1,0),C(0,2),AC=
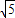
∴AC:y=-2x+2
AP1:y=
 x-
x- ,
,AP2:y=
 x+2,
x+2,由
 得:p1(5,2),AP1=2
得:p1(5,2),AP1=2 ,
,∵
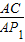 =
=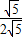 =
= =
=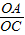 ,
,∴△P1AC∽△COA.
由
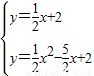 得:P2(6,5),CP2=3
得:P2(6,5),CP2=3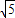 ,
,∵
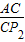 =
= =
= ≠
≠ ,
,∴△P2AC与△AOC不相似.
因此,存在点P(5,2).
点评:本题考查的是二次函数的综合题,(1)根据二次函数的性质,利用勾股定理求出m的值.(2)用面积法求出角的正弦值.(3)根据相似三角形的性质求出点P的坐标.

练习册系列答案
相关题目
 且x2>x1>0,抛物线y=
且x2>x1>0,抛物线y=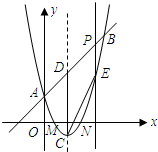 作x轴的垂线交抛物线于点E,使四边形DCEP为平行四边形?若存在,请求出该平行四边形的面积;若不存在,说明理由.
作x轴的垂线交抛物线于点E,使四边形DCEP为平行四边形?若存在,请求出该平行四边形的面积;若不存在,说明理由.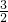 (m+1).
(m+1).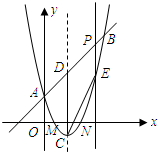 作x轴的垂线交抛物线于点E,使四边形DCEP为平行四边形?若存在,请求出该平行四边形的面积;若不存在,说明理由.
作x轴的垂线交抛物线于点E,使四边形DCEP为平行四边形?若存在,请求出该平行四边形的面积;若不存在,说明理由.
 (m+1).
(m+1).