题目内容
已知下列四个式子
①sin21+cos21
②(lg2)2+lg2•lg5+lg5
③tan15°+tan30°+tan15°tan30°
④sin40°(
-tan10°)
化简结果等于1的式子的代号分别是
①sin21+cos21
②(lg2)2+lg2•lg5+lg5
③tan15°+tan30°+tan15°tan30°
④sin40°(
| 3 |
化简结果等于1的式子的代号分别是
①②③④
①②③④
.分析:由平方关系化简①;由对数运算性质化简②;由两角和的正切公式得:tan15°+tan30°=tan(15°+30°)(1-tan15°tan30°),再代入式子化简③;根据切化弦、两角和的余弦公式、倍角的正弦公式和诱导公式化简④.
解答:解:①、由平方关系得sin21+cos21=1;
②、(lg2)2+lg2•lg5+lg5=lg2(lg2+lg5)+lg5=lg2+lg5=1;
③、由两角和的正切公式得,
tan15°+tan30°=tan(15°+30°)(1-tan15°tan30°)
∴tan15°+tan30°+tan15°tan30°
=tan45°(1-tan15°tan30°)+tan15°tan30°=1;
④、sin40°(
-tan10°)=sin40°(
-
)
=sin40°×
=sin40°×
=sin40°×
=
=1
综上得:①②③④,
故答案为:①②③④.
②、(lg2)2+lg2•lg5+lg5=lg2(lg2+lg5)+lg5=lg2+lg5=1;
③、由两角和的正切公式得,
tan15°+tan30°=tan(15°+30°)(1-tan15°tan30°)
∴tan15°+tan30°+tan15°tan30°
=tan45°(1-tan15°tan30°)+tan15°tan30°=1;
④、sin40°(
| 3 |
| 3 |
| sin10° |
| cos10° |
=sin40°×
| ||
| cos10° |
=sin40°×
2(
| ||||||
| cos10° |
=sin40°×
| 2cos40° |
| cos10° |
| sin80° |
| cos10° |
综上得:①②③④,
故答案为:①②③④.
点评:本题考查了对数运算性质,以及三角恒等变换中的公式和方法,如切化弦、公式的变形等,关键是熟练掌握公式并会灵活应用.

练习册系列答案
 新思维假期作业暑假吉林大学出版社系列答案
新思维假期作业暑假吉林大学出版社系列答案 蓝天教育暑假优化学习系列答案
蓝天教育暑假优化学习系列答案
相关题目
 (2011•静海县一模)已知下列四个命题:
(2011•静海县一模)已知下列四个命题: ;
; <0;
<0; ;
; 的角θ取值范围是(
的角θ取值范围是( +2kπ,
+2kπ, +2kπ),(k∈Z)
+2kπ),(k∈Z)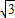 -tan10°)
-tan10°)