题目内容
已知二次函数y=f(x)在x= 处取得最小值-
处取得最小值- (t≠0)且f(1)=0.
(t≠0)且f(1)=0.
(1)求y=f(x)的表达式;
(2)若函数y=f(x)在区间[-1, ]上的最小值为-5,求此时t的值.
]上的最小值为-5,求此时t的值.
解:(1)设f(x)=a(x- )2-
)2- (a>0).
(a>0).
因为f(1)=0,所以(a-1) =0.
=0.
又t≠0,所以a=1,
所以f(x)=(x- )2-
)2- (t≠0).
(t≠0).
(2)因为f(x)=(x- )2-
)2- (t≠0),
(t≠0),
①当 <-1,即t<-4时,
<-1,即t<-4时,
f(x)min=f(-1)=(-1- )2-
)2- =-5,解得t=-
=-5,解得t=-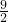 ;
;
②当-1≤ ≤
≤ ,即-4≤t≤-1时,
,即-4≤t≤-1时,
f(x)min=f( )=-
)=- =-5,解得t=±2
=-5,解得t=±2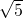 (舍去);
(舍去);
③当 >
> ,即t>-1时,
,即t>-1时,
f(x)min=f( )=(
)=( -
- )2-
)2-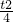 =-5,解得t=-
=-5,解得t=-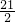 (舍去).
(舍去).
综上得,所求的t=-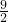 .
.
分析:(1)(1)根据条件可设二次函数的顶点式f(x)=a(x- )2-
)2- ,由f(1)=0,可得a,从而求得f(x)表达式.
,由f(1)=0,可得a,从而求得f(x)表达式.
(2)根据对称轴与区间的位置关系分三种情况进行讨论,求出其最小值,令其等于-5,即可求得t值.
点评:本题考查二次函数在闭区间上最值问题及二次函数解析式的求解,考查分类讨论思想、数形结合思想,属中档题.
 )2-
)2- (a>0).
(a>0).因为f(1)=0,所以(a-1)
 =0.
=0.又t≠0,所以a=1,
所以f(x)=(x-
 )2-
)2- (t≠0).
(t≠0).(2)因为f(x)=(x-
 )2-
)2- (t≠0),
(t≠0),①当
 <-1,即t<-4时,
<-1,即t<-4时,f(x)min=f(-1)=(-1-
 )2-
)2- =-5,解得t=-
=-5,解得t=-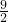 ;
;②当-1≤
 ≤
≤ ,即-4≤t≤-1时,
,即-4≤t≤-1时,f(x)min=f(
 )=-
)=- =-5,解得t=±2
=-5,解得t=±2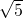 (舍去);
(舍去);③当
 >
> ,即t>-1时,
,即t>-1时,f(x)min=f(
 )=(
)=( -
- )2-
)2-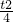 =-5,解得t=-
=-5,解得t=-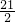 (舍去).
(舍去).综上得,所求的t=-
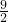 .
.分析:(1)(1)根据条件可设二次函数的顶点式f(x)=a(x-
 )2-
)2- ,由f(1)=0,可得a,从而求得f(x)表达式.
,由f(1)=0,可得a,从而求得f(x)表达式.(2)根据对称轴与区间的位置关系分三种情况进行讨论,求出其最小值,令其等于-5,即可求得t值.
点评:本题考查二次函数在闭区间上最值问题及二次函数解析式的求解,考查分类讨论思想、数形结合思想,属中档题.

练习册系列答案
相关题目
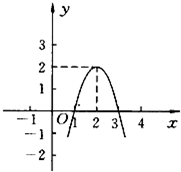 已知二次函数y=f(x)的图象如图所示:
已知二次函数y=f(x)的图象如图所示: