题目内容
设函数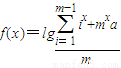
，其中a∈R，m是给定的正整数，且m≥2，如果不等式f（x）＞（x-1）lgm在区间[1，+∞）有解，则实数a的取值范围是 ．
【答案】分析：依据题意利用函数解析式，根据题设不等式求得1-a＜（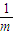
）x+（
）x+…+（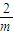
）x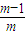=g（x）．根据m的范围，判断出g（x）在[1，+∞）上单调递减．，进而求得函数g（x）的最大值，利用g（x）max＞1-a求得a范围．
解答：解：f（x）=lg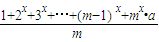
＞（x-1）lgm=lgmx-1，
∴
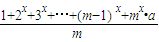＞mx-1．
∴1-a＜（
）x+（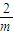
）x+…+（
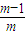）x=g（x）．
∵
，
，…，
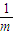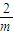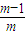∈（0，1），
∴g（x）在[1，+∞）上单调递减．
∴g（x）max=f（1）=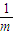
+
+…+
=
．
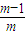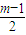由题意知，1-a＜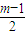
，
∴a＞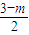
．又m是给定的正整数，且m≥2，故a＞
故答案为：a＞
．
点评：本题主要考查了函数的单调性的性质．考查了学生对函数基础知识的掌握程度．
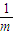）x+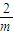（）x+…+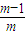（）x=g（x）．根据m的范围，判断出g（x）在[1，+∞）上单调递减．，进而求得函数g（x）的最大值，利用g（x）max＞1-a求得a范围．解答：解：f（x）=lg
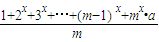＞（x-1）lgm=lgmx-1，∴
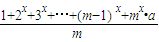＞mx-1．∴1-a＜（
）x+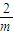（）x+…+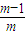（）x=g（x）．∵
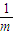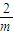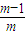，，…，∈（0，1），∴g（x）在[1，+∞）上单调递减．
∴g（x）max=f（1）=
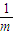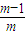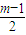++…+=．由题意知，1-a＜
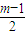，∴a＞
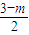．又m是给定的正整数，且m≥2，故a＞故答案为：a＞
．点评：本题主要考查了函数的单调性的性质．考查了学生对函数基础知识的掌握程度．
练习册系列答案
海淀课时新作业金榜卷系列答案
期末金牌卷系列答案
轻松课堂标准练系列答案
相关题目
，其中a∈R，m是给定的正整数，且m≥2．如果不等式f（x）＞（x-1）lgm在区间[1，+∞）上有解，则实数a的取值范围是 ．
，其中a∈R，m是给定的正整数，且m≥2，如果不等式f（x）＞（x-1）lgm在区间[1，+∞）有解，则实数a的取值范围是 ．
，其中a∈R，m是给定的正整数，且m≥2，如果不等式f（x）＞（x-1）lgm在区间[1，+∞）有解，则实数a的取值范围是 ．
，其中a∈R，m是给定的正整数，且m≥2，如果不等式f（x）＜（x-2）lgm在区间[1，+∞）上恒成立，则实数a的取值范围是 ．