题目内容
已知向量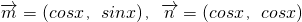 ,设函数
,设函数
(I)求f(x)的解析式,并求最小正周期;
(II)若函数g(x)的图象是由函数f(x)的图象向右平移 个单位得到的,求g(x)的最大值及使g(x)取得最大值时x的值.
个单位得到的,求g(x)的最大值及使g(x)取得最大值时x的值.
解:(I)∵向量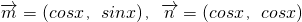 ,
,
∴函数 =cos2x+sinxcosx=
=cos2x+sinxcosx= (1+cos2x)+
(1+cos2x)+ sin2x=
sin2x= sin(2x+
sin(2x+ )+
)+
即f(x)的解析式为y= sin(2x+
sin(2x+ )+
)+ ,最小正周期为T=
,最小正周期为T=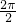 =π;
=π;
(II)将f(x)的图象向右平移 个单位,得到y=f(x-
个单位,得到y=f(x- )=
)= sin[2(x-
sin[2(x- )+
)+ ]+
]+ ,
,
即y= sin2x+
sin2x+ 的图象,因此g(x)=
的图象,因此g(x)= sin2x+
sin2x+
令2x= +2kπ(k∈Z),得x=
+2kπ(k∈Z),得x= +kπ(k∈Z)
+kπ(k∈Z)
∴当x= +kπ(k∈Z),g(x)=
+kπ(k∈Z),g(x)= sin2x+
sin2x+ 取得最大值
取得最大值 +
+
即[g(x)]max= +
+ ,相应的x=
,相应的x= +kπ(k∈Z)
+kπ(k∈Z)
分析:(I)根据向量数量积的坐标运算公式,结合三角恒等变换公式化简,得数f(x)= sin(2x+
sin(2x+ )+
)+ ,再由三角函数的周期公式即可算出求最小正周期T;
,再由三角函数的周期公式即可算出求最小正周期T;
(II)根据函数图象平移的公式,可得g(x)=f(x- )=
)= sin2x+
sin2x+ ,结合正弦函数的图象与性质,可得当x=
,结合正弦函数的图象与性质,可得当x= +kπ(k∈Z),g(x)=
+kπ(k∈Z),g(x)= sin2x+
sin2x+ 取得最大值
取得最大值 +
+ ,得到本题的答案.
,得到本题的答案.
点评:本题以向量的数量积运算为载体,求函数y=Asin(ωx+φ)+k的图象与性质.着重考查了平面向量数量积的坐标公式和三角函数的图象与性质等知识,属于基础题.
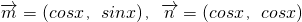 ,
,∴函数
 =cos2x+sinxcosx=
=cos2x+sinxcosx= (1+cos2x)+
(1+cos2x)+ sin2x=
sin2x= sin(2x+
sin(2x+ )+
)+
即f(x)的解析式为y=
 sin(2x+
sin(2x+ )+
)+ ,最小正周期为T=
,最小正周期为T=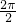 =π;
=π;(II)将f(x)的图象向右平移
 个单位,得到y=f(x-
个单位,得到y=f(x- )=
)= sin[2(x-
sin[2(x- )+
)+ ]+
]+ ,
,即y=
 sin2x+
sin2x+ 的图象,因此g(x)=
的图象,因此g(x)= sin2x+
sin2x+
令2x=
 +2kπ(k∈Z),得x=
+2kπ(k∈Z),得x= +kπ(k∈Z)
+kπ(k∈Z)∴当x=
 +kπ(k∈Z),g(x)=
+kπ(k∈Z),g(x)= sin2x+
sin2x+ 取得最大值
取得最大值 +
+
即[g(x)]max=
 +
+ ,相应的x=
,相应的x= +kπ(k∈Z)
+kπ(k∈Z)分析:(I)根据向量数量积的坐标运算公式,结合三角恒等变换公式化简,得数f(x)=
 sin(2x+
sin(2x+ )+
)+ ,再由三角函数的周期公式即可算出求最小正周期T;
,再由三角函数的周期公式即可算出求最小正周期T;(II)根据函数图象平移的公式,可得g(x)=f(x-
 )=
)= sin2x+
sin2x+ ,结合正弦函数的图象与性质,可得当x=
,结合正弦函数的图象与性质,可得当x= +kπ(k∈Z),g(x)=
+kπ(k∈Z),g(x)= sin2x+
sin2x+ 取得最大值
取得最大值 +
+ ,得到本题的答案.
,得到本题的答案.点评:本题以向量的数量积运算为载体,求函数y=Asin(ωx+φ)+k的图象与性质.着重考查了平面向量数量积的坐标公式和三角函数的图象与性质等知识,属于基础题.

练习册系列答案
相关题目
 ,设函数
,设函数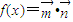
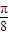 个单位得到的,求g(x)的最大值及使g(x)取得最大值时x的值.
个单位得到的,求g(x)的最大值及使g(x)取得最大值时x的值. ,设函数
,设函数 且f(x)的最小正周期为2π.
且f(x)的最小正周期为2π. ,求证:f(x)>g(x).
,求证:f(x)>g(x). ,设函数
,设函数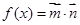
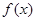 的解析式,并求最小正周期;
的解析式,并求最小正周期;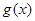 的图像是由函数
的图像是由函数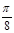 个单位得到的,求
个单位得到的,求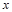 的值.
的值.