题目内容
已知定义在R上的函数f(x)= (sinωx+acosωx)(a∈R,0<ω≤1)满足:f(x)=f(
(sinωx+acosωx)(a∈R,0<ω≤1)满足:f(x)=f( -x),f(x-π)=f(x+π).
-x),f(x-π)=f(x+π).
(I)求f(x)的解析式;
(II)若m2-4n>0,m,n∈R,求证:“|m|+|n|<1”是“方程[f(x)]2+mf(x)+n=0在区间(- ,
, )内有两个不等的实根”的充分不必要条件.
)内有两个不等的实根”的充分不必要条件.
解:(I)∵f(x)= (sinωx+acosωx)=
(sinωx+acosωx)=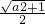 sin(ωx+?),其中sin?=
sin(ωx+?),其中sin?=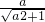 ,cos?=
,cos?=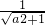 ,
,
由f(x-π)=f(x+π)知f(x)=f(x+2π),即函数f(x)的周期为2π.
∴ ≤2π,即|ω|≥1.又0<ω≤1,∴ω=1.
≤2π,即|ω|≥1.又0<ω≤1,∴ω=1.
又∵f(x)=f( -x),∴f(0)=f(
-x),∴f(0)=f( ),
),
即 (sin0+acos0)=
(sin0+acos0)= (sin
(sin +acos
+acos ),解得 a=
),解得 a= ,∴f(x)=sin(x+
,∴f(x)=sin(x+ ).
).
(II)显然,x∈(- ,
, )等价于x+
)等价于x+ ∈(-
∈(- ,
, ).
).
令u=x+ ,f(x)=t,g(t)=t2+mt+n,则f(x)=sinu,
,f(x)=t,g(t)=t2+mt+n,则f(x)=sinu,
由|m|+|n|<1得|m+n|≤|m|+|n|<1,∴m+n>-1.
同理由|m-n|≤|m|+|n|<1得m-n<1.
∴g(1)=m+n+1>0,g(-1)=1-m+n>0.
又∵|m|≤|m|+|n|<1,∴-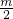 ∈(-1,1).
∈(-1,1).
又∵△=m2-4n>0,∴一元二次方程t2+mt+n=0在区间(-1,1)内有两个不等的实根.
∵函数y=sinu(u∈(- ,
, ))与u=x+
))与u=x+ (x∈(-
(x∈(- ,
, ))都是增函数,
))都是增函数,
∴[f(x)]2+mf(x)+n=0在区间(- ,
, )内有两个不等实根.
)内有两个不等实根.
∴“|m|+|n|<1”是“方程[f(x)]2+mf(x)+n=0在区间(- ,
, )内有两个不等实根”的充分条件.
)内有两个不等实根”的充分条件.
令m=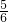 ,n=
,n=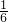 ,由于方程t2+
,由于方程t2+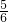 t+
t+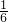 =0有两个不等的实根-
=0有两个不等的实根-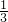 ,-
,- ,且-
,且-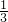 ,-
,- ∈(-1,1),
∈(-1,1),
∴方程sin2(x+ )+
)+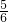 sin(x+
sin(x+ )+
)+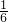 =0在(-
=0在(- ,
, )内有两个不等的实根,
)内有两个不等的实根,
但|m|+|n|=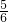 +
+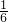 =1,
=1,
故“|m|+|n|<1”不是“方程[f(x)]2+mf(x)+n=0在区间(- ,
, )内有两个不等实根”的必要条件.
)内有两个不等实根”的必要条件.
综上,“|m|+|n|<1”是“方程[f(x)]2+mf(x)+n=0在区间(- ,
, )内有两个不等实根”的充分不必要条件.
)内有两个不等实根”的充分不必要条件.
分析:(I)利用辅助角公式化简函数的表达式为Asin(ωx+?),利用f(x-π)=f(x+π),求出函数的周期,通过周期公式求出ω,通过f(x)=f( -x),令x=0,得到f(0)=f(
-x),令x=0,得到f(0)=f( ),求出a,即可求f(x)的解析式;
),求出a,即可求f(x)的解析式;
(II)通过x∈(- ,
, ),求出x+
),求出x+ ∈(-
∈(- ,
, ).令u=x+
).令u=x+ ,f(x)=t,g(t)=t2+mt+n,则f(x)=sinu,
,f(x)=t,g(t)=t2+mt+n,则f(x)=sinu,
由|m|+|n|<1推出-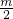 ∈(-1,1).利用△>0,说明一元二次方程t2+mt+n=0在区间(-1,1)内有两个不等的实根.
∈(-1,1).利用△>0,说明一元二次方程t2+mt+n=0在区间(-1,1)内有两个不等的实根.
结合函数y=sinu(u∈(- ,
, ))与u=x+
))与u=x+ (x∈(-
(x∈(- ,
, ))都是增函数,推出所证的充分条件.
))都是增函数,推出所证的充分条件.
通过m=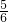 ,n=
,n=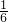 ,说明“|m|+|n|<1”不是“方程[f(x)]2+mf(x)+n=0在区间(-
,说明“|m|+|n|<1”不是“方程[f(x)]2+mf(x)+n=0在区间(- ,
, )内有两个不等实根”的必要条件.
)内有两个不等实根”的必要条件.
点评:本题考查三角函数的化简,解析式的求法,以及充分条件与必要条件的证明,方程的根的知识,考查转化思想,计算能力,反例证明问题的应用.
 (sinωx+acosωx)=
(sinωx+acosωx)=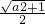 sin(ωx+?),其中sin?=
sin(ωx+?),其中sin?=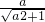 ,cos?=
,cos?=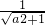 ,
,由f(x-π)=f(x+π)知f(x)=f(x+2π),即函数f(x)的周期为2π.
∴
 ≤2π,即|ω|≥1.又0<ω≤1,∴ω=1.
≤2π,即|ω|≥1.又0<ω≤1,∴ω=1.又∵f(x)=f(
 -x),∴f(0)=f(
-x),∴f(0)=f( ),
),即
 (sin0+acos0)=
(sin0+acos0)= (sin
(sin +acos
+acos ),解得 a=
),解得 a= ,∴f(x)=sin(x+
,∴f(x)=sin(x+ ).
).(II)显然,x∈(-
 ,
, )等价于x+
)等价于x+ ∈(-
∈(- ,
, ).
).令u=x+
 ,f(x)=t,g(t)=t2+mt+n,则f(x)=sinu,
,f(x)=t,g(t)=t2+mt+n,则f(x)=sinu,由|m|+|n|<1得|m+n|≤|m|+|n|<1,∴m+n>-1.
同理由|m-n|≤|m|+|n|<1得m-n<1.
∴g(1)=m+n+1>0,g(-1)=1-m+n>0.
又∵|m|≤|m|+|n|<1,∴-
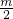 ∈(-1,1).
∈(-1,1).又∵△=m2-4n>0,∴一元二次方程t2+mt+n=0在区间(-1,1)内有两个不等的实根.
∵函数y=sinu(u∈(-
 ,
, ))与u=x+
))与u=x+ (x∈(-
(x∈(- ,
, ))都是增函数,
))都是增函数,∴[f(x)]2+mf(x)+n=0在区间(-
 ,
, )内有两个不等实根.
)内有两个不等实根.∴“|m|+|n|<1”是“方程[f(x)]2+mf(x)+n=0在区间(-
 ,
, )内有两个不等实根”的充分条件.
)内有两个不等实根”的充分条件.令m=
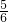 ,n=
,n=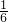 ,由于方程t2+
,由于方程t2+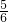 t+
t+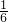 =0有两个不等的实根-
=0有两个不等的实根-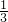 ,-
,- ,且-
,且-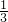 ,-
,- ∈(-1,1),
∈(-1,1),∴方程sin2(x+
 )+
)+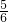 sin(x+
sin(x+ )+
)+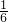 =0在(-
=0在(- ,
, )内有两个不等的实根,
)内有两个不等的实根,但|m|+|n|=
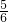 +
+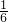 =1,
=1,故“|m|+|n|<1”不是“方程[f(x)]2+mf(x)+n=0在区间(-
 ,
, )内有两个不等实根”的必要条件.
)内有两个不等实根”的必要条件.综上,“|m|+|n|<1”是“方程[f(x)]2+mf(x)+n=0在区间(-
 ,
, )内有两个不等实根”的充分不必要条件.
)内有两个不等实根”的充分不必要条件.分析:(I)利用辅助角公式化简函数的表达式为Asin(ωx+?),利用f(x-π)=f(x+π),求出函数的周期,通过周期公式求出ω,通过f(x)=f(
 -x),令x=0,得到f(0)=f(
-x),令x=0,得到f(0)=f( ),求出a,即可求f(x)的解析式;
),求出a,即可求f(x)的解析式;(II)通过x∈(-
 ,
, ),求出x+
),求出x+ ∈(-
∈(- ,
, ).令u=x+
).令u=x+ ,f(x)=t,g(t)=t2+mt+n,则f(x)=sinu,
,f(x)=t,g(t)=t2+mt+n,则f(x)=sinu,由|m|+|n|<1推出-
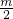 ∈(-1,1).利用△>0,说明一元二次方程t2+mt+n=0在区间(-1,1)内有两个不等的实根.
∈(-1,1).利用△>0,说明一元二次方程t2+mt+n=0在区间(-1,1)内有两个不等的实根.结合函数y=sinu(u∈(-
 ,
, ))与u=x+
))与u=x+ (x∈(-
(x∈(- ,
, ))都是增函数,推出所证的充分条件.
))都是增函数,推出所证的充分条件.通过m=
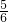 ,n=
,n=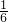 ,说明“|m|+|n|<1”不是“方程[f(x)]2+mf(x)+n=0在区间(-
,说明“|m|+|n|<1”不是“方程[f(x)]2+mf(x)+n=0在区间(- ,
, )内有两个不等实根”的必要条件.
)内有两个不等实根”的必要条件.点评:本题考查三角函数的化简,解析式的求法,以及充分条件与必要条件的证明,方程的根的知识,考查转化思想,计算能力,反例证明问题的应用.

练习册系列答案
 阅读快车系列答案
阅读快车系列答案
相关题目
已知定义在R上的函数f(x),对任意x∈R,都有f(x+6)=f(x)+f(3)成立,若函数y=f(x+1)的图象关于直线x=-1对称,则f(2013)=( )
| A、0 | B、2013 | C、3 | D、-2013 |