题目内容
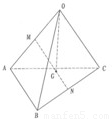
 已知如图,空间四边形OABC,其对角线为OB、AC,M、N分别是对边OA、BC的中点,点G在线段MN上,且使MG=2GN,
已知如图,空间四边形OABC,其对角线为OB、AC,M、N分别是对边OA、BC的中点,点G在线段MN上,且使MG=2GN,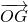 =x
=x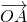 +y
+y +z
+z ,则x+y+z=
,则x+y+z=
- A.
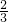
- B.
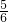
- C.1
- D.

B
分析:以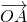 ,
, ,
, 为一组基向量,首先
为一组基向量,首先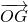 =
=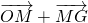 ,再将
,再将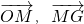 逐步地用基向量表示,最后合并整理得出结果.
逐步地用基向量表示,最后合并整理得出结果.
解答: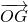 =
=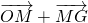 =
=
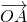 +
+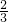
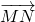 =
=
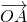 +
+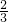 (
(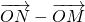 )
)
=
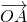 +
+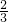 [
[ (
(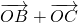 )-
)-
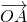 ]
]
=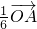 +
+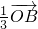 +
+
∴x+y+z=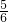
故选B.
点评:本题考查空间向量基本定理,关键是灵活准确的将不是基底中的向量的情况,转化为基向量.
分析:以
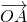 ,
, ,
, 为一组基向量,首先
为一组基向量,首先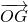 =
=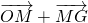 ,再将
,再将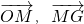 逐步地用基向量表示,最后合并整理得出结果.
逐步地用基向量表示,最后合并整理得出结果.解答:
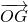 =
=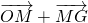 =
=
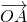 +
+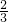
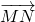 =
=
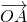 +
+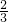 (
(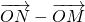 )
)=

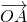 +
+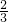 [
[ (
(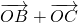 )-
)-
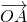 ]
]=
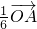 +
+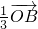 +
+
∴x+y+z=
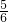
故选B.
点评:本题考查空间向量基本定理,关键是灵活准确的将不是基底中的向量的情况,转化为基向量.

练习册系列答案
 云南师大附小一线名师提优作业系列答案
云南师大附小一线名师提优作业系列答案 冲刺100分单元优化练考卷系列答案
冲刺100分单元优化练考卷系列答案
相关题目
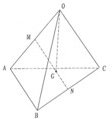
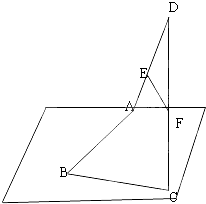 已知:如图,空间四边形ABCD中,E、F分别是DA、DC的中点.求证:EF∥平面ABC.
已知:如图,空间四边形ABCD中,E、F分别是DA、DC的中点.求证:EF∥平面ABC. 已知如图,空间四边形OABC,其对角线为OB、AC,M、N分别是对边OA、BC的中点,点G在线段MN上,且使MG=2GN,
已知如图,空间四边形OABC,其对角线为OB、AC,M、N分别是对边OA、BC的中点,点G在线段MN上,且使MG=2GN, =x
=x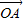 +y
+y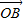 +z
+z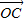 ,则x+y+z=( )
,则x+y+z=( )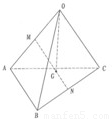



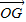 =x
=x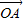 +y
+y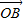 +z
+z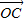 ,则x+y+z=( )
,则x+y+z=( )