题目内容
13.已知点G是△ABC的重心,∠BAC=120°,$\overrightarrow{AB}$•$\overrightarrow{CA}$=2,则|$\overrightarrow{AB}$$+\overrightarrow{AG}$$+\overrightarrow{AC}$|的最小值为$\frac{8}{3}$.分析 根据G为重心便可得到$\overrightarrow{AG}=\frac{1}{3}(\overrightarrow{AB}+\overrightarrow{AC})$,并根据条件可以得到AB•AC=4,本题是求最小值,可以看出需根据不等式求:$≥\frac{16}{9}(2AB•AC-4)$,求$|\overrightarrow{AB}+\overrightarrow{AG}+\overrightarrow{AC}{|}^{2}$=$\frac{16}{9}(A{B}^{2}+A{C}^{2}-4)$,这样即可求出$|\overrightarrow{AB}+\overrightarrow{AG}+\overrightarrow{AC}|$的最小值.
解答  解:如图,G是△ABC的重心;
解:如图,G是△ABC的重心;
∴$\overrightarrow{AG}=\frac{1}{3}(\overrightarrow{AB}+\overrightarrow{AC})$;
由条件得,AB•CA=4;
∴$|\overrightarrow{AB}+\overrightarrow{AG}+\overrightarrow{AC}{|}^{2}$=$|\overrightarrow{AB}+\overrightarrow{AC}+\frac{1}{3}(\overrightarrow{AB}+\overrightarrow{AC}){|}^{2}$=$\frac{16}{9}(\overrightarrow{AB}+\overrightarrow{AC})^{2}$=$\frac{16}{9}(A{B}^{2}+2\overrightarrow{AB}•\overrightarrow{AC}+A{C}^{2})$
=$\frac{16}{9}(A{B}^{2}+A{C}^{2}-4)$$≥\frac{16}{9}(2AB•AC-4)=\frac{16}{9}×4$,当AB=AC时取“=”;
∴$|\overrightarrow{AB}+\overrightarrow{AG}+\overrightarrow{AC}|≥\frac{8}{3}$;
即$|\overrightarrow{AB}+\overrightarrow{AG}+\overrightarrow{AC}|$的最小值为$\frac{8}{3}$.
故答案为:$\frac{8}{3}$.
点评 考查三角形重心的概念及性质,向量加法的平行四边形法则,向量数乘的几何意义,以及向量的加法和数乘运算,在直接求向量长度的最值不好求时,可以考虑求向量平方的最小值,以及不等式:a2+b2≥2ab的运用.

| A. | (±3,0) | B. | (±5,0) | C. | (0,±5) | D. | (0,±$\sqrt{7}$) |
| A. | {x|-3≤x≤5} | B. | {x|-3<x<5} | C. | {x|x≥5或x≤-3} | D. | R |
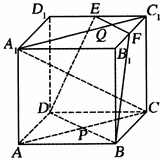 如图:在正方体ABCD-A1B1C1D1中,E,F分别为D1C1,B1C1的中点,AC∩BD=P,A1C1∩EF=Q,直线A1C与平面BDEF的交点为R.
如图:在正方体ABCD-A1B1C1D1中,E,F分别为D1C1,B1C1的中点,AC∩BD=P,A1C1∩EF=Q,直线A1C与平面BDEF的交点为R.