题目内容
已知函数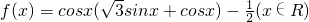
(Ⅰ)求函数f(x)的最小正周期及在区间 上的最大值和最小值;
上的最大值和最小值;
(Ⅱ)若 ,求cos2x0 的值.
,求cos2x0 的值.
解:(1)由题知: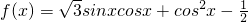 =
=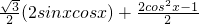 =
=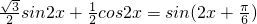 ,
,
所以函数f(x) 的最小正周期为π.…(5分)
因为 x∈ ,∴
,∴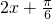 ∈
∈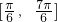 .…(7分)
.…(7分)
故当2x+ =
=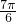 时,函数f(x)取得最小值为-
时,函数f(x)取得最小值为- ;当2x+
;当2x+ =
= 时,函数f(x)取得最大值为1,故函数在区间
时,函数f(x)取得最大值为1,故函数在区间 上的最大值为1,最小值为
上的最大值为1,最小值为 ..…(9分)
..…(9分)
(Ⅱ)由(1)可知 ,又因为
,又因为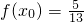 ,
,
所以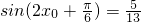 ,由
,由 ,得 2x0+
,得 2x0+ ∈[
∈[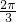 ,
,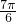 ],
],
从而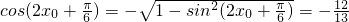 .…(12分)
.…(12分)
所以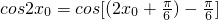 =
=
= . …(15分)
. …(15分)
分析:(1)利用两角和差的正弦化简函数f(x)的解析式为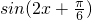 ,由此求得函数的最小正周期,再根据
,由此求得函数的最小正周期,再根据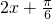 ∈
∈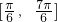 ,求得函数的最大值和最小值.
,求得函数的最大值和最小值.
(Ⅱ)由(1)可知 ,再根据 2x0+
,再根据 2x0+ 的范围利用同角三角函数的基本关系求得
的范围利用同角三角函数的基本关系求得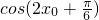 的值,再根据
的值,再根据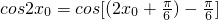 ,利用两角差
,利用两角差
的余弦公式求得结果.
点评:本题主要考查两角和差的正弦和余弦公式、二倍角公式、同角三角函数的基本关系的应用,正弦函数的定义域和值域,属于中档题.
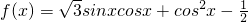 =
=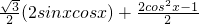 =
=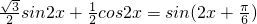 ,
,所以函数f(x) 的最小正周期为π.…(5分)
因为 x∈
 ,∴
,∴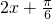 ∈
∈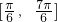 .…(7分)
.…(7分)故当2x+
 =
=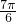 时,函数f(x)取得最小值为-
时,函数f(x)取得最小值为- ;当2x+
;当2x+ =
= 时,函数f(x)取得最大值为1,故函数在区间
时,函数f(x)取得最大值为1,故函数在区间 上的最大值为1,最小值为
上的最大值为1,最小值为 ..…(9分)
..…(9分)(Ⅱ)由(1)可知
 ,又因为
,又因为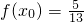 ,
,所以
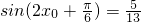 ,由
,由 ,得 2x0+
,得 2x0+ ∈[
∈[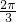 ,
,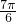 ],
],从而
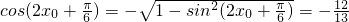 .…(12分)
.…(12分)所以
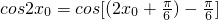 =
=
=
 . …(15分)
. …(15分)分析:(1)利用两角和差的正弦化简函数f(x)的解析式为
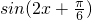 ,由此求得函数的最小正周期,再根据
,由此求得函数的最小正周期,再根据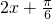 ∈
∈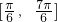 ,求得函数的最大值和最小值.
,求得函数的最大值和最小值.(Ⅱ)由(1)可知
 ,再根据 2x0+
,再根据 2x0+ 的范围利用同角三角函数的基本关系求得
的范围利用同角三角函数的基本关系求得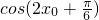 的值,再根据
的值,再根据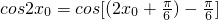 ,利用两角差
,利用两角差的余弦公式求得结果.
点评:本题主要考查两角和差的正弦和余弦公式、二倍角公式、同角三角函数的基本关系的应用,正弦函数的定义域和值域,属于中档题.

练习册系列答案
相关题目

 的定义域;
的定义域; 为何值时,函数值大于1.
为何值时,函数值大于1.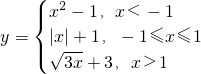 编写一程序求函数值.
编写一程序求函数值. 编写一程序求函数值.
编写一程序求函数值. 编写一程序求函数值.
编写一程序求函数值.