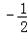题目内容
若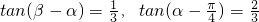 ,则tanβ=
,则tanβ=
- A.10
- B.5
- C.
- D.-8
D
分析:利用两角和的正切公式求出tan(β- )=tan[(β-α)+(α-
)=tan[(β-α)+(α- )]的值,再由tan(β-
)]的值,再由tan(β- )=
)=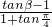 求出tanβ 的值.
求出tanβ 的值.
解答:∵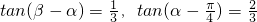 ,
,
∴tan(β- )=tan[(β-α)+(α-
)=tan[(β-α)+(α- )]=
)]=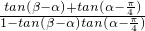 =
=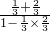 =
=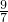 ,
,
故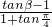 =
=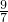 ,∴tanβ=-8.
,∴tanβ=-8.
故选:D.
点评:本题主要考查两角和差的正切公式的应用,角的变换是解题的关键,属于中档题.
分析:利用两角和的正切公式求出tan(β-
 )=tan[(β-α)+(α-
)=tan[(β-α)+(α- )]的值,再由tan(β-
)]的值,再由tan(β- )=
)=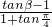 求出tanβ 的值.
求出tanβ 的值.解答:∵
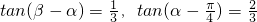 ,
,∴tan(β-
 )=tan[(β-α)+(α-
)=tan[(β-α)+(α- )]=
)]=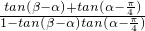 =
=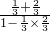 =
=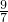 ,
,故
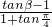 =
=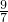 ,∴tanβ=-8.
,∴tanβ=-8.故选:D.
点评:本题主要考查两角和差的正切公式的应用,角的变换是解题的关键,属于中档题.

练习册系列答案
相关题目
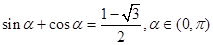 ,则tan
,则tan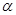 =
=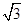 B.
B.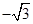 C.
C.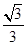 D.
D.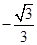
 ,则tanθ=
,则tanθ=


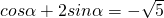 ,则tanα=
,则tanα=
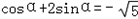 ,则tan
,则tan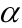 =
=