题目内容
己知椭圆的焦点在x轴上,它的一个焦点与抛物线x2=4y的焦点之间的距离为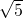 ,离心率e=
,离心率e=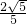 ,过椭圆的左焦点厂做一条与坐标轴不垂直的直线L交椭圆于A,B两点.
,过椭圆的左焦点厂做一条与坐标轴不垂直的直线L交椭圆于A,B两点.
(1)求椭圆的标准方程;
(2)设点M(m,0)是线段OF1上的一个动点,且(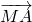 +
+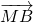 )⊥
)⊥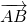 ,求m的取值范围.
,求m的取值范围.
解:(1)抛物线的焦点为(0,1),设椭圆的右焦点(c,0 ),则由题意可得 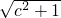 =
=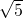 ,
,
∴c=2,∴再由离心率可得 a=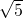 ,b=1,故椭圆的标准方程为
,b=1,故椭圆的标准方程为 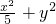 =1.
=1.
(2)设直线l的方程为 y=k(x+2),代入椭圆的方程化简可得 (1+5k2)x2+20k2x-5=0,
∴x1+x2= ,x1•x2=
,x1•x2=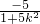 ,
,
∴(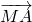 +
+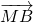 )=(x1-m,y1)+(x2-m,y2 )=(x1+x2-2m,y1+y2 ).
)=(x1-m,y1)+(x2-m,y2 )=(x1+x2-2m,y1+y2 ).
由(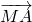 +
+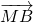 )⊥
)⊥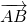 ,可得 (
,可得 (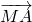 +
+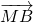 )•
)•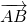 =(x1+x2-2m,y1+y2 )•(x2-x1,y2-y1)
=(x1+x2-2m,y1+y2 )•(x2-x1,y2-y1)
=(x1+x2-2m)(x2-x1)+(y2+y1)(y2-y1)=0,
化简可得 x1+x2-2m+k2(x1+x2+4)=0,∴2m=4k2-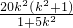 ,
,
∴m=-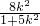 =-
=- .∵k2>0,∴0<
.∵k2>0,∴0<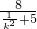 <
<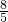 ,
,
∴-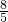 <m<0. 故m的取值范围是[-
<m<0. 故m的取值范围是[-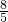 ,0).
,0).
分析:(1)根据题意设椭圆的右焦点(c,0 ),则由题意可得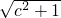 =
=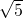 ,求出c值,由离心率可得 a,求出b值,即得椭圆的标准方程.
,求出c值,由离心率可得 a,求出b值,即得椭圆的标准方程.
(2)设直线l的方程为 y=k(x+2),代入椭圆的方程化简,把根与系数的关系代入(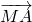 +
+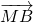 )•
)•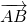 =0,解得 m=-
=0,解得 m=-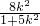 =-
=- ,再利用不等式的性质求出m的取值范围.
,再利用不等式的性质求出m的取值范围.
点评:本题考查求椭圆的标准方程,两个向量的数量积公式,不等式的性质,求出m=-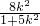 =-
=- ,是解题的关键,
,是解题的关键,
属于中档题.
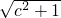 =
=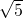 ,
,∴c=2,∴再由离心率可得 a=
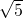 ,b=1,故椭圆的标准方程为
,b=1,故椭圆的标准方程为 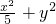 =1.
=1.(2)设直线l的方程为 y=k(x+2),代入椭圆的方程化简可得 (1+5k2)x2+20k2x-5=0,
∴x1+x2=
 ,x1•x2=
,x1•x2=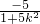 ,
,∴(
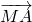 +
+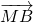 )=(x1-m,y1)+(x2-m,y2 )=(x1+x2-2m,y1+y2 ).
)=(x1-m,y1)+(x2-m,y2 )=(x1+x2-2m,y1+y2 ).由(
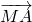 +
+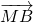 )⊥
)⊥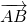 ,可得 (
,可得 (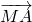 +
+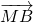 )•
)•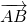 =(x1+x2-2m,y1+y2 )•(x2-x1,y2-y1)
=(x1+x2-2m,y1+y2 )•(x2-x1,y2-y1) =(x1+x2-2m)(x2-x1)+(y2+y1)(y2-y1)=0,
化简可得 x1+x2-2m+k2(x1+x2+4)=0,∴2m=4k2-
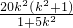 ,
,∴m=-
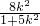 =-
=- .∵k2>0,∴0<
.∵k2>0,∴0<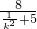 <
<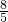 ,
,∴-
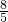 <m<0. 故m的取值范围是[-
<m<0. 故m的取值范围是[-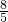 ,0).
,0).分析:(1)根据题意设椭圆的右焦点(c,0 ),则由题意可得
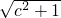 =
=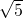 ,求出c值,由离心率可得 a,求出b值,即得椭圆的标准方程.
,求出c值,由离心率可得 a,求出b值,即得椭圆的标准方程.(2)设直线l的方程为 y=k(x+2),代入椭圆的方程化简,把根与系数的关系代入(
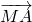 +
+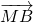 )•
)•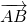 =0,解得 m=-
=0,解得 m=-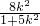 =-
=- ,再利用不等式的性质求出m的取值范围.
,再利用不等式的性质求出m的取值范围.点评:本题考查求椭圆的标准方程,两个向量的数量积公式,不等式的性质,求出m=-
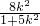 =-
=- ,是解题的关键,
,是解题的关键,属于中档题.

练习册系列答案
 名题金卷系列答案
名题金卷系列答案
相关题目
 给出下列5个命题:
给出下列5个命题: