题目内容

函数f(x)=x3+sinx+1(x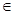R)，若f(a)=2,则f(－a)的值为                               (　　)

A．3                  B．0              C．－1            D．－2

 

【答案】

B

【解析】解：因为f(x)=x3+sinx+1(x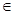R)，故g(x)=f(x)-1= x3+sinx为奇函数，则

g(a)+g(-a)=0,即f(a)+f(－a)=2，因为f(a)=2，所以f(－a)=0，故选B

 

练习册系列答案
相关题目
设M是由满足下列条件的函数f（x）构成的集合：“①方程f（x）-x=0有实数根；②函数f（x）的导数f'（x）满足0＜f'（x）＜1．”
（1）判断函数f(x)=
x
3
+
cosx
4
是否是集合M中的元素，并说明理由；
（2）集合M中的元素f（x）具有下面的性质：若f（x）的定义域为D，则对于任意[m，n]30D，都存在-15P[m，n]，使得等式f（n）-f（m）=（n-m）f'（x0）成立”，试用这一性质证明：方程f（x）-x=0只有一个实数根；
（3）设
1
5
是方程f（x）-x=0的实数根，求证：对于f（x）定义域中任意的x2，x3，当|x2-x1|＜1，且|x3-x1|＜1时，|f（x3）-f（x2）|＜2．
对于三次函数f（x）=ax3+bx2+cx+d（a≠0），定义：设f″（x）是函数y=f（x）的导数y=f′（x）的导数，若方程f″（x）=0有实数解x0，则称点（x0，f（x0））为函数y=f（x）的“拐点”．有同学发现“任何一个三次函数都有‘拐点’；任何一个三次函数都有对称中心；且‘拐点’就是对称中心．”请你将这一发现为条件，函数f(x)=x3-
3
2
x2+3x-
1
4
，则它的对称中心为
(
1
2
，1)
(
1
2
，1)
；计算f(
1
2013
)+f(
2
2013
)+f(
3
2013
)+…+f(
2012
2013
)=
2012
2012
．
已知函数f(x)=-x3+
1
2
ax2+b．
（1）若y=f（x）在x=1处的极值为
5
2
，求y=f（x）的解析式并确定其单调区间；
（2）当x∈（0，1]时，若y=f（x）的图象上任意一点处的切线的倾斜角为θ，求当0≤θ≤
π
4
时a的取值范围．
（2009•孝感模拟）命题p：方程2x2+mx-2m2-5m-3=0有一正根一负根；
命题q：函数f(x)=x3+mx2+(m+
43
)x+6在R上有极值；
若命题“p或q”为真，“p且q”为假，求实数m的取值范围．
判断函数f(x)=
x3(ax-1)ax+1
(a＞0，a≠1)的奇偶性，并加以证明．

违法和不良信息举报电话：027-86699610 举报邮箱：58377363@163.com

精英家教网