题目内容
函数f(x)=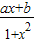 是定义在(-1,1)的奇函数,且f(
是定义在(-1,1)的奇函数,且f( )=
)= .
.(1)确定f(x)的解析式;
(2)判断函数在(-1,1)上的单调性;
(3)解不等式f(t-1)+f(t)<0.
【答案】分析:(1)若奇函数在x=0处有定义,则f(0)=0,代入即可得b,再由f( )=
)= 代入即可得a值
代入即可得a值
(2)因为函数为奇函数,故只需判断x>0时函数的单调性即可,利用单调性定义即可证明
(3)利用函数的单调性和奇偶性将不等式中的f脱去,等价转化为关于t的不等式组,解之即可
解答:解:(1)∵函数f(x)= 是定义在(-1,1)的奇函数
是定义在(-1,1)的奇函数
∴f(0)=0,即得b=0
∵f( )=
)= .
.
∴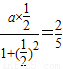 ,即得a=1
,即得a=1
∴f(x)=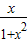
(2)设任意x1,x2∈(0,1),且x1<x2
则f(x1)-f(x2)=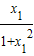 -
-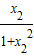
=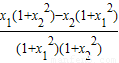
=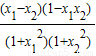 <0
<0
即f(x1)<f(x2)
∴函数f(x)在(0,1)上为增函数
∵函数f(x)是定义在(-1,1)的奇函数
∴函数f(x)在(-1,1)上为增函数
(3)不等式f(t-1)+f(t)<0
?f(t-1)<-f(t)
?f(t-1)<f(-t) (根据奇函数的性质)
? (根据定义域和单调性)
(根据定义域和单调性)
?0<t<
点评:本题综合考查了函数的奇偶性和函数的单调性,奇函数的性质,函数单调性的判断方法,利用函数性质解不等式
 )=
)= 代入即可得a值
代入即可得a值(2)因为函数为奇函数,故只需判断x>0时函数的单调性即可,利用单调性定义即可证明
(3)利用函数的单调性和奇偶性将不等式中的f脱去,等价转化为关于t的不等式组,解之即可
解答:解:(1)∵函数f(x)=
 是定义在(-1,1)的奇函数
是定义在(-1,1)的奇函数∴f(0)=0,即得b=0
∵f(
 )=
)= .
.∴
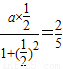 ,即得a=1
,即得a=1∴f(x)=
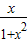
(2)设任意x1,x2∈(0,1),且x1<x2
则f(x1)-f(x2)=
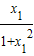 -
-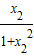
=
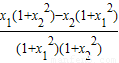
=
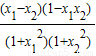 <0
<0即f(x1)<f(x2)
∴函数f(x)在(0,1)上为增函数
∵函数f(x)是定义在(-1,1)的奇函数
∴函数f(x)在(-1,1)上为增函数
(3)不等式f(t-1)+f(t)<0
?f(t-1)<-f(t)
?f(t-1)<f(-t) (根据奇函数的性质)
?
 (根据定义域和单调性)
(根据定义域和单调性)?0<t<

点评:本题综合考查了函数的奇偶性和函数的单调性,奇函数的性质,函数单调性的判断方法,利用函数性质解不等式

练习册系列答案
 名校课堂系列答案
名校课堂系列答案
相关题目