题目内容
14.向量$\overrightarrow{a}$,$\overrightarrow{b}$满足|$\overrightarrow{a}$|=3,|$\overrightarrow{b}$|=4,|$\overrightarrow{a}$+$\overrightarrow{b}$|=5,求|$\overrightarrow{a}$-$\overrightarrow{b}$|的值.分析 由|$\overrightarrow{a}$|=3,|$\overrightarrow{b}$|=4,|$\overrightarrow{a}$+$\overrightarrow{b}$|=5,可得$\sqrt{{\overrightarrow{a}}^{2}+{\overrightarrow{b}}^{2}+2\overrightarrow{a}•\overrightarrow{b}}$=5,解得$\overrightarrow{a}•\overrightarrow{b}$=0,可得|$\overrightarrow{a}$-$\overrightarrow{b}$|=$\sqrt{{\overrightarrow{a}}^{2}+{\overrightarrow{b}}^{2}-2\overrightarrow{a}•\overrightarrow{b}}$.
解答 解:∵|$\overrightarrow{a}$|=3,|$\overrightarrow{b}$|=4,|$\overrightarrow{a}$+$\overrightarrow{b}$|=5,
∴$\sqrt{{\overrightarrow{a}}^{2}+{\overrightarrow{b}}^{2}+2\overrightarrow{a}•\overrightarrow{b}}$=$\sqrt{9+16+2\overrightarrow{a}•\overrightarrow{b}}=5$,解得$\overrightarrow{a}•\overrightarrow{b}$=0,
∴|$\overrightarrow{a}$-$\overrightarrow{b}$|=$\sqrt{{\overrightarrow{a}}^{2}+{\overrightarrow{b}}^{2}-2\overrightarrow{a}•\overrightarrow{b}}$=$\sqrt{{3}^{2}+{4}^{2}}$=5.
点评 本题考查了向量数量积运算性质,考查了推理能力与计算能力,属于中档题.

 暑假作业暑假快乐练西安出版社系列答案
暑假作业暑假快乐练西安出版社系列答案| A. | $y=1o{g_{\frac{1}{2}}}x$ | B. | y=2x | C. | $y=\frac{1}{x}$ | D. | $y={x^{-\frac{2}{3}}}$ |
| A. | AB边中线的中点 | B. | AB边的中线的四等分点(非中点) | ||
| C. | 重心 | D. | AB边中线的三等分点(非重心) |
| 气温/(℃) | 4 | 2 | 1 | -1 | -3 |
| 杯数 | 24 | 36 | 40 | 49 | 61 |
| A. | y=4x+36 | B. | y=5x+20 | C. | y=-4x+44 | D. | y=-5x+45 |
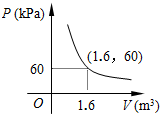 某气球内充满了一定质量的气体,当温度不变时,气球内气体的气压P ( kPa ) 是气体体积V ( m3) 的反比例函数,其图象如图所示.当气球内的气压大于120kPa时,气球将爆炸.为了安全起见,气球的体积应( )
某气球内充满了一定质量的气体,当温度不变时,气球内气体的气压P ( kPa ) 是气体体积V ( m3) 的反比例函数,其图象如图所示.当气球内的气压大于120kPa时,气球将爆炸.为了安全起见,气球的体积应( )| A. | 不小于$\frac{5}{4}$m3 | B. | 小于$\frac{5}{4}$m3 | C. | 不小于$\frac{4}{5}$m3 | D. | 不大于$\frac{4}{5}$m3 |