题目内容

如图所示,定椭圆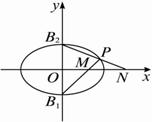=1(a>b>0)上的动点P不重合于短轴两端点B1与B2,设两直线B1P、B2P与x轴分别相交于点M、N.问|OM|·|ON|是否为定值?

      

解析:取P(a,0),则M(a,0)、N(a,0),从而?|OM|·|ON|=a2;?

       取P(c,),则M(,0),N(,0).?

       故|OM|·|ON|=a2.?

       于是猜想|OM|·|ON|=a2为定值.?

       证明:设P(acosθ,bsinθ),其中|sinθ|≠1,?

       且设M(x1,0),N(x2,0).?

       ∵三点B、M、P共线,且三点B2、N、P共线,?

       ∴,,?

       即x1=,x2=.?

       则|OM|·|ON|=|x1|·|x2|=|x1·x2|?

       =||=||＝a2(定值).?

       故|OM|·|ON|为定值.

练习册系列答案
相关题目
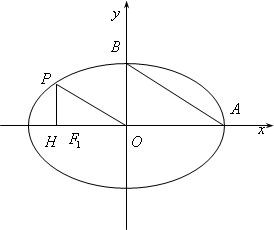精英家教网已知在椭圆C：
x2
a2
+
y2
b2
=1(a＞b＞0)中，F1（-c，0）（c＞0）是椭圆的左焦点，A（a，0），B（0，b）分别是椭圆的右顶点和上顶点，点O是椭圆的中心．又点P在椭圆上，且满足条件：OP∥AB，点H是点P在x轴上的投影．
（Ⅰ）求证：当a取定值时，点H必为定点；
（Ⅱ）如图所示，当点P在第二象限，以OP为直径的圆与直线AB相切，且四边形ABPH的面积等于3+
2
，求椭圆的标准方程．
作斜率为
1
3
的直线l与椭圆C：
x2
36
+
y2
4
=1交于A，B两点（如图所示），且P(3
2
，
2
)在直线l的左上方．
（1）证明：△PAB的内切圆的圆心在一条定直线上；
（2）若∠APB=60°，求△PAB的面积．
如图所示，椭圆C：
 x2   
b2
+
y2    
a2
=1(a＞b＞0)的焦点为F1（0，c），F2（0，-c）（c＞0），抛物线x2=2py（p＞0）的焦点与F1重合，过F2的直线l与抛物线P相切，切点在第一象限，且与椭圆C相交于A，B两点，且
F2B
=λ
AF2
．
（1）求证：切线l的斜率为定值；
（2）当λ∈[2，4]时，求椭圆的离心率e的取值范围．

如图所示，A为椭圆＝1(a＞b0)上的一个动点，弦AB、AC分别过焦点F1、F2．当AC垂直于x轴时，恰好AF1∶AF2＝3∶1．

(1)求该椭圆的离心率；

(2)设，试判断λ1＋λ2是否为定值？若是，则求出该定值；若不是，请说明理由．

违法和不良信息举报电话：027-86699610 举报邮箱：58377363@163.com

精英家教网