题目内容
已知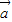 ,
,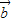 是两个非零向量,且
是两个非零向量,且 =
=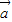 +
+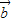 ,
,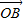 =
=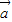 +2
+2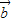 ,
, =
=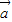 +3
+3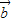 ,则
,则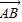 与
与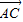 的夹角为 .
的夹角为 .
【答案】分析:化简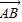 、
、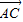 可得
可得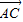 =2
=2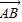 ,从而求得
,从而求得 与
与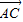 的夹角.
的夹角.
解答:解: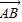 =
=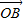 -
-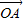 =(
=(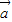 +2
+2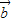 )-(
)-( +
+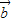 )=
)=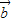 ,
,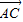 =
=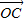 -
-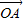 =(
=(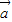 +3
+3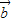 )-(
)-(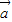 +
+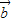 )=2
)=2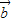 ,∴
,∴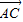 =2
=2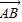 .
.
故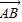 与
与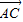 的夹角为0°,
的夹角为0°,
故答案为 0°.
点评:本题主要考查两个向量的加减法的法则,以及其几何意义,两个向量共线性质,属于中档题.
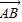 、
、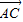 可得
可得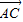 =2
=2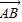 ,从而求得
,从而求得 与
与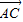 的夹角.
的夹角.解答:解:
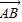 =
=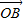 -
-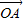 =(
=(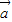 +2
+2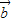 )-(
)-( +
+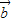 )=
)=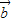 ,
,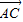 =
=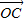 -
-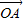 =(
=(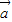 +3
+3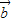 )-(
)-(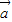 +
+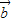 )=2
)=2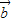 ,∴
,∴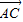 =2
=2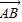 .
.故
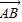 与
与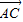 的夹角为0°,
的夹角为0°,故答案为 0°.
点评:本题主要考查两个向量的加减法的法则,以及其几何意义,两个向量共线性质,属于中档题.

练习册系列答案
相关题目
 ,
, 是两个非零向量,当
是两个非零向量,当 +t
+t (t∈R)的模取最小值时,
(t∈R)的模取最小值时,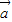 与
与 共线且同向,求证:
共线且同向,求证: 与
与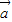 +t
+t 垂直.
垂直. ,
, 是两个非零向量,且|
是两个非零向量,且| |=|
|=| |=|
|=| -
- |,则
|,则 与
与 +
+ 的夹角大小为 .
的夹角大小为 . 、
、 是两个非零向量,且满足|
是两个非零向量,且满足| |=|
|=| |=|
|=| -
- |,求:
|,求: 与
与 +
+ 的夹角;
的夹角; 的值.
的值.