题目内容
抛物线C的方程为y=ax2(a<0),过抛物线C上一点P(x0,y0)(x0≠0)作斜率为k1,k2的两条直线分别交抛物线C于A(x1,y1)、B(x2,y2)两点(P、A、B三点互不相同)且满足k2+λk1=0(λ≠0且
λ≠-1),
(Ⅰ)求抛物线C的焦点坐标和准线方程;
(Ⅱ)设直线AB上一点M,满足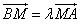 ,证明线段PM的中点在y轴上;
,证明线段PM的中点在y轴上;
(Ⅲ)当λ=1时,若点P的坐标为(1,-1),求∠PAB为钝角时点A的纵坐标y1的取值范围。
λ≠-1),
(Ⅰ)求抛物线C的焦点坐标和准线方程;
(Ⅱ)设直线AB上一点M,满足
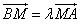 ,证明线段PM的中点在y轴上;
,证明线段PM的中点在y轴上;(Ⅲ)当λ=1时,若点P的坐标为(1,-1),求∠PAB为钝角时点A的纵坐标y1的取值范围。
(Ⅰ)解:由抛物线C的方程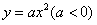 ,得焦点坐标为
,得焦点坐标为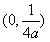 ,准线方程为
,准线方程为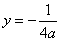 ;
;
(Ⅱ)证明:设直线PA的方程为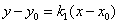 ,
,
直线PB的方程为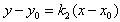 ,
,
点 的坐标是方程组
的坐标是方程组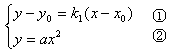 的解,
的解,
将②式代入①式得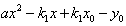 =0,
=0,
于是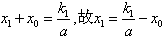 ,③
,③
又点 的坐标是方程组
的坐标是方程组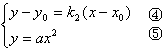 的解,
的解,
将⑤式代入④式得 ,
,
由已知得,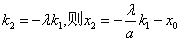 , ⑥
, ⑥
设点M的坐标为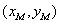 ,
,
由 ,
,
将③式和⑥式代入上式得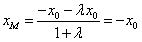 ,即
,即 ,
,
所以线段PM的中点在y轴上。
(Ⅲ)解:因为点P(1,-1)在抛物线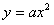 上,所以a=-1,
上,所以a=-1,
抛物线的方程为 ,
,
由③式知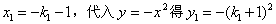 ,
,
将λ=1代入⑥式得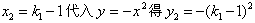 ,
,
因此,直线PA、PB分别与抛物线C的交点A、B的坐标为
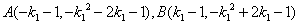 ,
,
于是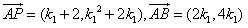 ,
,
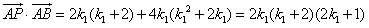 ,
,
因∠PAB为钝角且P、A、B三点互不相同,
故必有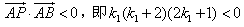 ,
,
求得k1的取值范围为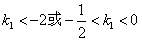 ,
,
又点A的纵坐标y1满足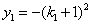 ,
,
故当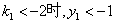 ;当
;当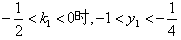 ;
;
所以∠PAB为钝角时点A的纵坐标y1的取值范围为 。
。
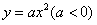 ,得焦点坐标为
,得焦点坐标为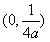 ,准线方程为
,准线方程为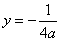 ;
;(Ⅱ)证明:设直线PA的方程为
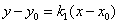 ,
,直线PB的方程为
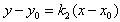 ,
,点
 的坐标是方程组
的坐标是方程组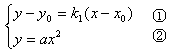 的解,
的解,将②式代入①式得
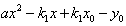 =0,
=0,于是
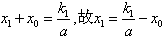 ,③
,③ 又点
 的坐标是方程组
的坐标是方程组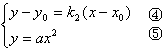 的解,
的解,将⑤式代入④式得
 ,
,由已知得,
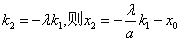 , ⑥
, ⑥ 设点M的坐标为
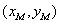 ,
,由
 ,
,将③式和⑥式代入上式得
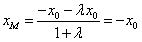 ,即
,即 ,
,所以线段PM的中点在y轴上。
(Ⅲ)解:因为点P(1,-1)在抛物线
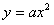 上,所以a=-1,
上,所以a=-1,抛物线的方程为
 ,
,由③式知
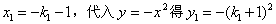 ,
,将λ=1代入⑥式得
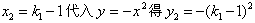 ,
,因此,直线PA、PB分别与抛物线C的交点A、B的坐标为
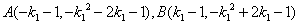 ,
,于是
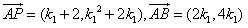 ,
,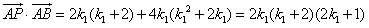 ,
,因∠PAB为钝角且P、A、B三点互不相同,
故必有
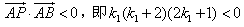 ,
,求得k1的取值范围为
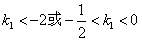 ,
,又点A的纵坐标y1满足
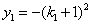 ,
,故当
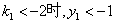 ;当
;当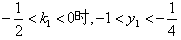 ;
;所以∠PAB为钝角时点A的纵坐标y1的取值范围为
 。
。
练习册系列答案
 阅读快车系列答案
阅读快车系列答案
相关题目
