题目内容
已知函数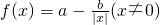 .
.
(1)若函数f(x)是(0,+∞)上的增函数,求实数b的取值范围;
(2)当b=2时,若不等式f(x)<x在区间(1,+∞)上恒成立,求实数a的取值范围;
(3)对于函数g(x)若存在区间[m,n](m<n),使x∈[m,n]时,函数g(x)的值域也是[m,n],则称g(x)是[m,n]上的闭函数.若函数f(x)是某区间上的闭函数,试探求a,b应满足的条件.
解:(1)当x∈(0,+∞)时,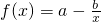
设x1,x2∈(0,+∞)且x1<x2,由f(x)是(0,+∞)上的增函数,则f(x1)<f(x2)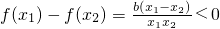
由x1<x2,x1,x2∈(0,+∞)知x1-x2<0,x1x2>0,所以b>0,即b∈(0,+∞)
(2)当b=2时, 在x∈(1,+∞)上恒成立,即
在x∈(1,+∞)上恒成立,即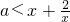
因为 ,当
,当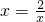 即
即 时取等号,
时取等号,
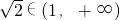 ,所以
,所以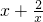 在x∈(1,+∞)上的最小值为
在x∈(1,+∞)上的最小值为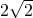 .则
.则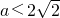
(3)因为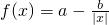 的定义域是(-∞,0)∪(0,+∞),
的定义域是(-∞,0)∪(0,+∞),
设f(x)是区间[m,n]上的闭函数,则mn>0且b≠0
①若0<m<n
当b>0时,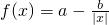 是(0,+∞)上的增函数,则
是(0,+∞)上的增函数,则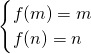 ,
,
所以方程 在(0,+∞)上有两不等实根,
在(0,+∞)上有两不等实根,
即x2-ax+b=0在(0,+∞)上有两不等实根,所以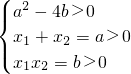 ,即a>0,b>0且a2-4b>0
,即a>0,b>0且a2-4b>0
当b<0时,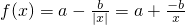 在(0,+∞)上递减,则
在(0,+∞)上递减,则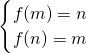 ,即
,即 ,
,
所以a=0,b<0
②若m<n<0
当b>0时, 是(-∞,0)上的减函数,所以
是(-∞,0)上的减函数,所以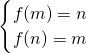 ,即
,即 ,
,
所以a=0,b>0
当b<0 是(-∞,0)上的增函数,所以
是(-∞,0)上的增函数,所以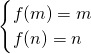 所以方程
所以方程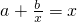 在(-∞,0)上有两不等实根,即x2+ax-b=0在(-∞,0)上有两不等实根,
在(-∞,0)上有两不等实根,即x2+ax-b=0在(-∞,0)上有两不等实根,
所以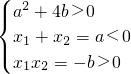 即a<0,b<0且a2+4b>0
即a<0,b<0且a2+4b>0
综上知:a=0,b≠0或a<0,b<0且a2+4b>0或a>0,b>0且a2-4b>0.
即:a=0,b≠0或ab>0且a2-4|b|>0
分析:(1)先去绝对值,然后设x1,x2∈(0,+∞)且x1<x2,根据函数f(x)是(0,+∞)上的增函数,则f(x1)<f(x2),建立关系式,化简整理可求出b的取值范围;
(2)若不等式f(x)<x在区间(1,+∞)上恒成立,可转化成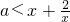 在(1,+∞)上恒成立,求出不等式右边的最小值即,使得a小于此最小值即可;
在(1,+∞)上恒成立,求出不等式右边的最小值即,使得a小于此最小值即可;
(3)设f(x)是区间[m,n]上的闭函数,则mn>0且b≠0,讨论m与n同正与同负两种情形,以及讨论b的正负,根据函数的单调性建立关系式,即可求出a与b满足的条件.
点评:本题主要考查了函数的单调性,以及函数恒成立和函数的值域,是一道综合题,有一定的难度.
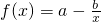
设x1,x2∈(0,+∞)且x1<x2,由f(x)是(0,+∞)上的增函数,则f(x1)<f(x2)
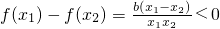
由x1<x2,x1,x2∈(0,+∞)知x1-x2<0,x1x2>0,所以b>0,即b∈(0,+∞)
(2)当b=2时,
 在x∈(1,+∞)上恒成立,即
在x∈(1,+∞)上恒成立,即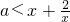
因为
 ,当
,当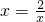 即
即 时取等号,
时取等号,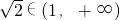 ,所以
,所以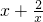 在x∈(1,+∞)上的最小值为
在x∈(1,+∞)上的最小值为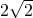 .则
.则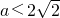
(3)因为
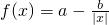 的定义域是(-∞,0)∪(0,+∞),
的定义域是(-∞,0)∪(0,+∞),设f(x)是区间[m,n]上的闭函数,则mn>0且b≠0
①若0<m<n
当b>0时,
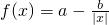 是(0,+∞)上的增函数,则
是(0,+∞)上的增函数,则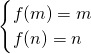 ,
,所以方程
 在(0,+∞)上有两不等实根,
在(0,+∞)上有两不等实根,即x2-ax+b=0在(0,+∞)上有两不等实根,所以
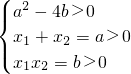 ,即a>0,b>0且a2-4b>0
,即a>0,b>0且a2-4b>0当b<0时,
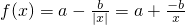 在(0,+∞)上递减,则
在(0,+∞)上递减,则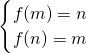 ,即
,即 ,
,所以a=0,b<0
②若m<n<0
当b>0时,
 是(-∞,0)上的减函数,所以
是(-∞,0)上的减函数,所以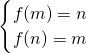 ,即
,即 ,
,所以a=0,b>0
当b<0
 是(-∞,0)上的增函数,所以
是(-∞,0)上的增函数,所以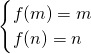 所以方程
所以方程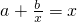 在(-∞,0)上有两不等实根,即x2+ax-b=0在(-∞,0)上有两不等实根,
在(-∞,0)上有两不等实根,即x2+ax-b=0在(-∞,0)上有两不等实根,所以
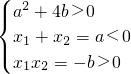 即a<0,b<0且a2+4b>0
即a<0,b<0且a2+4b>0综上知:a=0,b≠0或a<0,b<0且a2+4b>0或a>0,b>0且a2-4b>0.
即:a=0,b≠0或ab>0且a2-4|b|>0
分析:(1)先去绝对值,然后设x1,x2∈(0,+∞)且x1<x2,根据函数f(x)是(0,+∞)上的增函数,则f(x1)<f(x2),建立关系式,化简整理可求出b的取值范围;
(2)若不等式f(x)<x在区间(1,+∞)上恒成立,可转化成
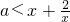 在(1,+∞)上恒成立,求出不等式右边的最小值即,使得a小于此最小值即可;
在(1,+∞)上恒成立,求出不等式右边的最小值即,使得a小于此最小值即可;(3)设f(x)是区间[m,n]上的闭函数,则mn>0且b≠0,讨论m与n同正与同负两种情形,以及讨论b的正负,根据函数的单调性建立关系式,即可求出a与b满足的条件.
点评:本题主要考查了函数的单调性,以及函数恒成立和函数的值域,是一道综合题,有一定的难度.

练习册系列答案
相关题目





 的定义域为
的定义域为 ,部分函数值如表所示,其导函数的图象如图所示,若正数
,部分函数值如表所示,其导函数的图象如图所示,若正数 ,
, 满足
满足 ,则
,则 的取值范围是( )
的取值范围是( ) 



 B.
B. C.
C. D.
D.
 ,如果满足;对任意
,如果满足;对任意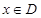 ,存在常数
,存在常数 ,都有
,都有 成立,则称
成立,则称 ,
,
 时,求函数
时,求函数 上的值域,并判断函数
上的值域,并判断函数 上是以3为上界函数值,求实数
上是以3为上界函数值,求实数 的取值范围;
的取值范围; ,求函数
,求函数 在
在 上的上界T的取值范围。
上的上界T的取值范围。 ,如果满足;对任意
,如果满足;对任意 ,存在常数
,存在常数 ,都有
,都有 成立,则称
成立,则称 ,
,
 时,求函数
时,求函数 上的值域,并判断函数
上的值域,并判断函数 上是以3为上界函数值,求实数
上是以3为上界函数值,求实数 的取值范围;
的取值范围; ,求函数
,求函数 在
在 上的上界T的取值范围。
上的上界T的取值范围。