题目内容
把函数y=lnx-2的图象按向量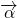 =(-1,2)平移得到函数y=f(x)的图象.
=(-1,2)平移得到函数y=f(x)的图象.
(1)若x>0,证明;f(x)>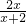 ;
;
(2不等式 x2≤f(x2)+m2-2bm-3对b∈[-1,1],x∈[-1,1]时恒成立,求实数m的取值范围.
x2≤f(x2)+m2-2bm-3对b∈[-1,1],x∈[-1,1]时恒成立,求实数m的取值范围.
(1)证明:∵函数y=lnx-2的图象按向量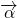 =(-1,2)平移得到函数y=f(x)的图象
=(-1,2)平移得到函数y=f(x)的图象
∴f(x)=ln(x+1),
构建函数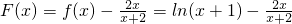 ,
,
求导函数得
∵x>0,∴F′(x)>0,
∴在(0,+∞)上,F(x)为增函数.
∴F(x)>F(0)=0,
∴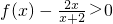
∴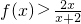 ;
;
(2)解:∵不等式 x2≤f(x2)+m2-2bm-3对b∈[-1,1],x∈[-1,1]时恒成立
x2≤f(x2)+m2-2bm-3对b∈[-1,1],x∈[-1,1]时恒成立
∴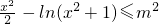 -2bm-3,对b∈[-1,1],x∈[-1,1]时恒成立
-2bm-3,对b∈[-1,1],x∈[-1,1]时恒成立
设g(x)=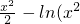 +1),
+1),
则g′(x)=x-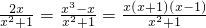 ,
,
x∈(-1,0)时,g′(x)>0,x∈(0,1)时,g′(x)<0.
∴x∈(-1,1)时,g(x)≤g(0)=0.
∴x∈(-1,1)时,0≤m2-2bm-3,
∴问题可化为对b∈[-1,1]时,0≤m2-2bm-3恒成立,即使2mb+3-m2≤0恒成立.
∴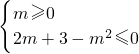 或
或 ,
,
∴m≤-3或m≥3
综上,实数m的取值范围是(-∞,-3]∪[3,+∞).
分析:(1)先根据向量的平移,求得f(x)=ln(x+1),再构建函数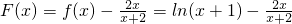 ,确定函数的单调性,从而可证不等式;
,确定函数的单调性,从而可证不等式;
(2)不等式 x2≤f(x2)+m2-2bm-3对b∈[-1,1],x∈[-1,1]时恒成立,等价于
x2≤f(x2)+m2-2bm-3对b∈[-1,1],x∈[-1,1]时恒成立,等价于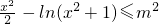 -2bm-3,对b∈[-1,1],x∈[-1,1]时恒成立,求出左边函数的最大值,进一步可化为对b∈[-1,1]时,0≤m2-2bm-3恒成立,即使2mb+3-m2≤0恒成立,从而可求实数m的取值范围.
-2bm-3,对b∈[-1,1],x∈[-1,1]时恒成立,求出左边函数的最大值,进一步可化为对b∈[-1,1]时,0≤m2-2bm-3恒成立,即使2mb+3-m2≤0恒成立,从而可求实数m的取值范围.
点评:本题重点考查导数知识的运用,考查利用导数确定函数的单调性,进而证明不等式,考查恒成立问题的理解与处理,综合性强.
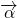 =(-1,2)平移得到函数y=f(x)的图象
=(-1,2)平移得到函数y=f(x)的图象∴f(x)=ln(x+1),
构建函数
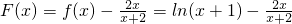 ,
,求导函数得

∵x>0,∴F′(x)>0,
∴在(0,+∞)上,F(x)为增函数.
∴F(x)>F(0)=0,
∴
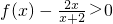
∴
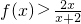 ;
;(2)解:∵不等式
 x2≤f(x2)+m2-2bm-3对b∈[-1,1],x∈[-1,1]时恒成立
x2≤f(x2)+m2-2bm-3对b∈[-1,1],x∈[-1,1]时恒成立∴
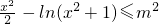 -2bm-3,对b∈[-1,1],x∈[-1,1]时恒成立
-2bm-3,对b∈[-1,1],x∈[-1,1]时恒成立设g(x)=
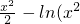 +1),
+1),则g′(x)=x-
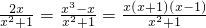 ,
,x∈(-1,0)时,g′(x)>0,x∈(0,1)时,g′(x)<0.
∴x∈(-1,1)时,g(x)≤g(0)=0.
∴x∈(-1,1)时,0≤m2-2bm-3,
∴问题可化为对b∈[-1,1]时,0≤m2-2bm-3恒成立,即使2mb+3-m2≤0恒成立.
∴
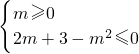 或
或 ,
,∴m≤-3或m≥3
综上,实数m的取值范围是(-∞,-3]∪[3,+∞).
分析:(1)先根据向量的平移,求得f(x)=ln(x+1),再构建函数
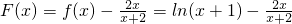 ,确定函数的单调性,从而可证不等式;
,确定函数的单调性,从而可证不等式;(2)不等式
 x2≤f(x2)+m2-2bm-3对b∈[-1,1],x∈[-1,1]时恒成立,等价于
x2≤f(x2)+m2-2bm-3对b∈[-1,1],x∈[-1,1]时恒成立,等价于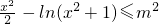 -2bm-3,对b∈[-1,1],x∈[-1,1]时恒成立,求出左边函数的最大值,进一步可化为对b∈[-1,1]时,0≤m2-2bm-3恒成立,即使2mb+3-m2≤0恒成立,从而可求实数m的取值范围.
-2bm-3,对b∈[-1,1],x∈[-1,1]时恒成立,求出左边函数的最大值,进一步可化为对b∈[-1,1]时,0≤m2-2bm-3恒成立,即使2mb+3-m2≤0恒成立,从而可求实数m的取值范围.点评:本题重点考查导数知识的运用,考查利用导数确定函数的单调性,进而证明不等式,考查恒成立问题的理解与处理,综合性强.

练习册系列答案
 智趣暑假温故知新系列答案
智趣暑假温故知新系列答案 英语小英雄天天默写系列答案
英语小英雄天天默写系列答案 暑假作业安徽少年儿童出版社系列答案
暑假作业安徽少年儿童出版社系列答案
相关题目
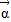 =(-1,2)平移得到函数y=f(x)的图象.
=(-1,2)平移得到函数y=f(x)的图象. ;
; x2≤f(x2)+m2-2bm-3对b∈[-1,1],x∈[-1,1]时恒成立,求实数m的取值范围.
x2≤f(x2)+m2-2bm-3对b∈[-1,1],x∈[-1,1]时恒成立,求实数m的取值范围.