题目内容
请选做一题,都做时按先做的题判分,都做不加分.(1)已知向量
| m |
| n |
| 3 |
| m |
| n |
①求函数f(x)的最小正周期和值域;
②在△ABC中,角A、B、C所对的边分别是a、b、c,若f(
| A |
| 2 |
(2)已知锐角△ABC,sin(A+B)=
| 3 |
| 5 |
| 1 |
| 5 |
①求证:tanA=2tanB;
②设AB=3,求AB边上的高CD的长.
分析:(1)①用向量的数量积将函数f(x)的解析式表示出来后化简成y=Asin(ωx+θ)可得答案.
②将
代入函数f(x)可求出A的值,再由余弦定理可得到b=c,从而得到答案.
(2)①根据两角和与差的正弦公式展开,可得sinAcosB=2cosAsinB,进而得到答案.
②根据正切函数的两角和公式,得出tanA与tanB的关系,再通过①中tanA=2tanB求出tanA和tanB的值.再通过AB=AD+BD=
+
进而求出CD的值.
②将
| A |
| 2 |
(2)①根据两角和与差的正弦公式展开,可得sinAcosB=2cosAsinB,进而得到答案.
②根据正切函数的两角和公式,得出tanA与tanB的关系,再通过①中tanA=2tanB求出tanA和tanB的值.再通过AB=AD+BD=
| CD |
| tanA |
| CD |
| tanB |
解答:解:(1)①f(x)=
•
=(2sinx,cosx-sinx)•(
cosx,cosx+sinx)
=2
sinxcosx+cos2x-sin2x=
sin2x+cos2x=2sin(2x+
)
T=
=π,值域为[-2,2].
②∵f(
)=2sin(A+
)=2∴A=
∵a2=bc
∴cosA=
=
=
∴b=c
∴△ABC为等边三角形.
(2)①由sin(A+B)=
,sin(A-B)=
展开可整理得:
∴sinAcosB=2cosAsinB
∴tanA=2tanB
②∵
<A+B<π,sin(A+B)=
,
∵tan(A+B)=-
,即
=-
,
又∵tanA=2tanB,∴2tan2B-4tan2B-1=0.
∴tanB=1+
或tanB=1-
(舍),
∴tanA=2tanB=2+
,
∴AB=AD+BD=
+
=3,
∴CD=2+
.
| m |
| n |
| 3 |
=2
| 3 |
| 3 |
| π |
| 6 |
T=
| 2π |
| 2 |
②∵f(
| A |
| 2 |
| π |
| 6 |
| π |
| 3 |
∵a2=bc
∴cosA=
| b2+c2-a2 |
| 2bc |
| b2+c2-bc |
| 2bc |
| 1 |
| 2 |
∴△ABC为等边三角形.
(2)①由sin(A+B)=
| 3 |
| 5 |
| 1 |
| 5 |
|
∴sinAcosB=2cosAsinB
∴tanA=2tanB
②∵
| π |
| 2 |
| 3 |
| 5 |
∵tan(A+B)=-
| 3 |
| 4 |
| tanA+tanB |
| 1-tanAtanB |
| 3 |
| 4 |
又∵tanA=2tanB,∴2tan2B-4tan2B-1=0.
∴tanB=1+
| ||
| 2 |
| ||
| 2 |
∴tanA=2tanB=2+
| 6 |
∴AB=AD+BD=
| CD |
| tanA |
| CD |
| tanB |
∴CD=2+
| 6 |
点评:本题主要考查了三角函数中的两角和公式的运用.此类题常综合三角函数性质、诱导公式、向量等问题.

练习册系列答案
相关题目
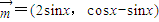 ,
,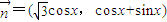 ,函数
,函数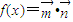 .
.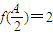 且a2=bc,试判断△ABC的形状.
且a2=bc,试判断△ABC的形状.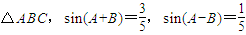 .
.

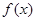 的最小正周期和值域;
的最小正周期和值域; 且
且 ,试判断△ABC的形状.
,试判断△ABC的形状. .
. ;
; ,求AB边上的高CD的长.
,求AB边上的高CD的长.

 的最小正周期和值域;
的最小正周期和值域; 且
且 ,试判断△ABC的形状.
,试判断△ABC的形状. .
. ;
; ,求AB边上的高CD的长.
,求AB边上的高CD的长.