题目内容
已知cos(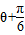 )=
)= ,且θ为锐角,sinθ的值.
,且θ为锐角,sinθ的值.
【答案】分析:根据θ为锐角,得到θ+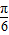 的范围,然后由cos(θ+
的范围,然后由cos(θ+ )的值,利用同角三角函数间的基本关系求出sin(θ+
)的值,利用同角三角函数间的基本关系求出sin(θ+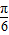 )的值,然后把所求的式子中的θ拆项为(
)的值,然后把所求的式子中的θ拆项为(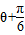 )-
)-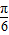 ,利用两角差的正弦函数公式及特殊角的三角函数值化简后,将sin(θ+
,利用两角差的正弦函数公式及特殊角的三角函数值化简后,将sin(θ+ )和cos(θ+
)和cos(θ+ )的值代入即可求出值.
)的值代入即可求出值.
解答:解:由θ∈(0, ),得到θ+
),得到θ+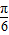 ∈(
∈(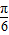 ,
,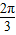 ),又cos(
),又cos(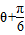 )=
)= ,
,
所以sin(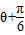 )=
)=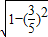 =
= ,
,
则sinθ=sin[(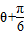 )-
)-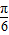 ]
]
=sin(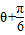 )cos
)cos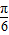 -cos(
-cos(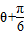 )sin
)sin
= ×
×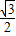 -
- ×
×
=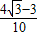 .
.
点评:此题考查学生灵活运用同角三角函数间的基本关系及两角差的正弦函数公式化简求值,是一道基础题.本题的关键是将所求式子中的角θ拆项为(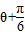 )-
)-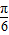 .
.
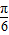 的范围,然后由cos(θ+
的范围,然后由cos(θ+ )的值,利用同角三角函数间的基本关系求出sin(θ+
)的值,利用同角三角函数间的基本关系求出sin(θ+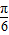 )的值,然后把所求的式子中的θ拆项为(
)的值,然后把所求的式子中的θ拆项为(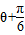 )-
)-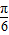 ,利用两角差的正弦函数公式及特殊角的三角函数值化简后,将sin(θ+
,利用两角差的正弦函数公式及特殊角的三角函数值化简后,将sin(θ+ )和cos(θ+
)和cos(θ+ )的值代入即可求出值.
)的值代入即可求出值.解答:解:由θ∈(0,
 ),得到θ+
),得到θ+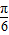 ∈(
∈(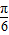 ,
,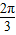 ),又cos(
),又cos(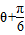 )=
)= ,
,所以sin(
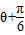 )=
)=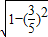 =
= ,
,则sinθ=sin[(
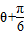 )-
)-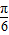 ]
]=sin(
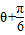 )cos
)cos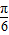 -cos(
-cos(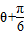 )sin
)sin
=
 ×
×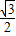 -
- ×
×
=
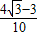 .
.点评:此题考查学生灵活运用同角三角函数间的基本关系及两角差的正弦函数公式化简求值,是一道基础题.本题的关键是将所求式子中的角θ拆项为(
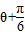 )-
)-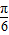 .
. 
练习册系列答案
相关题目
已知cos(
+α)=
,且-π<α<-
,则cos(
-α)等于( )
| 5π |
| 12 |
| 1 |
| 3 |
| π |
| 2 |
| π |
| 12 |
A、.
| ||||
B、
| ||||
C、-
| ||||
D、-
|