题目内容
已知函数 ,且给定条件p:“
,且给定条件p:“ ”,
”,
(1)求f(x)的最大值及最小值
(2)若又给条件q:“|f(x)-m|<2“且p是q的充分条件,求实数m的取值范围.
解:(1)∵f(x)=2[1-cos( +2x)]-2
+2x)]-2 cos2x-1=2sin2x-2
cos2x-1=2sin2x-2 cos2x+1
cos2x+1
=4sin(2x- )+1.
)+1.
又∵ ≤x≤
≤x≤ ,
,
∴ ≤2x-
≤2x-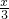 ≤
≤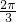 ,
,
即3≤4sin(2x- )+1≤5
)+1≤5
∴f(x)max=5,f(x)min=3
(2)∵|f(x)-m|<2,
∴m-2<f(x)<m+2
又p是q的充分条件
∴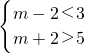 ,
,
∴3<m<5.
分析:(1)先根据两角和与差的公式进行化简,再由x的范围求出2x-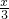 的范围,再结合正弦函数的性质可求出其最大、最小值.
的范围,再结合正弦函数的性质可求出其最大、最小值.
(2)先根据|f(x)-m|<2求出f(x)的范围,再由p是q的充分条件和(1)中f(x)的最大、最小值可得到m的范围.
点评:本题主要考查两角和与差的公式的应用和正弦函数的性质.高考中对三角函数的考查以基础题为主,平时要注意对基础知识的积累和运用的灵活性的锻炼.
 +2x)]-2
+2x)]-2 cos2x-1=2sin2x-2
cos2x-1=2sin2x-2 cos2x+1
cos2x+1=4sin(2x-
 )+1.
)+1.又∵
 ≤x≤
≤x≤ ,
,∴
 ≤2x-
≤2x-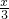 ≤
≤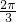 ,
,即3≤4sin(2x-
 )+1≤5
)+1≤5∴f(x)max=5,f(x)min=3
(2)∵|f(x)-m|<2,
∴m-2<f(x)<m+2
又p是q的充分条件
∴
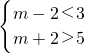 ,
,∴3<m<5.
分析:(1)先根据两角和与差的公式进行化简,再由x的范围求出2x-
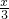 的范围,再结合正弦函数的性质可求出其最大、最小值.
的范围,再结合正弦函数的性质可求出其最大、最小值.(2)先根据|f(x)-m|<2求出f(x)的范围,再由p是q的充分条件和(1)中f(x)的最大、最小值可得到m的范围.
点评:本题主要考查两角和与差的公式的应用和正弦函数的性质.高考中对三角函数的考查以基础题为主,平时要注意对基础知识的积累和运用的灵活性的锻炼.

练习册系列答案
相关题目
 ,且给定条件p:
,且给定条件p: .
. ,且给定条件p:
,且给定条件p: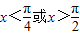 .
. ,且给定条件p:“
,且给定条件p:“ ”,
”,