题目内容
过点B(0,1)的直线l1交曲线x=2于P(2,y0),过点B'(0,-1)的直线l2交x轴于P'(x0,0)点,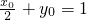 ,l1∩l2=M.
,l1∩l2=M.
(Ⅰ)求动点M的轨迹C的方程;
(Ⅱ)设直线l与C相交于不同的两点S、T,已知点S的坐标为(-2,0),点Q(0,m)在线段ST的垂直平分线上且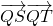 ≤4,求m的取值范围.
≤4,求m的取值范围.
解:(Ⅰ)由题意,直线l1的方程是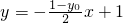 ,
,
∵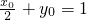 ,∴l1的方程是
,∴l1的方程是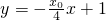
若直线l2与y轴重合,则M(0,1);
若直线l2不与y重合,可求得直线l2的方程是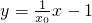 ,与l1的方程联立消去x0得
,与l1的方程联立消去x0得 ,
,
因l1不经过(0,-1),故动点M的轨迹C的方程是 (y≠-1)…(5分)
(y≠-1)…(5分)
(Ⅱ)设T(x1,y1),直线l的方程为y=k(x+2)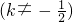
于是S、T两点的坐标满足方程组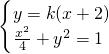 ,由方程消去y并整理得(1+4k2)x2+16k2x+16k2-4=0
,由方程消去y并整理得(1+4k2)x2+16k2x+16k2-4=0
由-2x1=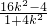 得x1=
得x1=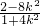 ,从而y1=
,从而y1=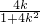
设线段ST的中点为N,则N(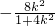 ,
,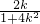 )…(7分)
)…(7分)
以下分两种情况:①当k=0时,点T的坐标为(2,0),线段ST的垂直平分线为y轴,
于是 ,由
,由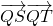 ≤4得:-2
≤4得:-2 ≤m≤2
≤m≤2 .
.
②当k≠0时,线段ST的垂直平分线方程为y-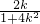 =-
=-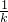 (x+
(x+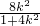 )
)
令x=0,得m=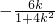
∵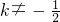 ,∴
,∴ ,
,
由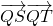 =-2x1-m(y1-m)=
=-2x1-m(y1-m)=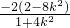 +
+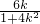 (
(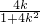 +
+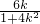 )=
)=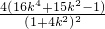 ≤4
≤4
解得-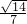 ≤k≤
≤k≤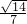 且k≠0,∴m=
且k≠0,∴m=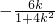 =
=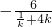
∴当-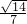 ≤k<0时,
≤k<0时,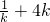 ≤-4;当0<k≤
≤-4;当0<k≤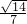 时,
时,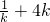 ≥4
≥4
∴-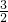 ≤m≤
≤m≤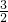 ,且m≠0
,且m≠0
综上所述,-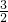 ≤m<
≤m<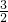 ,且m≠0.…(12分)
,且m≠0.…(12分)
分析:(Ⅰ)确定直线l1、l2的方程,联立方程可得动点M的轨迹C的方程;
(Ⅱ)设直线l的方程,代入椭圆方程,利用韦达定理,确定线段ST的中点坐标,分类讨论,利用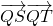 ≤4,即可得到结论.
≤4,即可得到结论.
点评:本题考查轨迹方程,考查直线与椭圆的位置关系,考查向量知识的运用,考查分类讨论的数学思想,考查学生分析解决问题的能力,属于中档题.
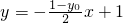 ,
,∵
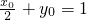 ,∴l1的方程是
,∴l1的方程是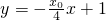
若直线l2与y轴重合,则M(0,1);
若直线l2不与y重合,可求得直线l2的方程是
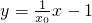 ,与l1的方程联立消去x0得
,与l1的方程联立消去x0得 ,
,因l1不经过(0,-1),故动点M的轨迹C的方程是
 (y≠-1)…(5分)
(y≠-1)…(5分)(Ⅱ)设T(x1,y1),直线l的方程为y=k(x+2)
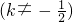
于是S、T两点的坐标满足方程组
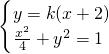 ,由方程消去y并整理得(1+4k2)x2+16k2x+16k2-4=0
,由方程消去y并整理得(1+4k2)x2+16k2x+16k2-4=0由-2x1=
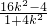 得x1=
得x1=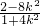 ,从而y1=
,从而y1=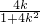
设线段ST的中点为N,则N(
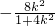 ,
,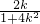 )…(7分)
)…(7分)以下分两种情况:①当k=0时,点T的坐标为(2,0),线段ST的垂直平分线为y轴,
于是
 ,由
,由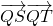 ≤4得:-2
≤4得:-2 ≤m≤2
≤m≤2 .
.②当k≠0时,线段ST的垂直平分线方程为y-
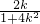 =-
=-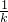 (x+
(x+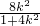 )
)令x=0,得m=
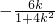
∵
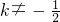 ,∴
,∴ ,
,由
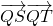 =-2x1-m(y1-m)=
=-2x1-m(y1-m)=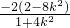 +
+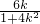 (
(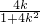 +
+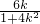 )=
)=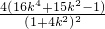 ≤4
≤4解得-
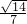 ≤k≤
≤k≤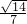 且k≠0,∴m=
且k≠0,∴m=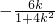 =
=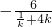
∴当-
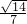 ≤k<0时,
≤k<0时,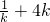 ≤-4;当0<k≤
≤-4;当0<k≤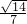 时,
时,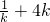 ≥4
≥4∴-
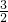 ≤m≤
≤m≤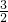 ,且m≠0
,且m≠0综上所述,-
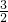 ≤m<
≤m<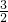 ,且m≠0.…(12分)
,且m≠0.…(12分)分析:(Ⅰ)确定直线l1、l2的方程,联立方程可得动点M的轨迹C的方程;
(Ⅱ)设直线l的方程,代入椭圆方程,利用韦达定理,确定线段ST的中点坐标,分类讨论,利用
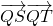 ≤4,即可得到结论.
≤4,即可得到结论.点评:本题考查轨迹方程,考查直线与椭圆的位置关系,考查向量知识的运用,考查分类讨论的数学思想,考查学生分析解决问题的能力,属于中档题.

练习册系列答案
相关题目
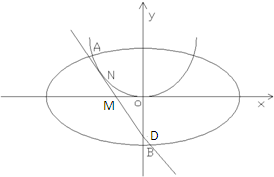 椭圆E:
椭圆E: 过点A(a,0),B(0,b)的直
过点A(a,0),B(0,b)的直 ,原点到该直线的距离为
,原点到该直线的距离为 .
. 求直线MN的方程;
求直线MN的方程; 交椭圆于P、Q两点,以PQ为直径的圆过点D(1,0)?若存在,求出k的值;若不存在,请说明理由。
交椭圆于P、Q两点,以PQ为直径的圆过点D(1,0)?若存在,求出k的值;若不存在,请说明理由。