题目内容
已知函数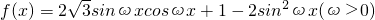 ,且函数f(x)的最小正周期为π.
,且函数f(x)的最小正周期为π.
(1)若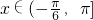 ,求函数f(x)的单调递减区间;
,求函数f(x)的单调递减区间;
(2)将函数y=f(x)的图象上各点的纵坐标保持不变,横坐标缩短到原来的 ,把所得到的图象再向左平移
,把所得到的图象再向左平移 个单位,得到函数y=g(x)的图象,求函数y=g(x)在区间
个单位,得到函数y=g(x)的图象,求函数y=g(x)在区间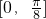 上的最小值.
上的最小值.
解:(1)∵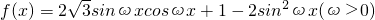
∴利用三角函数的降次公式,得f(x)= sin(2ωx)+cos(2ωx)=2sin(2ωx+
sin(2ωx)+cos(2ωx)=2sin(2ωx+ )
)
∵函数f(x)的最小正周期为T=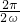 =π
=π
∴2ω=2,可得函数f(x)的解析式为:y=2sin(2x+ )
)
令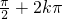 <2x+
<2x+ <
<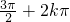 ,得
,得 +kπ<x<
+kπ<x<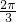 +kπ,其中k是整数,
+kπ,其中k是整数,
∵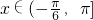 ,
,
∴取k=0,得x∈
所以函数f(x)的单调递减区间是 ;
;
(2)函数y=f(x)的图象上各点的纵坐标保持不变,横坐标缩短到原来的 ,
,
所得函数解析式为:y=2sin(4x+ )
)
再把所得到的图象再向左平移 个单位,得到函数y=g(x)的图象,
个单位,得到函数y=g(x)的图象,
∴g(x)=2sin[4(x+ )+
)+ ]=2sin(4x+
]=2sin(4x+ )
)
∵函数y=g(x)定义在区间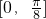 上,
上,
∴4x+ ∈[
∈[ ,
,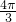 ]?sin
]?sin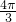 ≤sin(4x+
≤sin(4x+ )≤sin
)≤sin
即- ≤sin(4x+
≤sin(4x+ )≤
)≤
∴函数y=g(x)的值域为[- ,1],函数的最小值为-
,1],函数的最小值为- .
.
分析:(1)利用三角函数的降次公式进行化简,得f(x)=2sin(2ωx+ ),根据函数y=Asin(ωx+φ)的周期的公式,计算出ω的值,得到函数的表达式,最后根据函数函数y=Asin(ωx+φ)的单调区间的结论,可以求得函数f(x)的单调递减区间;
),根据函数y=Asin(ωx+φ)的周期的公式,计算出ω的值,得到函数的表达式,最后根据函数函数y=Asin(ωx+φ)的单调区间的结论,可以求得函数f(x)的单调递减区间;
(2)根据函数y=Asin(ωx+φ)的图象变换的规律,得到变换后函数y=g(x)的解析式是:g(x)=2sin(4x+ ),然后根据函数y=Asin(ωx+φ)的单调性的结论,可得函数g(x)在区间
),然后根据函数y=Asin(ωx+φ)的单调性的结论,可得函数g(x)在区间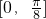 上的值域,从而得到y=g(x)在区间
上的值域,从而得到y=g(x)在区间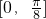 上的最小值.
上的最小值.
点评:本题以一个特殊的三角函数为例加以研究,着重考查了三角函数中的恒等变换、函数y=Asin(ωx+φ)的图象和性质和三角函数的最值等知识点,属于中档题.
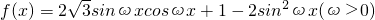
∴利用三角函数的降次公式,得f(x)=
 sin(2ωx)+cos(2ωx)=2sin(2ωx+
sin(2ωx)+cos(2ωx)=2sin(2ωx+ )
)∵函数f(x)的最小正周期为T=
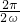 =π
=π∴2ω=2,可得函数f(x)的解析式为:y=2sin(2x+
 )
)令
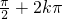 <2x+
<2x+ <
<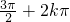 ,得
,得 +kπ<x<
+kπ<x<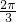 +kπ,其中k是整数,
+kπ,其中k是整数,∵
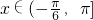 ,
,∴取k=0,得x∈

所以函数f(x)的单调递减区间是
 ;
;(2)函数y=f(x)的图象上各点的纵坐标保持不变,横坐标缩短到原来的
 ,
,所得函数解析式为:y=2sin(4x+
 )
)再把所得到的图象再向左平移
 个单位,得到函数y=g(x)的图象,
个单位,得到函数y=g(x)的图象,∴g(x)=2sin[4(x+
 )+
)+ ]=2sin(4x+
]=2sin(4x+ )
)∵函数y=g(x)定义在区间
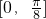 上,
上,∴4x+
 ∈[
∈[ ,
,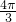 ]?sin
]?sin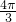 ≤sin(4x+
≤sin(4x+ )≤sin
)≤sin
即-
 ≤sin(4x+
≤sin(4x+ )≤
)≤
∴函数y=g(x)的值域为[-
 ,1],函数的最小值为-
,1],函数的最小值为- .
.分析:(1)利用三角函数的降次公式进行化简,得f(x)=2sin(2ωx+
 ),根据函数y=Asin(ωx+φ)的周期的公式,计算出ω的值,得到函数的表达式,最后根据函数函数y=Asin(ωx+φ)的单调区间的结论,可以求得函数f(x)的单调递减区间;
),根据函数y=Asin(ωx+φ)的周期的公式,计算出ω的值,得到函数的表达式,最后根据函数函数y=Asin(ωx+φ)的单调区间的结论,可以求得函数f(x)的单调递减区间;(2)根据函数y=Asin(ωx+φ)的图象变换的规律,得到变换后函数y=g(x)的解析式是:g(x)=2sin(4x+
 ),然后根据函数y=Asin(ωx+φ)的单调性的结论,可得函数g(x)在区间
),然后根据函数y=Asin(ωx+φ)的单调性的结论,可得函数g(x)在区间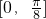 上的值域,从而得到y=g(x)在区间
上的值域,从而得到y=g(x)在区间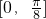 上的最小值.
上的最小值.点评:本题以一个特殊的三角函数为例加以研究,着重考查了三角函数中的恒等变换、函数y=Asin(ωx+φ)的图象和性质和三角函数的最值等知识点,属于中档题.

练习册系列答案
 名校课堂系列答案
名校课堂系列答案
相关题目