题目内容
已知函数 在(0,1)上为减函数.
在(0,1)上为减函数.
(1)讨论f(x)的单调性(指出单调区间);
(2)当a>0时,如果f(x)在(0,1)上为减函数,g(x)=x2-2alnx在(1,2)上是增函数,求实数a的值;
(3)当a=2时,若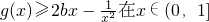 内恒成立,求b的取值范围.
内恒成立,求b的取值范围.
解:(1)∵函数 ,∴f′(x)=1-
,∴f′(x)=1-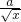 ,
,
∵函数 在(0,1)上为减函数.
在(0,1)上为减函数.
∴f′(x)=1-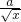 ≤0在(0,1)上恒成立,
≤0在(0,1)上恒成立,
∴a≥1.
f′(x)=1-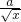 >0得:x>a2,
>0得:x>a2,
故f(x)的单调增区间为:(a2,+∞),减区间为(0,a2)
(2)由(1)得a≥1,
又g(x)=x2-2alnx在(1,2)上是增函数,
∴g′(x)=2x-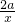 ≥0在(1,2)上恒成立,
≥0在(1,2)上恒成立,
?a≤x2,?a≤1,
∴a=1.
(3)当a=2时,若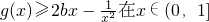 内恒成立,
内恒成立,
即:x2-4lnx≥2bx-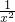 ,
,
2b≤x+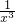 -
-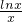 ,设h(x)=x+
,设h(x)=x+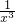 -
-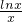 ,它在(0,1)上是减函数,
,它在(0,1)上是减函数,
∴2b≤h(1)?2b≤2,?b≤1.
∴b的取值范围b≤1.
分析:(1)先求导数得:f′(x)=1-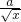 ,根据函数
,根据函数 在(0,1)上为减函数.得出f′(x)=1-
在(0,1)上为减函数.得出f′(x)=1-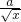 ≤0在(0,1)上恒成立,得到a的取值范围,再利用导数研究函数的单调性得出f(x)的单调区间;
≤0在(0,1)上恒成立,得到a的取值范围,再利用导数研究函数的单调性得出f(x)的单调区间;
(2)由(1)得a≥1,又g(x)=x2-2alnx在(1,2)上是增函数,利用导数研究函数的单调性得出a≤1,从而得出a的值;
(3)当a=2时,若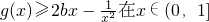 内恒成立,再分离出2b:2b≤x+
内恒成立,再分离出2b:2b≤x+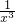 -
-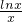 ,设h(x)=x+
,设h(x)=x+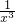 -
-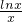 ,它在(0,1)上是减函数,只须2b小于h(1)即可求出b的取值范围.
,它在(0,1)上是减函数,只须2b小于h(1)即可求出b的取值范围.
点评:本小题主要考查函数恒成立问题\函数单调性的应用、利用导数研究函数的单调性、不等式的解法等基础知识,考查运算求解能力,考查化归与转化思想.属于基础题.
 ,∴f′(x)=1-
,∴f′(x)=1-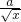 ,
,∵函数
 在(0,1)上为减函数.
在(0,1)上为减函数.∴f′(x)=1-
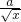 ≤0在(0,1)上恒成立,
≤0在(0,1)上恒成立,∴a≥1.
f′(x)=1-
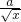 >0得:x>a2,
>0得:x>a2,故f(x)的单调增区间为:(a2,+∞),减区间为(0,a2)
(2)由(1)得a≥1,
又g(x)=x2-2alnx在(1,2)上是增函数,
∴g′(x)=2x-
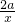 ≥0在(1,2)上恒成立,
≥0在(1,2)上恒成立,?a≤x2,?a≤1,
∴a=1.
(3)当a=2时,若
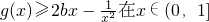 内恒成立,
内恒成立,即:x2-4lnx≥2bx-
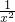 ,
,2b≤x+
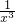 -
-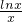 ,设h(x)=x+
,设h(x)=x+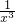 -
-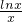 ,它在(0,1)上是减函数,
,它在(0,1)上是减函数,∴2b≤h(1)?2b≤2,?b≤1.
∴b的取值范围b≤1.
分析:(1)先求导数得:f′(x)=1-
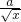 ,根据函数
,根据函数 在(0,1)上为减函数.得出f′(x)=1-
在(0,1)上为减函数.得出f′(x)=1-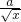 ≤0在(0,1)上恒成立,得到a的取值范围,再利用导数研究函数的单调性得出f(x)的单调区间;
≤0在(0,1)上恒成立,得到a的取值范围,再利用导数研究函数的单调性得出f(x)的单调区间;(2)由(1)得a≥1,又g(x)=x2-2alnx在(1,2)上是增函数,利用导数研究函数的单调性得出a≤1,从而得出a的值;
(3)当a=2时,若
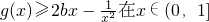 内恒成立,再分离出2b:2b≤x+
内恒成立,再分离出2b:2b≤x+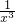 -
-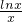 ,设h(x)=x+
,设h(x)=x+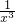 -
-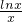 ,它在(0,1)上是减函数,只须2b小于h(1)即可求出b的取值范围.
,它在(0,1)上是减函数,只须2b小于h(1)即可求出b的取值范围.点评:本小题主要考查函数恒成立问题\函数单调性的应用、利用导数研究函数的单调性、不等式的解法等基础知识,考查运算求解能力,考查化归与转化思想.属于基础题.

练习册系列答案
相关题目
 在区间[0,1]上的最大值是2,求实数a的值.
在区间[0,1]上的最大值是2,求实数a的值.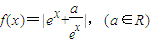 在区间[0,1]上单调递增,则实数a的取值范围是( )
在区间[0,1]上单调递增,则实数a的取值范围是( )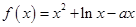 在(0,1)上是增函数.(1)求
在(0,1)上是增函数.(1)求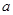 的取值范围;
的取值范围; (
( ),试求函数
),试求函数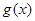 的最小值.
的最小值.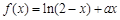 在(0,1)内是增函数.
在(0,1)内是增函数.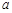 的取值范围;
的取值范围;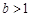 ,求证:
,求证: .
. 在区间[0,1]上的最小值为0,则a的值为
。
在区间[0,1]上的最小值为0,则a的值为
。