题目内容
关于平面向量有下列四个命题:①若
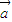 •
•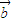 =
=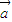 •
•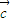 ,则
,则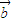 =
=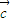 ,;
,;②已知
 =(k,3),
=(k,3),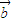 =(-2,6).若
=(-2,6).若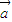 ∥
∥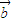 ,则k=-1.
,则k=-1.③非零向量
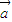 和
和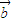 ,满足|
,满足|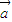 |=|
|=|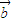 |=|
|=|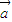 -
- |,则
|,则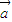 与
与 +
+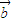 的夹角为30°.
的夹角为30°.④(
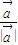 +
+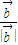 )•(
)•( -
-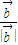 )=0.
)=0.其中正确的命题为 .(写出所有正确命题的序号)
【答案】分析:通过举反例知①不成立,由平行向量的坐标对应成比列知②正确,由向量加减法的意义知,③正确,通过化简计算得④正确.
解答:解:当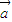 =
=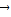 时,可得到①不成立.
时,可得到①不成立.
对于② ∥
∥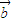 时,有
时,有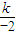 =
= ,∴k=-1,故②正确.
,∴k=-1,故②正确.
当|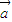 |=|
|=|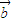 |=|
|=| -
- |时,
|时,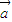 、
、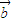 、
、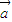 -
-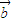 这三个向量平移后构成一个等边三角形,
这三个向量平移后构成一个等边三角形,
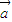 +
+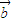 是这个等边三角形一条角平分线,故③正确.
是这个等边三角形一条角平分线,故③正确.
∵(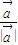 +
+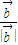 )•(
)•(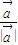 -
-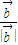 )=
)=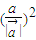 -
-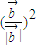 =1-1=0,故④正确.
=1-1=0,故④正确.
综上,②③④正确,①不正确,
故答案为 ②③④.
点评:本题考查两个向量的数量积公式,两个向量加减法的几何意义,以及共线向量的坐标特点.
解答:解:当
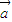 =
=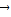 时,可得到①不成立.
时,可得到①不成立. 对于②
 ∥
∥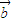 时,有
时,有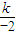 =
= ,∴k=-1,故②正确.
,∴k=-1,故②正确.当|
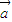 |=|
|=|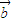 |=|
|=| -
- |时,
|时,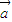 、
、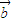 、
、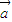 -
-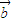 这三个向量平移后构成一个等边三角形,
这三个向量平移后构成一个等边三角形,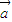 +
+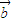 是这个等边三角形一条角平分线,故③正确.
是这个等边三角形一条角平分线,故③正确.∵(
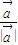 +
+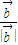 )•(
)•(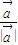 -
-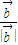 )=
)=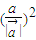 -
-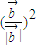 =1-1=0,故④正确.
=1-1=0,故④正确.综上,②③④正确,①不正确,
故答案为 ②③④.
点评:本题考查两个向量的数量积公式,两个向量加减法的几何意义,以及共线向量的坐标特点.

练习册系列答案
 精英口算卡系列答案
精英口算卡系列答案 应用题点拨系列答案
应用题点拨系列答案 状元及第系列答案
状元及第系列答案 同步奥数系列答案
同步奥数系列答案
相关题目
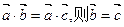 ;②已知
;②已知 .若
.若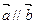 ,则
,则 ;③非零向量
;③非零向量 ,满足
,满足 ,则
,则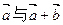 的夹角为
的夹角为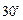 ;④
;④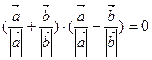 .其中正确的命题为___________.(写出所有正确命题的序号)
.其中正确的命题为___________.(写出所有正确命题的序号)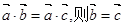 ;②已知
;②已知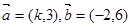 .若
.若 ,则
,则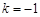 ;③非零向量
;③非零向量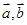 ,满足
,满足 ,则
,则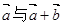 的夹角为
的夹角为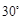 ;④
;④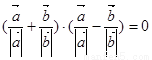 .其中正确的命题为___________.(写出所有正确命题的序号)
.其中正确的命题为___________.(写出所有正确命题的序号)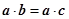 ,则
,则 ; ②已知
; ②已知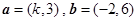 .若
.若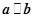 ,则
,则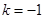 ;
;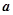 和
和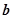 ,满足
,满足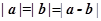 ,则
,则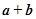 的夹角为
的夹角为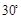 ;
;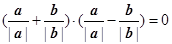 .
.