网址:http://m.1010jiajiao.com/timu_id_48531[举报]
一. 选择题(每小题5分)
题号
1
2
3
4
5
6
7
8
9
10
答案
A
B
D
C
D
B
C
B
C
A
二. 填空题(每小题5分)
11. 12。
12。 13。-1
14。
13。-1
14。 15。
15。
三. 解答题
 ……………2分
……………2分
且2R= ,由正弦定理得:
,由正弦定理得:
化简得:  ……………4分
……………4分
由余弦定理:


 ……………11分
……………11分
所以, ……………12分
……………12分
17.解:(I)记事件A=“该单位所派的选手都是男职工” ……………1分
则P(A)= ……………3分
……………3分
(II)记事件B=“该单位男职工、女职工选手参加比赛” ……………4分
则P(B)= ……………7分
……………7分
(III)设该单位至少有一名选手获奖的概率为P,则

或 ……………12分
……………12分
 18.(解法一)(I)取AB的中点为Q,连接PQ,则
18.(解法一)(I)取AB的中点为Q,连接PQ,则 ,所以,
,所以, 为AC与BD所成角……………2分
为AC与BD所成角……………2分


又CD=BD=1, ,而PQ=1,DQ=1
,而PQ=1,DQ=1
 ……………4分
……………4分
(II)过D作 ,连接CR,
,连接CR, ,
,

 ……………6分
……………6分
在 ,
,
 ……………8分
……………8分
 ……………9分
……………9分
(解法二)(I)如图,以D为坐标原点,DB、AD、DC所在直线分别为x,y,z轴建立直角坐标系。则A( ),C(0,0,1),B(1,0,0),P(
),C(0,0,1),B(1,0,0),P( ),D(0,0,0)
),D(0,0,0)

 ,
, ……2分
……2分

所以,异面直线AC与BD所成角的余弦值为 ……………4分
……………4分
(II)面DAB的一个法向量为 ………5分
………5分
设面ABC的一个法向量 ,则
,则
 ,取
,取 ,……………7分
,……………7分
则
 ……………8分
……………8分
 …………9分
…………9分
(III)不存在。若存在S使得AC ,则
,则 ,与(I)矛盾。故不存在…12分
,与(I)矛盾。故不存在…12分
19.解:(I) 在区间
在区间 上递减,其导函数
上递减,其导函数 ……………1分
……………1分
 ……………4分
……………4分
故 是函数
是函数 在区间
在区间 上递减的必要而不充分的条件……………5分
上递减的必要而不充分的条件……………5分
(II)
 ……………6分
……………6分
当a>0时,函数 在(
在( )上递增,在
)上递增,在 上递减,在
上递减,在 上递增,故有
上递增,故有
 ……………9分
……………9分
当a〈0时,函数 在
在 上递增,
上递增, 只要
只要
令 ,则
,则 …………11分
…………11分
所以 在
在 上递增,又
上递增,又
 不能恒成立。
不能恒成立。
故所求的a的取值范围为 ……………12分
……………12分
20.解:(I)由条件,M到F(1,0)的距离等于到直线 x= -1的距离,所以,曲线C是以F为焦点、直线 x= -1为准线的抛物线,其方程为 ……………3分
……………3分
(II)设 ,代入
,代入 得:
得: ……………5分
……………5分
由韦达定理
 ,
,
 ……………6分
……………6分
 ,只要将A点坐标中的
,只要将A点坐标中的 换成
换成 ,得
,得 ……7分
……7分
 ……………8分
……………8分
所以, 最小时,弦PQ、RS所在直线的方程为
最小时,弦PQ、RS所在直线的方程为 ,
,
即 或
或 ……………9分
……………9分
(III) ,即A、T、B三点共线。
,即A、T、B三点共线。
 是否存在一定点T,使得
是否存在一定点T,使得 ,即探求直线AB是否过定点。
,即探求直线AB是否过定点。
由(II)知,直线AB的方程为 ………10分
………10分
即 ,
, 直线AB过定点(3,0).……………12分
直线AB过定点(3,0).……………12分
故存在一定点T(3,0),使得 ……………13分
……………13分
21.解:(I)因为曲线在 处的切线与
处的切线与 平行
平行
 ……………4分
……………4分
 ,
,

(III)。由(II)知: =
=
 ,从而
,从而 ……………11分
……………11分


 ,
,

设f(x)是定义在[0,1]上的函数,若存在x*∈(0,1),使得f(x)在[0,x*]上单调递增,在[x*,1]上单调递减,则称f(x)为[0,1]上的单峰函数,x*为峰点,包含峰点的区间为含峰区间.对任意的[0,1]上的单峰函数f(x),下面研究缩短其含峰区间长度的方法.
(I)证明:对任意的 ∈(O,1),
∈(O,1), ,若f(
,若f( )≥f(
)≥f( ),则(0,
),则(0, )为含峰区间:若f(
)为含峰区间:若f( )
) f(
f( ),则
),则 为含峰区间:
为含峰区间:
(II)对给定的r(0<r<0.5),证明:存在 ∈(0,1),满足
∈(0,1),满足 ,使得由(I)所确定的含峰区间的长度不大于0.5+r:
,使得由(I)所确定的含峰区间的长度不大于0.5+r:
(III)选取 ∈(O,1),,由(I)可确定含峰区间为
∈(O,1),,由(I)可确定含峰区间为 或
或 ,在所得的含峰区间内选取
,在所得的含峰区间内选取 ,由
,由 与
与 或
或 与
与 类似地可确定一个新的含峰区间,在第一次确定的含峰区间为(0,
类似地可确定一个新的含峰区间,在第一次确定的含峰区间为(0, )的情况下,试确定的值
)的情况下,试确定的值 ,满足两两之差的绝对值不小于0.02,且使得新的含峰区间的长度缩短到0. 34(区间长度等于区间的右端点与左端点之差)
,满足两两之差的绝对值不小于0.02,且使得新的含峰区间的长度缩短到0. 34(区间长度等于区间的右端点与左端点之差)
查看习题详情和答案>>
(I)证明:对任意的
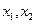 ∈(O,1),
∈(O,1),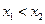 ,若f(
,若f( )≥f(
)≥f(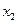 ),则(0,
),则(0,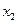 )为含峰区间:若f(
)为含峰区间:若f( )
)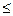 f(
f(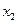 ),则
),则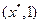 为含峰区间:
为含峰区间:(II)对给定的r(0<r<0.5),证明:存在
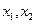 ∈(0,1),满足
∈(0,1),满足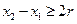 ,使得由(I)所确定的含峰区间的长度不大于0.5+r:
,使得由(I)所确定的含峰区间的长度不大于0.5+r:(III)选取
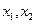 ∈(O,1),,由(I)可确定含峰区间为
∈(O,1),,由(I)可确定含峰区间为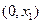 或
或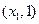 ,在所得的含峰区间内选取
,在所得的含峰区间内选取 ,由
,由 与
与 或
或 与
与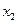 类似地可确定一个新的含峰区间,在第一次确定的含峰区间为(0,
类似地可确定一个新的含峰区间,在第一次确定的含峰区间为(0,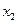 )的情况下,试确定的值
)的情况下,试确定的值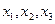 ,满足两两之差的绝对值不小于0.02,且使得新的含峰区间的长度缩短到0. 34(区间长度等于区间的右端点与左端点之差)
,满足两两之差的绝对值不小于0.02,且使得新的含峰区间的长度缩短到0. 34(区间长度等于区间的右端点与左端点之差)(I)证明:a<3是函数f(x)在区间(1,2)上递减的必要而不充分的条件;
(II)若x∈[0,|a|+1]时,f(x)<2a2恒成立,且f(0)=0,求实数a的取值范围. 查看习题详情和答案>>